January 11, 2019 - Mono Mole

How do we solve the differential equation $E^2(E^2\psi)=0$?

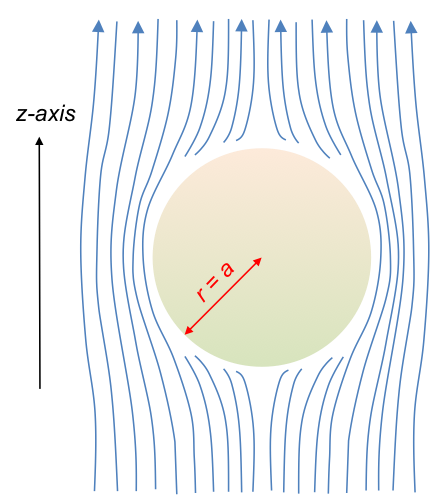
We have, in an earlier article, defined the Stokes stream function for an incompressible fluid at the boundaries of r = a and r = ∞ where:

$\psi=0\; \; \; \; \; if\; r=a$

$\psi=\frac{\textbf{\textit{u}}}{2}r^2sin^2\theta\; \; \; \; \; if\; r=\infty$

To develop a Stokes stream function that satisfies eq36 for a ≤ r ≤ ∞ , let’s consider a solution of the form:

$\psi=fsin^2\theta\; \; \; \; \; \; \; (37)$

where $f$ is a function of r.

Substitute eq37 in eq36 and noting that $\frac{\partial }{\partial \theta}\left ( \frac{1}{sin\theta}\frac{\partial }{\partial \theta} \right )sin^2\theta=-2sin\theta$ , we have:

$\left ( \frac{\partial^2 }{\partial r^2} -\frac{2}{r^2}\right )^2f=0\; \; \; \; \; \; \; (38)$

Letting $f=r^n$ and solving eq38, we get n = ±1, 2, 4. Therefore, the function $f$ takes the form:

$f=\frac{A}{r}+Br+Cr^2+Dr^4\; \; \; \; \; \; \; (39)$

Substitute eq39 in eq37,

$\psi=\left ( \frac{A}{r}+Br+Cr^2+Dr^4 \right )sin^2\theta\; \; \; \; \; \; \; (40)$

To satisfy the function at the top of the page at r → ∞, we let D = 0 and C = u/2. Eq40 becomes:

$\psi=\left ( \frac{A}{r}+Br+\frac{\textbf{\textit{u}}}{2}r^2 \right )sin^2\theta\; \; \; \; \; \; \; (41)$

Substitute eq41 in eq15 and eq16, we have:

$u_r=2cos\theta\left ( \frac{A}{r^3}+\frac{B}{r}+\frac{\textbf{\textit{u}}}{2} \right )\; \; \; \; \; \; \; (42)$

$u_\theta=sin\theta\left (- \frac{A}{r^3}+\frac{B}{r}+\textbf{\textit{u}} \right )\; \; \; \; \; \; \; (43)$

To satisfy the function at the top of the page at r = a, u_r = 0 and u_θ = 0, i.e. velocity of the fluid on the surface of the object is zero relative to the velocity of the object. This is because the force of attraction between the fluid particles and solid particles of the object is greater than that between the fluid particles. Eq42 and eq43 become:

$\frac{A}{a^3}+\frac{B}{a}+\frac{\textbf{\textit{u}}}{2}=0\; \; \; \; and\; \; \; \;-\frac{A}{a^3}+\frac{B}{a}+\textbf{\textit{u}}=0$

Solving the simultaneous equations gives:

$A=\frac{\textbf{\textit{u}}}{4}a^3\; \; \; \; and\; \; \; \; B=-\frac{3\textbf{\textit{u}}}{4}a$

Substitute A and B in eq41,

$\psi=sin^2\theta\frac{\textbf{\textit{u}}}{4}\left ( \frac{a^3}{r}-3ar+2r^2 \right )\; \; \; \; \; \; \; (44)$

Therefore, we have a Stokes stream function that satisfies the differential equation eq36 for a ≤ r ≤ ∞ .

Substitute eq44 in eq15 and eq16, we have:

$u_r=\textbf{\textit{u}}cos\theta\left ( \frac{a^3}{2r^3}-\frac{3a}{2r}+1 \right )\; \; \; \; \; \; \; (45)$

$u_\theta=-\textbf{\textit{u}}sin\theta\left ( -\frac{a^3}{4r^3}-\frac{3a}{4r}+1 \right )\; \; \; \; \; \; \; (46)$

Eq45 and eq46 are the component flow velocities of the viscous incompressible fluid.

NExt article: derivation of stokes’ law

Previous article: derivation of the differential equation, **E²(E²ψ) = 0**

Content page of Stokes’ law

Content page of advanced chemistry

Main content page

The Stokes stream function is a mathematical representation of the trajectories of particles in a steady flow of fluid over an object. In other words, a plot of the Stokes stream function results in streamlines seen in the diagram below.

To derive the Stokes stream function, we make the following assumptions:

The flow of the fluid is axisymmetric, i.e. defined along the polar axis (z-axis), and therefore the velocity components are independent of Φ.
The fluid is incompressible and therefore has a constant density.

Eq12 of the previous article becomes:

$\frac{1}{r^2}\frac{\partial r^2u_r}{\partial r}+\frac{1}{rsin\theta}\frac{\partial u_\theta sin\theta}{\partial \theta}=0\; \; \; \; \; \; \; (13)$

Question

Show that $\nabla\cdot u=0$ .

Answer

The divergence of a function in spherical coordinate is:

$\nabla\cdot \boldsymbol{\mathit{f}}=\frac{1}{r^{2}}\frac{\partial r^{2}f_r}{\partial r}+\frac{1}{rsin\theta}\frac{\partial f_{\theta}sin\theta}{\partial\theta}+\frac{1}{rsin\theta}\frac{\partial f_{\phi}}{\partial \phi}$

If the flow is axisymmetric, the divergence of an axisymmetric flow function in spherical coordinates is exactly the LHS of eq13 and we can write eq13 as:

$\nabla \cdot\textbf{\textit{u}}=0\; \; \; \; \; \; \; (14)$

Eq14 is needed later for the derivation of the differential equation E²(E²ψ=0).

George Stokes developed the solution to eq13 by defining the Stokes stream function ψ where:

$u_r=\frac{1}{r^2sin\theta}\frac{\partial \psi}{\partial \theta}\; \; \; \; \; \; \; (15)$

and

$u_\theta=-\frac{1}{rsin\theta}\frac{\partial \psi}{\partial r}\; \; \; \; \; \; \; (16)$

Question

Show that eq15 and eq16 satisfy eq13.

Answer

Substitute eq15 and eq16 in eq13:

$\frac{1}{r^2sin\theta}\frac{\partial }{\partial r}\left ( \frac{\partial \psi}{\partial \theta} \right )-\frac{1}{r^2sin\theta}\frac{\partial }{\partial \theta}\left ( \frac{\partial \psi}{\partial r} \right )=0$

Since $\frac{\partial }{\partial r}\left ( \frac{\partial \psi}{\partial \theta} \right )= \frac{\partial }{\partial \theta}\left ( \frac{\partial \psi}{\partial r} \right )$ , eq15 and eq16 satisfy eq13.

With reference to the diagram at the top of the page, the flow velocity u, which is defined in the z-direction, varies at different distances from the surface of the sphere. On the surface of the sphere,

$r=a\; \; \; \; and\; \; \; \; \psi=0\; \; \; \; \; \; \; (17)$

At r = ∞, we assume that the flow velocity of the fluid is uniform. The flow velocity at any point in fluid at can be deconstructed into its radial and polar components (see above diagram) where:

$u_r=\textbf{\textit{u}}cos\theta\; \; \; \; \; \; \; (18)$

and

$u_\theta=-\textbf{\textit{u}}sin\theta\; \; \; \; \; \; \; (19)$

Substitute eq15 in eq18 and eq16 in eq19, we have,

$\frac{1}{r^2sin\theta}\frac{\partial \psi}{\partial \theta}= \textbf{\textit{u}}cos\theta\; \; \; \; \; \; \; (20)$

$\frac{1}{rsin\theta}\frac{\partial \psi}{\partial r}= \textbf{\textit{u}}sin\theta\; \; \; \; \; \; \; (21)$

Integrating eq21, we get:

$\textbf{\textit{u}}sin^2\theta\int_{a}^{r}r\; dr=\int_{0}^{\psi}d\psi$

$\psi=\frac{\textbf{\textit{u}}}{2}\left ( r^2-a^2 \right )sin^2\theta$

At r = ∞, r²» a², so

$\psi\approx\frac{\textbf{\textit{u}}}{2}r^2sin^2\theta\; \; \; \; \; \; \; (22)$

Eq17 and eq22 express the Stokes stream function for an incompressible fluid at the boundaries of r = a and r = ∞ respectively. In the next few articles, we shall define the Stokes stream function at a ≤ r ≤ ∞.

Day: January 11, 2019