Category: Basic Chemistry

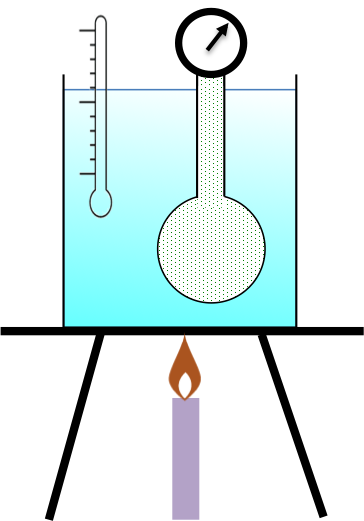
Joseph Gay-Lussac, a French scientist, is often credited with the discovery of the pressure-temperature law in the early 1800s even though Guillaume Amontons, another French scientist had conducted a similar experiment earlier on with the same conclusion. Gay-Lussac’s experimental apparatus involves immersing a gas-filled, fixed-volume bulb-like copper container in a temperature controlled water bath. The container is connected to a pressure gauge, which measures the pressure of the gas in the container at various temperatures (see diagram below).

Both Gay-Lussac and Amontons found that the relationship between the pressure of a gas and its temperature in Celsius is linear at constant volume.

where m is the gradient of the function and c is the intercept the function makes with the vertical axis.

Furthermore, the function extrapolates to -273.15 ^oC at zero pressure regardless of the gas investigated (see graph above). Similar to the way a Celsius-based expression is derived from Jacques Charles’ experimental data, the Celsius-based formula for Gay-Lussac’s experiments is:

where p₀ is the pressure of the gas at zero degrees Celsius and .

By changing the units of the horizontal axis from Celsius to Kevin (see diagram above), the relationship between pressure and temperature becomes directly proportional:

where k₃ is the proportionality constant.

Eq11 is known as the Gay-Lussac’s law (or Amontons’ law), which states:

The pressure of a given mass of gas is directly proportional to its absolute temperature (K) at constant volume

Note that eq10 is also called the Gay-Lussac’s law (or Amontons’ law) with T in Celsius. Since p₁ = k₃T₁  and p₂ = k₃T₂, Gay-Lussac’s law can also be expressed as

Gay-Lussac also experimented on other properties of gases and discovered the law of combining volumes, which states:

Volumes of gases that react with one another are in the ratios of small whole numbers at a given pressure and temperature.

For example, two volumes of hydrogen gas react with one volume of oxygen gas to form two volumes of water:

 

next article: Avogadro’s law

Previous article: Charles’s law

Content page of gas laws

Content page of Basic chemistry

Main content page

Amedeo Avogadro, an Italian scientist, analysed Gay-Lussac’s law of combining volumes and hypothesised in 1811 that

Equal volumes of all gases at the same temperature and pressure have the same number of molecules

This became known as Avogadro’s law or Avogadro’s principle. It implies that for a given temperature and pressure, the volume V of a gas is directly proportional to its amount (in moles), n:

where k₄ is the proportionality constant.

Since V₁ = k₄n₁ and V₂ = k₄n₂, Avogadro’s law can also be expressed as

 

next article: Ideal gas law

Previous article: Gay-Lussac’s law

Content page of gas laws

Content page of Basic chemistry

Main content page

The ideal gas law is a mathematical expression that describes the behaviour of an ideal gas.

So far, all the gas laws mentioned in the previous sections work reasonably well when the experiments are done at low pressures (≤ 1 atm), where the following assumptions about gas particles are valid:

1. 1. They are in constant random motion.
   2. They are point masses with zero volume.
   3. They are very small compared to the space between them, i.e., they are far apart from one another.
   4. There is no intermolecular forces of attraction or repulsion between them.
   5. No energy is gained or lost during collisions.

Gases that are made up of such particles are called ideal gases. We can therefore mathematically describe the properties of ideal gases by combining Boyle’s law, Charles’ law and Avogadro’s law as follows:

Boyle’s law:           

Charles’ law:         

Avogadro’s law:   

Combining the above laws and rearranging,

where n is defined as the number of moles of gas instead of the earlier arbitrary description of amount of gas and R is the universal gas constant with a value of 8.3145 J K^-1 mol^-1.

Eq14 is called the ideal gas law. Note that the individual laws still apply; for instance, Gay-Lussac’s law is represented by p = k₃T at constant volume for a given mass of gas, where .

Since p₁V₁ = n₁RT₁ and p₂V₂ = n₂RT₂, Avogadro’s law can also be expressed as

Eq14 and eq15 are also known as equations of state, as they interrelate a gas’ physical properties, leading to the knowledge of its physical state. Although these two equations work reasonably well at low pressures, where gases behave ideally, they need to be modified to describe the physical state of gases at high pressures or low temperatures. Gases that are under such non-ideal conditions are called real gases.

 

 

 

Next article: Partial pressure

Previous article: Avogadro’s law

Content page of gas laws

Content page of Basic chemistry

Main content page

Chemical energetics, also known as thermochemistry, is the study of the transformation of energy that occurs during a chemical reaction. It focuses on the exchange of energy between a reaction’s system and its surroundings and utilises the theories of chemical thermodynamics and chemical kinetics to measure, explain and predict these energy exchanges.

 

next article: Endothermic and exothermic reactions

Content page of basic chemical energetics

Content page of Basic chemistry

Main content page

Endothermic and exothermic reactions represent two fundamental categories of chemical processes, with endothermic reactions absorbing heat from their surroundings and exothermic reactions releasing heat, fundamentally influencing temperature changes in their environments.

Chemical reactions involve the breaking of bonds in reactants to give transient or intermediate chemical structures, and the formation of bonds within or between these structures to give the products (see diagram below).

For a reaction system that is not isolated from its surroundings, energy is absorbed by the reaction system from the surroundings during the breaking of bonds and is stored as potential energy, which is then released from the reaction system to the surroundings during the formation of bonds. Hence, energy is neither created or destroyed but is changed from one form to another, and flows from the surroundings to the system or vice versa.

 

 

Potential energy profile diagrams, with the vertical axis representing potential energy and the horizontal axis representing the progress of a reaction, are used to illustrate energy changes during a reaction. The amounts of energy involved in the processes of bond breaking and bond formation do not exactly match. Consider the case of a reaction depicted in the diagram below.

The energy change in the bond breaking process, q₁ = E_i – E_rt (i.e. energy with respect to the intermediate structures minus the energy with respect to the reactants), is positive. The energy change in the bond formation process, q₂ = E_p – E_i (i.e. energy with respect to the products minus the energy with respect to the intermediate structures), is negative. The total change in energy of the reaction is the energy with respect to the products minus the energy with respect to the reactants:

Substituting q₂ = E_p – E_i and q₁ = E_i – E_rt in eq1

For the reaction depicted in the above diagram, q_net > 0. In general, a reaction that requires more energy in the process of bond breaking (energy absorbed from surroundings) than in the process of bond formation (energy released to the surroundings), resulting in the system having a net positive energy (net energy absorbed from surroundings), is called an endothermic reaction.

Conversely, a reaction that requires more energy in the process of bond formation (energy released to the surroundings) than in the process of bond breaking (energy absorbed from surroundings), resulting in a system having a net negative energy (net energy released to the surroundings, q_net < 0), is called an exothermic reaction (see diagram below).

 

next article: The change in enthalpy of a reaction

Previous article: Overview

Content page of basic chemical energetics

Content page of Basic chemistry

Main content page

The change in enthalpy of a reaction is the net transfer of heat of the reaction at constant pressure.

As explained in the previous article, we can use eq1 or eq2 to calculate the change in energy of a reaction. It is difficult to measure the absolute potential energies of the products and reactants, E_p and E_rt. It is also hard to separately quantify the heat transferred from the surroundings to the system q₁ during the bond breaking process, and the heat transferred from the system to its surroundings q₂ during the bond forming process. However, q_net, the net change in energy during the reaction, is easily measured through experiments. Since many chemical reactions occur at constant pressure, the net transfer of heat q_net at constant pressure is denoted by ΔH, which is called the change in enthalpy of the system. Consequently, the potenital energy profile diagrams are replaced with enthalpy diagrams:

which can also be in the form of enthalpy curves:

where E_a is the activation energy of the reaction, i.e. the minimum amount of energy needed for the reactants to form products.

An endothermic reaction is therefore also defined as a reaction where its change of enthalpy is positive and an exothermic reaction is a reaction where its change of enthalpy is negative. An example of an endothermic reaction is the thermal decomposition of calcium carbonate where net energy is absorbed to breakdown the carbonate into the products:

An example of an exothermic reaction is the combustion of methane where net energy is released in the formation of the relatively stable products of carbon dioxide and water:

Theoretically, every reaction is reversible to a certain extent. As shown in the diagram below, the reverse reaction (of any reaction) has an equal magnitude of enthalpy change as the forward reaction but with an opposite sign, e.g. the reverse reaction of the combustion of methane is:

Note that the activation energy for the reverse reaction is

 

next article: Heat capacity and specific heat capacity

Previous article: Endothermic and exothermic reactions

Content page of basic chemical energetics

Content page of Basic chemistry

Main content page

The following are some devices for measuring enthalpy changes of reactions.

A calorimeter (see above diagram) allows the change in temperature of the surroundings of a reaction system to be measured, and hence, the change in enthalpy of the reaction*.

The bomb calorimeter is useful for measuring the change in enthalpy of combustion reactions, e.g.

The sample is placed in a crucible enclosed in a chamber made of a thermally conductive material. Oxygen is pumped into the chamber and the reaction is initiated by a current flowing through a wire embedded in the sample. All the energy released by the combustion reaction is assumed to flow across the chamber walls to heat up the water in the reservoir (surroundings). The change in enthalpy of the reaction is calculated by noting the change in temperature of the water reservoir. For example,

Mass of Ca used:                                      0.001000 kg

Mass of water in the reservoir:          0.4500 kg

Temperature change recorded:          13.10 K

Specific heat capacity of water:        4186 J K^-1 kg^-1

Using eq5, the enthalpy change for the reaction is:

We have further assumed that heat gained by the chamber walls, and heat lost to the atmosphere, are negligible. The enthalpy change for one mole of calcium is:

Therefore,

It is important to note that the enthalpy change quoted for a reaction changes when we modify the stoichiometric coefficients of the equation. For instance, the quoted ΔH doubles if we rewrite the above equation as:

In other words, we interpret ΔH = -990 kJmol^-1 as the enthalpy change per one mole of calcium or half a mole of oxygen or one mole of calcium oxide for the reaction . Similarly, ΔH = -1980 kJmol^-1 is the enthalpy change for two moles of calcium or one mole of oxygen or two moles of calcium oxide in the reaction .

Another perhaps less confusing way to quote the enthalpy change of a reaction is to drop the ‘per mole’ part of the unit to give:

We use a solution calorimeter (see above diagram) when we want to measure the enthalpy change of an aqueous reaction, e.g. the neutralisation of hydrochloric acid with sodium hydroxide:

Consider the reaction of 20.0 cm³ of 1.000 M HCl with 30.0 cm³ of 1.000 M NaOH resulting in an increase in temperature of 5.46 K. Note that water is considered the surroundings in this experiment, i.e. the reactants are dissolved in their surroundings. There are 0.0200 moles of HCl in 20.0 cm³ of water and 0.0300 moles of NaOH in 30.0 cm³ of water, which means that the total volume of solution is 50.0 cm³. Assuming that the density of the solution is the same as the density of water (1.00 g cm^-3), the mass of the solution is 50.0 g. If we make the further assumption that the specific heat capacity of the solution is the same as that of water (4.18 Jg^-1K^-1), the change in enthalpy of the reaction is:

Since HCl is the limiting reagent, the enthalpy change per mole of H₂O is:

 

* The bomb calorimeter actually measures the change in internal energy of the system, which we approximate it to be equal to the change of enthalpy. This is because the system in the bomb calorimeter is a constant-volume environment instead of a constant-pressure environment.

 

next article: Bond enthalpy

Previous article: Heat capacity and specific heat capacity

Content page of basic chemical energetics

Content page of Basic chemistry

Main content page

The bond enthalpy (also known as bond energy) is the average change in energy required to break one mole of a bond in the gaseous state, e.g. the bond enthalpy for breaking one mole of hydrogen-hydrogen bonds is:

Bond enthalpies are always positive as reaction systems absorb energy to break bonds.

The reason for including the term ‘average’ in the definition of bond enthalpy is that some bonds, e.g. the carbon-hydrogen bond, are present in different types of molecules:

Methane:                    H — CH₃

Methanol:                   H — CH₂OH

Methanoic acid:       H — COOH

The bond dissociation energy, which is a specific value and not an average value, for the carbon-hydrogen bond in methane, is different from that in methanol, which is in turn different from that in methanoic acid. This is because the electron density in each carbon-hydrogen bond is influenced by its neighbouring atoms, which are different for each compound. Furthermore, each of the four carbon-hydrogen bonds in methane has a different bond dissociating energy if we were to remove the hydrogen atoms successively, since the electronic environment of the molecule changes from one fragment to another. The table below shows the various bond dissociation energies for one mole of each carbon-hydrogen bond for methane:

Bond	Bond dissociation energy (kJmol-1)
H — CH3	435
H — CH2	444
H — CH	444
H — C	339

Therefore, the average bond dissociation energy for one mole of the carbon-hydrogen bond across a range of compounds is called the bond enthalpy of the carbon-hydrogen bond and is ΔH_be = +413 kJmol^-1. The table below shows bond enthalpies of some common bonds:

Bond	Bond enthalpy, (kJmol-1)
	435
	416
	348
	612
	837
	743
	146
	498
	460
	243
	366
	192

Even though bond enthalpy, ΔH_be, is defined as the average change in energy required to break one mole of a bond in the gaseous state, the magnitude of the change in enthalpy to break one mole of a particular bond, , is the same as the magnitude of the change in enthalpy to form one mole of the same bond, . Hence, the data in the bond enthalpy table can be used to represent both and .

 

next article: Using bond enthalpies to Calculate the change in enthalpy of reactions

Previous article: Measuring the change in enthalpy of a reaction (Part 2)

Content page of basic chemical energetics

Content page of Basic chemistry

Main content page

The heat capacity C of a substance of mass m is the net amount of heat q_net needed to change its temperature T by one Kelvin. As mentioned in the previous article, the net amount of heat absorbed or released by a system at constant pressure is equal to the change in enthalpy of the system, q_net = ΔH, which can also be written as q_sys = ΔH_sys. This net amount of heat absorbed or released, respectively raises or lowers the temperature of a substance in the system T_sys. The heat capacity of a substance of mass m in a system at constant pressure is therefore:

where C has units of J K^-1.

The specific heat capacity c of a substance in a system is the heat capacity of the substance divided by the mass of the substance. In other words, it is the net amount of heat absorbed or released by the substance in the system that changes one kilogram of the substance by a temperature of one Kelvin. At constant pressure, we have

where c has units of J K^-1 kg^-1.

It is important to remember that ΔH_sys is the transfer of heat content at constant pressure between a system and its surroundings. If ΔH_sys > 0, heat is transferred from the surroundings to the system, in which case, the temperature of the system increases, while the temperature of the surroundings decreases (assuming that the surroundings is a limited volume of solid, liquid or gas). Conversely, if ΔH_sys < 0, heat is transferred from the system to its surroundings, in which case, the temperature of the system decreases, while the temperature of the surroundings increases.

An example of the application of eq3 is the measure of the change in enthalpy of a known mass of liquid that is heated from T₁ to T₂ (see diagram above). In this experiment, we consider the system as the liquid in the container and the surroundings as the Bunsen burner. Using eq3,

Since T₂ > T₁ and both c and m are positive, ΔH_sys > 0 and the liquid in the system undergoes an endothermic process.

The above experiment measures the change in temperature of the system to arrive at the value of ΔH_sys. We can also determine ΔH_sys indirectly by measuring the change in temperature of the surroundings. Consider the following experiment to measure ΔH_sys of an exothermic reaction:

If the system and its surroundings are initially at the same temperature, any thermal energy produced by the reaction increases the temperature of the system, causing the flow of heat q from the system to its surroundings. Since we are measuring the temperature change of the surroundings, eq3 becomes,

where c and m are the specific heat capacity of material in the surroundings and the mass of material in the surroundings respectively.

By the theory of conservation of energy, any heat gained in the surroundings is heat lost by the system, i.e.

Substituting q_sur = –q_sys in eq4, we have q_sys = – cmΔT_sur or

Since most experiments are designed to indirectly measure the change in temperature of the surroundings to obtain ΔH_sys, eq5 is usually used to analyze the enthalpy change of a chemical reaction in a system instead of eq3. For an exothermic reaction, the temperature of the surroundings increases (ΔT_sur > 0) and according to eq5, ΔH_sys < 0. For an endothermic reaction, ΔT_sur < 0 and therefore ΔH_sys > 0.

In the next article, we shall look at some actual experiment setups for measuring enthalpy changes of reactions.

 

next article: Measuring the change in enthalpy of a reaction

Previous article: The change in enthalpy of a reaction

Content page of basic chemical energetics

Content page of Basic chemistry

Main content page

In the article on ‘Measuring the change in enthalpy of a reaction (Part 2)’, the change in enthalpy of a reaction is determined via calorimetry. Enthalpy change of reactions can also be estimated using bond enthalpy data.

Rewriting eq2 in terms of enthalpy,

where ΔH is the change in enthalpy of the reaction, ΔH_bf is the bond enthalpy of formation (ΔH_bf < 0) and ΔH_bb is the bond enthalpy of breaking (ΔH_bb > 0).

Since both ΔH_bb and ΔH_bf are taken from the same bond enthalpy data set, which has all numbers quoted as positive values, eq6 becomes

or to be specific, if there are multiple bonds broken and multiple bonds formed:

In other words,

The change in enthalpy of a reaction is the difference between the sum of the magnitude of bond enthalpies of all bonds broken and the sum of the magnitude of bond enthalpies of all bonds formed.

 

 

Previous article: Bond enthalpy

Content page of basic chemical energetics

Content page of Basic chemistry

Main content page