Atomic structure: an introduction

Atomic structure forms the foundation of chemistry and physics, providing the key to understanding matter at its most fundamental level. Early experiments on atomic behavior, such as those exploring the emission spectrum of hydrogen, played a pivotal role in shaping our modern view of the atom.

Just before Rutherford proposed his model of the atom, Johann Balmer, a Swiss scientist, conducted an experiment to analyse the emission spectrum of hydrogen. This happened in the late 1800s when the wave theory of light was universally accepted by scientists.

The experiment involves using a high potential difference to create an electric spark to split hydrogen gas into atoms in a discharge tube. The excited hydrogen atoms emit a pink radiation, which is separated by a prism into different wavelengths (see diagram below). These spectral lines in the visible range of the electromagnetic spectrum are collectively known as the Balmer series.

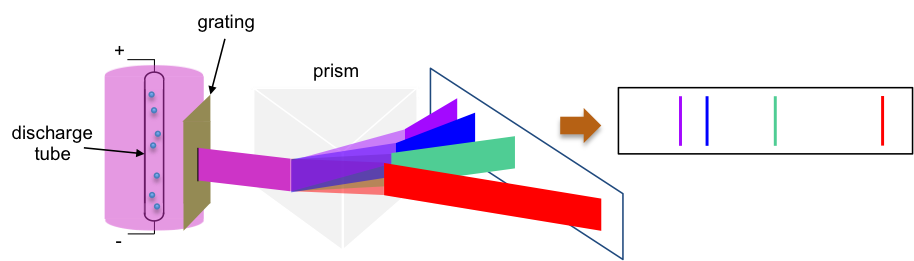
Three years after Balmer published his results, Johannes Rydberg, a Swedish physicist developed an empirical formula in 1888 to accurately calculate the wavelengths of spectral lines of the Balmer series for hydrogen. The formula, known as the Rydberg formula, is:

where λ is the wavelength of a spectral line, R_H is the Rydberg constant with the value 1.097 x 10⁷ m^-1, and n₁ and n₂ are integers with n₂ > n₁.

As the formula was developed empirically, it could not explain why excited hydrogen atoms emitted light of specific wavelengths. Niels Bohr attempted to answer this problem by proposing a new model of the atom with the following postulates:

1. 1. Electrons can only revolve around the nucleus in stable orbits of certain discrete radii. These orbits are called energy shells.
   2. Orbits further away from the nucleus exist at higher energies. The orbitals are denoted by n = 1,2,3…, with a higher-number orbit being further away from the nucleus.
   3. Electrons gain or lose energy in the form of electromagnetic radiation when they transit between orbits, absorbing energy when they transit from a lower energy orbit to a higher one and emitting energy when they fall from a higher energy orbit to a lower one.

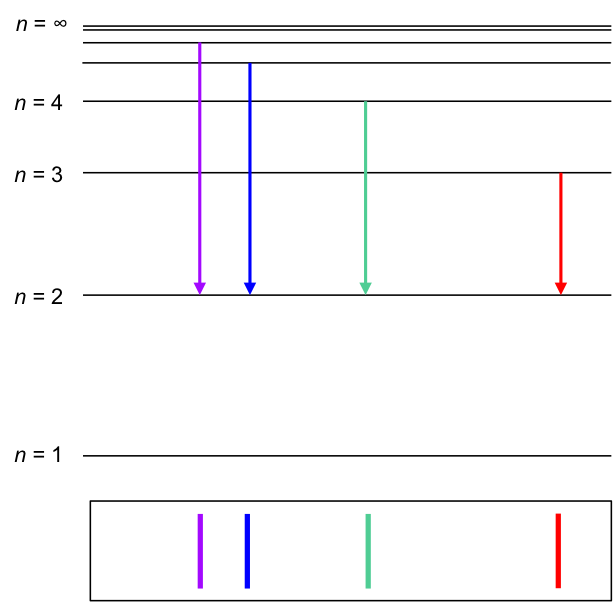
Using the above postulates, Bohr was also able to derive the Rydberg formula theoretically (see this article for details). He explained that when hydrogen gas was split into atoms in Balmer’s experiment, the sole electron in a hydrogen atom was promoted from the n = 1 shell to higher energy shells (n > 2). When the electron fell back down to the n = 2 shell, light of different wavelengths were emitted:

• • 656.3 nm for n = 3 to n = 2, corresponding to red light
  • 486.1 nm for n = 4 to n = 2, corresponding to blue-green light
  • 434.1 nm for n = 5 to n = 2, corresponding to blue-violet light
  • 410.2 nm for n = 6 to n = 2, corresponding to violet light

n₁ and n₂ in the Rydberg formula now corresponds to energy shells of an electron transition, where n₁ refers to the lower energy shell and n₂, the higher energy shell.

 

 

Bohr also suggested that each shell contained a limited number of electrons. For elements heavier than hydrogen, electrons occupy the lower energy shells first. When the limit of electrons in a shell is reached, excess electrons occupy a higher energy shell. Even though Bohr’s derivation of the Rydberg formula was later proven by quantum mechanics to be mathematically sound, his model has certain shortcomings, e.g. according to the model, an electron orbiting around the nucleus would constantly radiate electromagnetic energy and eventually crash into the nucleus. The Bohr model also could not derive the maximum number of electrons for each shell, which was later proven by quantum mechanics to be 2n² (see below table). Nevertheless, he was awarded the Nobel Prize in Physics in 1922 for his work.

Shell number or principal quantum number (n)	Maximum number of electrons
1	2
2	8
3	18
4	32

 

 

 

Previous article: Atomic theory

Content page of basic quantum chemistry

Content page of Basic chemistry

Main content page