Category: Basic Chemistry

A chemical equation describes the changes that occur during a chemical reaction.

Instead of writing an equation using words, for example:

1 part of solid zinc & 2 parts of hydrochloric acid gives 1 part of zinc chloride & 1 part of hydrogen gas

we express it as:-

or simply (by omitting the number 1, which is quite redundant):

In general, a chemical equation has the form:

where

1. 1. The upper-case letters represent elements, ionic formula or molecular formula (each entity is known as a chemical species);
   2. The lower-case letters (known as stoichiometric coefficients) represent the relative portions of chemical species in the reaction;
   3. The letters in brackets indicate the physical state of the elements or molecules (s for solid, l for liquid, g for gas and aq for aqueous, i.e. a species dissolved in water); and
   4. A single arrow or a double arrow is used, depending on whether the reaction is non-reversible or reversible, respectively.

Since the ratio of stoichiometric coefficients for the chemical species in the zinc-acid chemical equation above is 1:2:1:1, we say that one mole of zinc reacts with two moles of hydrochloric acid to give one mole zinc chloride and one mole hydrogen gas.

 

NEXT ARTICLE: Balancing a CHEMICAL EQUATION

Previous article: Stoichiometry: overview

Content page of Stoichiometry

Content page of Basic chemistry

Main content page

Stoichiometry is the calculation of the relative amounts of products and reactants in a chemical reaction.

The word stoichiometry comes from the greek words stoicheion, meaning element, and metron, meaning measure. To calculate the amounts of products and reactants in a chemical reaction, we need to first know how to construct and balance a chemical equation.

 

Next article: The chemical equation

Content page of Stoichiometry

Content page of Basic chemistry

Main content page

Chemical kinetics is the study of the rates of chemical reactions. The analysis of the rates of chemical processes involves understanding how different factors like temperature and pressure affect the speed of these processes, which in turn allow us to optimise conditions to synthesise useful products safely and efficiently.

The rate of a reaction is defined as:

with the amount of reactants or products being measured by mass, volume or number of moles.

 

Next article: measuring the rate of a reaction

Content page of basic chemical kinetics

Content page of Basic chemistry

Main content page

Collision theory posits that reactions occur when particles collide with sufficient energy to overcome the potential energy of repulsion between the electron densities of the approaching particles, and often to break and rearrange bonds within those particles.

Therefore, not all collisions successfully result in a reaction. For a reaction to take place, particles must collide with the correct orientation and a minimum amount of energy called the activation energy, E_a, which differs between reactions.

Consider the collision of dinitrogen tetraoxide molecules to form nitrogen dioxide.

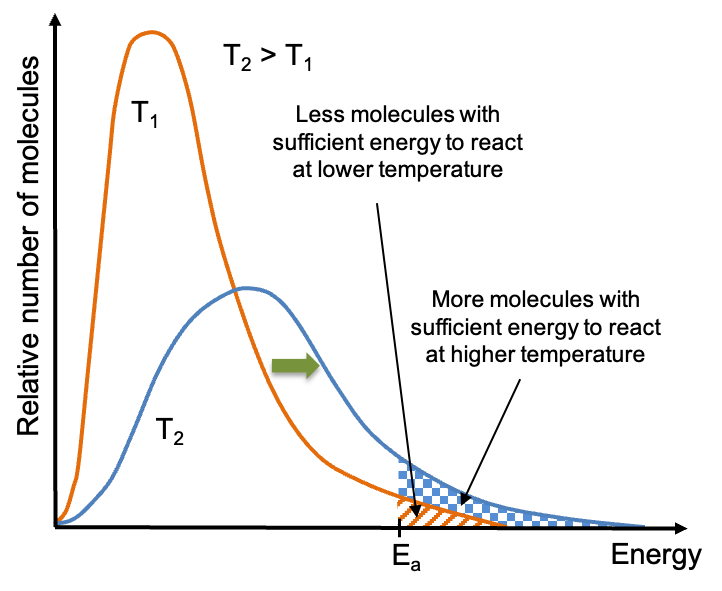
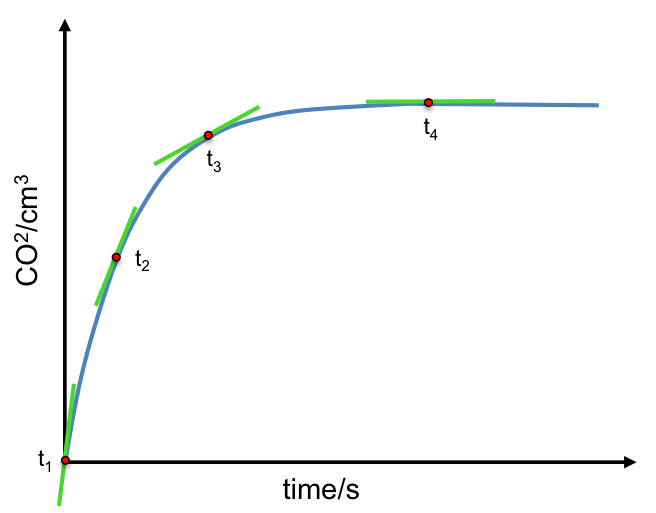
A sample of at a particular temperature consists of molecules with various energies. This is because, after colliding with one another, some molecules gain energy while others lose energy, resulting in the bulk of the molecules having intermediate energies. However, a fraction of them have sufficient energy (≥ E_a) to collide with each other in the correct orientation and dissociate into nitrogen dioxide molecules. Such collisions are called effective collisions.

The different energies of the molecules can be described by the graph above. The graph, known as the Maxwell-Boltzmann distribution, was first derived by James Maxwell, a Scottish scientist in 1860 and later improved upon by Ludwig Boltzmann, an Austrian physicist. The various areas under the curve represent the fraction of molecules with certain energies, with the green area expressing the proportion of molecules with sufficient energies to react.

In general, factors that increase the rate of effective collisions between particles, or provide alternate reaction pathways with lower activation energies, will enhance the rate of the reaction. These factors are:

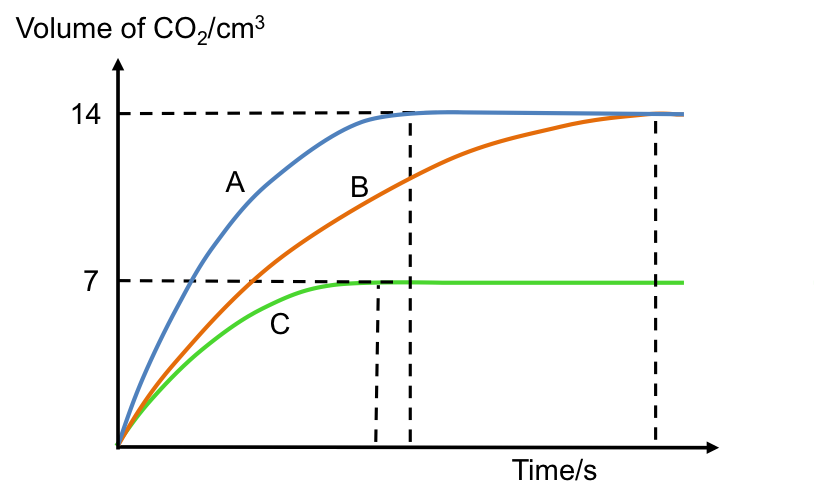
1. 1. Concentration of the reactants (or pressure, if the reactants are gases).
   2. Surface area of the reacting particles.
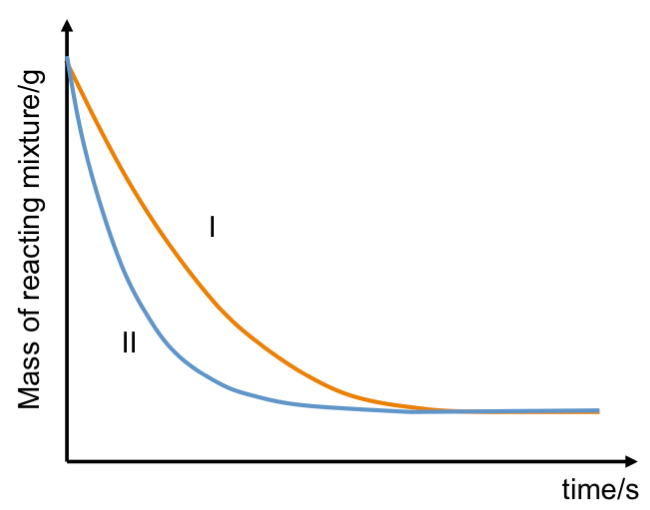
   3. Temperature at which the reaction is occurring.
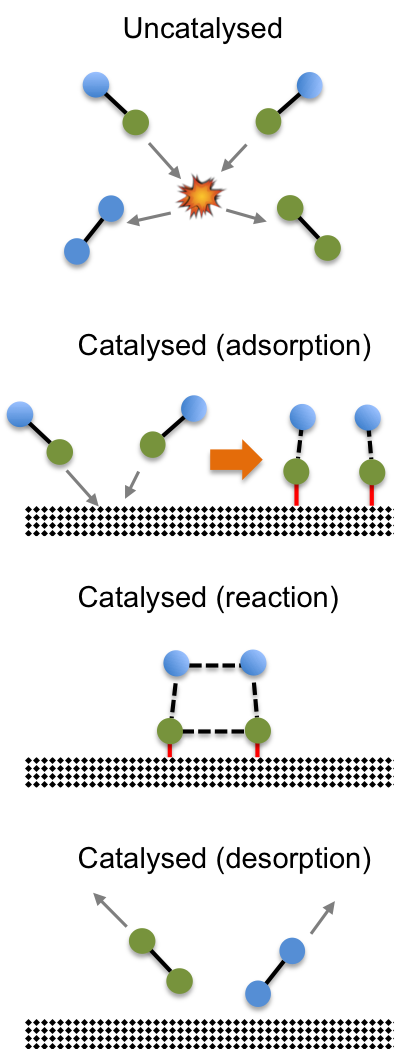
   4. Presence of a catalyst.

 

Next article: Effect of concentration (or pressure) on the rate of a reaction

Previous article: Measuring the rate of a reaction

Content page of basic chemical kinetics

Content page of Basic chemistry

Main content page

The effect of concentration (or pressure) on the rate of a reaction is a key factor in chemical kinetics, as higher concentrations or pressures typically increase the frequency of collisions between reactant molecules, leading to faster reaction rates.

Consider the reaction between an acid and a strip of magnesium:

When we increase the concentration of the acid, we increase the number of hydroxonium ions per unit volume. This leads to a higher frequency of collisions between the hydroxonium ions and the magnesium strip, which in turn increases the probability of collisions occurring with the correct orientation and with energies equal or greater than the activation energy required for the reaction to take place. Similarly, for the reaction , an amount of N₂O₄ that exerts a higher pressure on the walls of a piston chamber implies that more N₂O₄ molecules within the bulk are colliding with one another effectively per unit time. A higher pressure can be obtained by injecting more N₂O₄ into the chamber or decreasing the volume of the chamber. Therefore, an increase in the concentration (or pressure) of reactants increases the rate of a reaction.

 

 

Next article: Effect of surface area of particles on the rate of a reaction

Previous article: Collision theory

Content page of basic chemical kinetics

Content page of Basic chemistry

Main content page

The surface area of particles plays a crucial role in determining the rate of chemical reactions. In a reaction involving a solid reactant, increasing the surface area of the solid enhances the reaction rate. Consider the following reaction:

A larger surface area of calcium carbonate allows more hydroxonium ions to collide with the carbonate molecules, facilitating more frequent and effective interactions. 

As illustrated by the diagram above, a large cube of CaCO₃ has six surfaces with a total area of 24 cm² available for the hydroxonium ions to collide. However, if the cube is broken down into smaller cubes, the total surface area accessible to the hydroxonium ions increases to 48 cm². This leads to a higher frequency of effective collision and therefore, a higher rate of reaction.

 

Next article: Effect of temperature on the rate of a reaction

Previous article: Effect of concentration (or pressure) on the rate of a reaction

Content page of basic chemical kinetics

Content page of Basic chemistry

Main content page

The effect of temperature on the rate of a reaction is a critical aspect of chemical kinetics, as increasing temperature generally enhances molecular energy and collision frequency, resulting in faster reaction rates.

As mentioned in the section on collision theory, the energies of a gaseous reactant are distributed over a range that can be described by the Maxwell-Boltzmann distribution. These energies are mainly kinetic energies, which means that the gas particles are moving with different velocities. When we increase the temperature of a reaction mixture, we increase the average kinetic energy of the particles and hence their average velocities. This results in a higher rate of effective collisions between the reactants and therefore a higher rate of reaction.

The energy profiles of a reactant at various temperatures are shown on the Maxwell-Boltzmann distribution diagram above. At higher temperatures, the energy distribution curve shifts to the right with more reactant molecules reaching and exceeding the activation energy, E_a, of the reaction. Since the total number of molecules for the two curves must be the same (i.e. the area under the curve at T₁ must be equal to that at T₂), the curve at the higher temperature, with respect to the one at the lower temperature, is expected to flatten as the number of molecules with higher energies increases.

The effect of temperature on the rate of reactions can be observed in everyday life, for example, the spoiling of a bottle of milk that is left in the summer sun and the preservation of animal corpses that are buried deep in snow.

 

Next article: Effect of a catalyst on the rate of a reaction

Previous article: Effect of surface area of particles on the rate of a reaction

Content page of basic chemical kinetics

Content page of Basic chemistry

Main content page

A catalyst is a substance that increases the rate of a reaction without itself being chemically changed at the end of the reaction. Catalysts can be categorised into two groups: homogeneous and heterogeneous.

A homogeneous catalyst is one that is in the same phase as the reactants, while a heterogeneous catalyst is one that is in a different phase as the reactants. Consider the decomposition of aqueous hydrogen peroxide to oxygen:

The reaction occurs very slowly under normal conditions due to its high activation energy. If a homogeneous catalyst, e.g. hydrobromic acid HBr, is added, the reaction proceeds rapidly with the following two-step mechanism:

In the first step, a molecule of H₂O₂, in the presence of H⁺, oxidises a bromide ion (oxidation state -1) to aqueous hypobromous acid HOBr (oxidation state of Br is +1), while it is reduced to water. In the second step, another molecule of H₂O₂ reduces the key intermediate HOBr back to Br^– and H⁺, with itself being oxidised to a molecule of oxygen. If we add the two equations, we get eq2. You’ll realise that the hydrogen and bromide ions participate in the reaction but are not consumed in the overall process.

 

 

So, instead of the high activation energy route of two H₂O₂ colliding with each other to give oxygen, a suitably chosen catalyst offers a lower activation energy pathway of H₂O₂ colliding with a bromide ion, and subsequently with hypobromous acid, to produce oxygen. This is illustrated by the energy profile diagram above. With a lower activation energy, the rate of the reaction increases, as a larger proportion of molecules have sufficient energy to react.

The same reaction can be catalysed by a heterogeneous catalyst such as manganese dioxide. The mechanism for heterogeneous catalysis can be complex but normally involves the adsorption of reactants onto the surface of the catalyst, which changes the bond strengths of the reactants. The reactants, now being in close proximity to one another, react to form the products, which are desorbed or released from the surface of the catalyst (see diagram below).

 

The catalyst, one again, participates in the reaction but remains chemically unaltered at the end of the reaction. Just like the example of the homogeneous catalyst, a heterogeneous catalyst offers an alternate reaction pathway for the reaction to occur. Instead of the high activation energy route of molecules colliding with one another to form products, a heterogeneous catalyst offers a lower activation energy pathway by allowing the molecules to be adsorbed onto its surface, where they react to form products.

 

 

In summary, a catalyst:

1. 1. Increases the rate of a reaction because it lowers the activation energy of the reaction by offering an alternate pathway for the reaction to proceed. For a reversible reaction, a catalyst increases the rates of both the forward and reverse reactions by the same factor because the activation energy is lowered for the reaction in both directions.
   2. Functions selectively, that is, it increases the rate of only certain reactions.
   3. Is required only in small amounts to increase the rate of reactions, as it is not consumed but reused in the process. Nevertheless, increasing the concentration of the catalyst further increases the rate of a single-step reaction, assuming that the reactants are in excess.
   4. May be inactivated by impurities over time.
   5. Can be denatured by heat if the catalyst is an enzyme.

Some common catalysts include:

1. 1. Iron in the Haber process for the manufacture of ammonia.
   2. Vanadium (V) oxide in the Contact process for the manufacture of sulphuric acid.
   3. Platinum, palladium or rhodium in catalytic converters.
   4. Nickel, platinum and palladium in the hydrogenation of alkenes to alkanes.
   5. Phosphoric acid on silica for the hydration of alkenes to alcohols.
   6. Concentrated acids for esterification.
   7. Biological enzymes produced by yeast in the conversion of sugar or starch to ethanol.

 

Previous article: Effect of temperature on the rate of a reaction

Content page of basic chemical kinetics

Content page of Basic chemistry

Main content page

There are many ways to measure the rate of a reaction, some of which are depicted in the diagram below.

 

The rate of a reaction that produces a gas can be monitored by measuring the volume of gas produced at different time with a syringe, or by noting the pressure of the gas at various time intervals with a pressure gauge (A). Another way to determine the rate of a reaction is to measure the change in mass of a reaction mixture over time (B).  We can also measure the rate of a reaction via colorimetry (C) if a reacting species is coloured, and by titrimetric methods (D) if a reacting species can be oxidised or reduced by a titrant.

The rate of reaction between hydrochloric acid and calcium carbonate to give carbon dioxide is an example that can be determined by either method A or B.

With respect to the syringe method A, the volumes of CO₂ recorded are plotted against time (see diagram below), where the gradient at any point on the curve is the change of the amount of product versus the change in time, i.e. the rate of the reaction given by eq1.

The graph shows that the gradient is the steepest at the beginning of the reaction, t₁, which means that the rate of reaction is fastest at the beginning. As the reaction progresses, the gradient decreases at t₂ and t₃, implying that the rate of reaction is decreasing. This is due to decreasing concentration of reactants, which are converted to products over time. Finally at t₄, the gradient is zero, signifying that the reaction has stopped.

The above rates that are measured at specific times of the reaction are called instantaneous rates of reaction. We can also measure the average rate of reaction over a certain period of time just like we measure the average speed of a car over a certain stretch of a journey. For example, the average rate of reaction for the production of CO₂ over the 1^st five minutes of the reaction is:

where is the volume of CO₂ at x seconds.

Since a reaction’s rate generally decreases over time, we need a way to compare the rates of reaction between different reactions. This can be done using the initial rate of reaction, which happens to be a very useful analytical tool in chemical kinetics. Conceptually, the initial rate of reaction is the rate of reaction just after the start of the reaction when t ≈ 0, i.e.we measure the initial rate of reaction immediately after the beginning of the reaction. As elaborated in subsequent sections, this is a good reference point to compare the rates of reaction of reactants with different concentrations because their concentrations are assumed to be approximately the same as that before the start of their respective reactions.

 

Next article: Collision theory

Previous article: OVerview

Content page of basic chemical kinetics

Content page of Basic chemistry

Main content page

John Dalton, an English chemist, experimented on the behaviour of gas mixtures in 1801 and concluded that:

The total pressure exerted by a mixture of non-reacting gases is equal to the sum of the partial pressures of each gas.

This became known as Dalton’s law of partial pressures. The law is best understood using an ideal gas, which is described by the ideal gas law, pV = nRT.

Consider an ideal gas consisting of a mixture of chemical species in a rigid container at constant temperature. Since ideal gas particles are assumed to be point masses with elastic collisions and no intermolecular forces of interaction, they are expected to collide onto the walls of the container independently from one another in the mixture. The total pressure exerted by the gas mixture on the walls of the container is therefore proportional to the total number of particles of ideal gases in the container. The ideal gas law then becomes:

where p_T is the total pressure of the gas mixture and n_i is the number of moles of gas i in the mixture.

Expanding eq16 gives:

where p_i is known as the partial pressure of gas i:

Dividing eq18 with eq16 and rearranging yields:

where is the mole fraction of gas i.

Even though eq17 and eq19 are derived from the ideal gas law, they are applicable to real gases in many situations, especially in their dilute forms.

 

 

 

Previous article: Ideal gas law

Content page of gas laws

Content page of Basic chemistry

Main content page