The heat capacity C of a substance of mass m is the net amount of heat qnet needed to change its temperature T by one Kelvin.
As mentioned in the previous article, the net amount of heat absorbed or released by a system at constant pressure is equal to the change in enthalpy of the system, qnet = ΔH, which can also be written as qsys = ΔHsys. This net amount of heat absorbed or released, respectively raises or lowers the temperature of a substance in the system Tsys. The heat capacity of a substance of mass m in a system at constant pressure, by definition, is therefore:
where C has units of J K-1.
The specific heat capacity c of a substance in a system is the heat capacity of the substance divided by the mass of the substance. In other words, it is the net amount of heat absorbed or released by the substance in the system that changes one kilogramme of the substance by a temperature of one Kelvin. At constant pressure, we have
where c has units of J K-1 kg-1.
It is important to remember that ΔHsys is the transfer of heat at constant pressure between a system and its surroundings. If ΔHsys > 0, heat is transferred from the surroundings to the system, in which case, the temperature of the system increases, while the temperature of the surroundings decreases (assuming that the surroundings is a limited volume of solid, liquid or gas). Conversely, if ΔHsys < 0, heat is transferred from the system to its surroundings, in which case, the temperature of the system decreases, while the temperature of the surroundings increases.
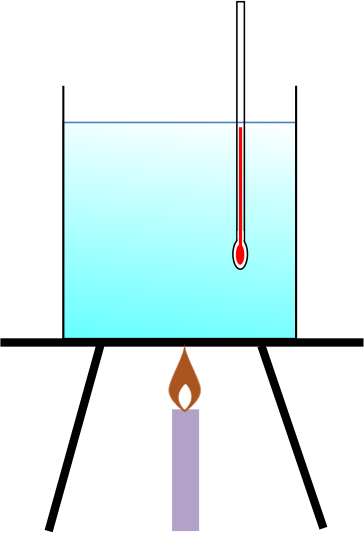
An example of the application of eq3 is the measure of the change in enthalpy of a known mass of liquid that is heated from T1 to T2 (see diagram above). In this experiment, we consider the system as the liquid in the container and the surroundings as the Bunsen burner. Using eq3,
Since T2 > T1 and both c and m are positive, ΔHsys > 0 and the liquid in the system undergoes an endothermic process.
The above experiment measures the change in temperature of the system to arrive at the value of ΔHsys. We can also determine ΔHsys indirectly by measuring the change in temperature of the surroundings. Consider the following experiment to measure ΔHsys of an exothermic reaction:
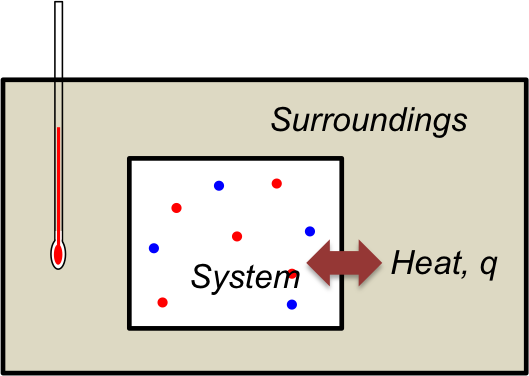
If the system and its surroundings are initially at the same temperature, then any thermal energy produced by the reaction increases the temperature of the system, causing the flow of heat q from the system to its surroundings. Since we are measuring the temperature change of the surroundings, eq3 becomes,
where c and m are the specific heat capacity of material in the surroundings and the mass of material in the surroundings respectively.
By the theory of conservation of energy, any heat gained in the surroundings is heat lost by the system, i.e.
Substituting qsur = –qsys in eq4, we have qsys = – cmΔTsur or
Since most experiments are designed to indirectly measure the change in temperature of the surroundings to obtain ΔHsys, eq5 is usually used to analyse the enthalpy change of a chemical reaction in a system instead of eq3. For an exothermic reaction, the temperature of the surroundings increases (ΔTsur > 0) and according to eq5, ΔHsys < 0. For an endothermic reaction, ΔTsur < 0 and therefore ΔHsys > 0.
In the next article, we shall look at some actual experiment setups for measuring enthalpy changes of reactions.