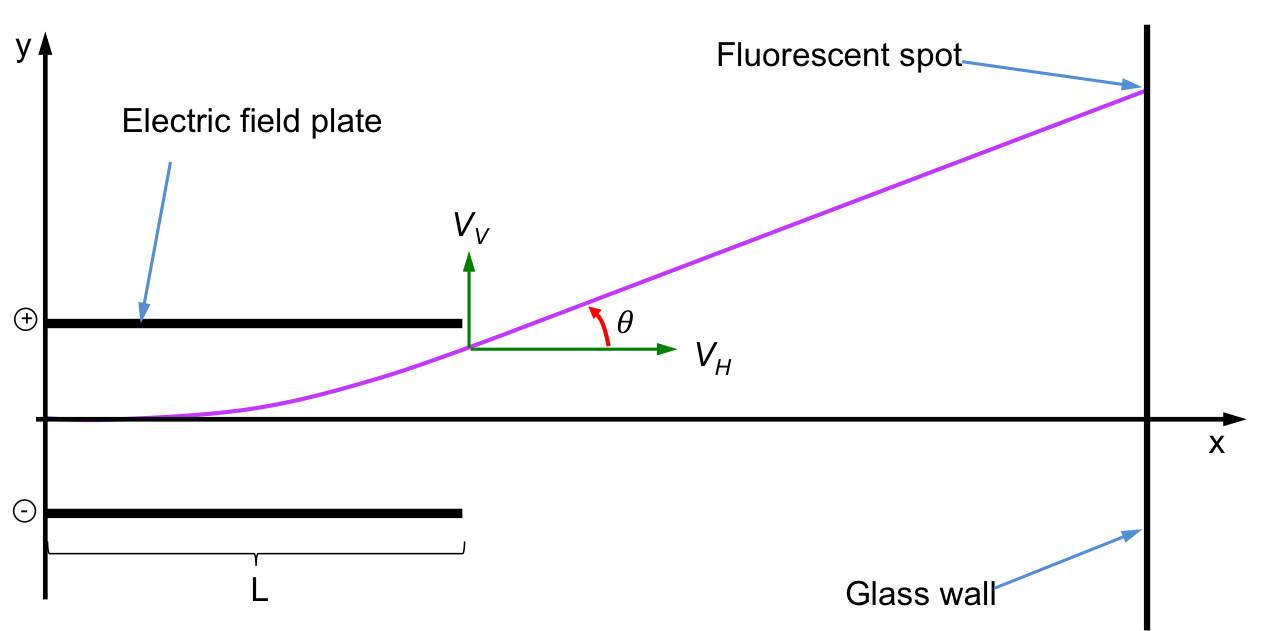
Mass spectrometry in the early days focuses on determining the charge-to-mass ratio, e/m, of an electron. This involves a few steps, beginning with the electric field turned on and the magnetic field turned off. The diagram below shows the mechanics of the electrons as they pass through the electric field plates.
An electric force, Fe, acts on an electron with charge, e, passing through the electric field, E, in the x-direction where
and gives the electron with mass, m, an acceleration, a:
Combining eq1 and eq2,
As the electric force acts only in the y-direction, the velocity of the electron in the x-direction remains constant at vH. The velocity of the electron in the y-direction, vV, is zero at the point where it enters the electric field and increases to a maximum value at the point where the electron leaves the electric field. This maximum value of vV remains constant after the electron exits the electric field and is given by:
where S is the displacement of the electron in the y-direction, u is the initial velocity of the electron in the y-direction before entering the electric field (i.e. u = 0) and t is the time during which the electric force acts on the electron.
In other words, t is the time taken for the electron to travel the length of the electric field plate, L. Therefore,
The trigonometric relationship between vH and vV after the electron leaves the electric field is:
The electric field strength is adjusted so that the angle of deflection is small. Therefore, tanθ ≈ θ and eq5 becomes,
The value of θ is recorded, leaving vH as the only unknown variable (in eq6) for the calculation of e/m. To determine vH , the magnetic field, B, is turned on while the electric field is still on. Using Fleming’s left hand rule, the magnetic force, FB, acts on the electron (at the point when the electron just enters the magnetic field from the left side) and deflects it in the negative y-direction:
The magnetic field is then adjusted to the extent that the angle of deflection of the cathode ray is zero, meaning Fe = FB. Substituting eq1 and eq7 in Fe = FB, we have:
Substitute eq8 in eq6
The values of all the variables on the right hand side of eq9 are now known and the charge-to-mass ratio e/m of the electron can be determined. The magnitude of e/m that Thomson obtained ranged from 0.7 x 1011 C/kg to 2 x 1011 C/kg. The currently accepted value is 1.758820024 x 1011 C/kg.
Finally, in 1909, when Robert Millikan determined the charge of an electron via his famous oil drop experiment, the estimated mass of an electron was calculated.