One-particle, time-independent Schrodinger equation

The one-particle, time-independent Schrodinger equation is a partial differential equation whose solutions are the one-particle, time-independent wave functions of quantum-mechanical systems.

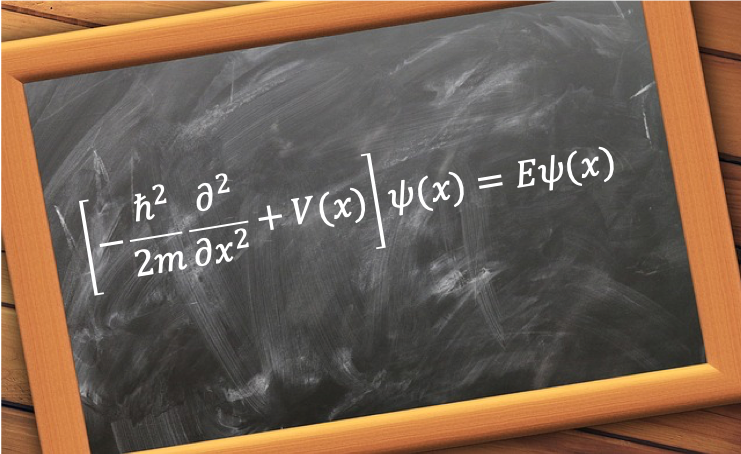
Even though it is widely regarded as a postulate, we can list the scientific findings that could have inspired Schrodinger to develop the equation.

Year	Development	Formula	Scientist
1500s	Equations of motion	Linear rotational motion equations	Many scientists including Galileo and Newton
1746	1-D classical wave equation, whose solutions are wave functions that describe the motion of waves.	$\frac{\partial^{2}u(x,t)}{\partial x^{2}}=\frac{1}{c^{2}}\frac{\partial^{2}u(x,t)}{\partial t^{2}}$	Jean d’Alembert
1924	de Broglie’s hypothesis: all matter exhibit wave-like properties	$p=\frac{h}{\lambda}$	Louis de Broglie

Many scientists at that time were trying to develop models of the atom, in particular, the behaviour of electrons in an atom. It is possible that when de Broglie proposed that all matter have wave—like properties, Schrodinger thought that an equation similar to the classical wave equation could be derived to describe the wave motion of an electron in an atom. Since the classical wave equation is solved by the separation of variables method, we can express $u(x,t)$ as $u(x,t)=h(x)g(t)=\psi(x)cos\omega t$ . Substituting $u(x,t)$ in the classical wave equation, we have:

$cos\omega t\frac{\partial^{2}}{\partial x^{2}}\psi(x)=-\frac{1}{c^{2}}\psi(x)\omega^{2}cos\omega t$

$\frac{\partial^{2}}{\partial x^{2}}\psi(x)+\frac{\omega^{2}}{c^{2}}\psi(x)=0$

Substitute $\omega=2\pi v$ and $c=v\lambda$ in the above equation

$\frac{\partial^{2}}{\partial x^{2}}\psi(x)+\frac{4\pi^{2}}{\lambda^{2}}\psi(x)=0\; \; \; \; \; \; \; \; 43$

The total energy of an electron is $E=KE+PE=\frac{p^{2}}{2m}+V(x)$ or $p=\sqrt{2m[E-V(x)]}$ , where $p$ is the momentum of the electron. Substituting $p$ in de Broglie’s relation, we have $\sqrt{2m[E-V(x)]}=\frac{h}{\lambda}$ , which we then substitute in eq43 to give:

$\frac{\partial^{2}}{\partial x^{2}}\psi(x)+\frac{2m}{\hbar^{2}}[E-V(x)]\psi(x)=0$

where $\hbar=\frac{h}{2\pi}$ , or

$\hat{H}\psi=E\psi\; \; \; \; \; \; \; \; 44$

where $\hat{H}=-\frac{\hbar^{2}}{2m}\frac{\partial^{2}}{\partial x^{2}}+V$ .

$\hat{H}$ is called the Hamiltonian operator and Eq44 is the one-dimensional, one-particle time-independent Schrodinger equation. As it is an eigenvalue equation, $\psi$ (known as a wave function) is an eigenfunction and $E$ is the corresponding eigenvalue. For a three-dimensional, one-particle system, the Hamiltonian is:

$\hat{H}=-\frac{\hbar^{2}}{2m}\nabla^{2} +V(x,y,z)\; \; \; \; \; \; \; \; 45$

where $\nabla^{2}=\frac{\partial^{2}}{\partial x^{2}}+\frac{\partial^{2}}{\partial y^{2}}+\frac{\partial^{2}}{\partial z^{2}}$ and is called the Laplacian.

One-particle, time-independent Schrodinger equation

Next article: one-particle, time-independent schrodinger equation in spherical coordinates

Previous article: postulates of quantum mechanics

Content page of quantum mechanics

Content page of advanced chemistry

Main content page

Leave a Reply Cancel reply