Relation between magnetic dipole moment and angular momentum

In classical electrodynamics, a magnetic dipole moment $\mu$ is associated with a current loop (see diagram below), and represents the magnitude and orientation of a magnetic dipole. It is a pseudo-vector, whose direction is perpendicular to the plane of the loop and given by the right hand thumb rule.

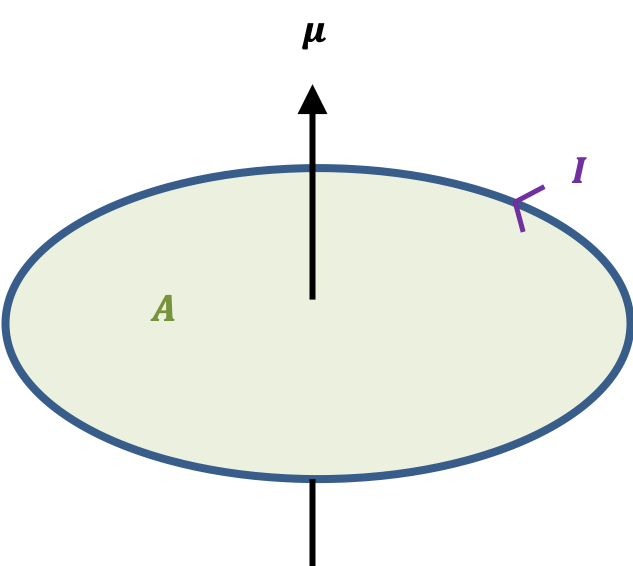
The magnitude of a magnetic dipole moment of a current loop is defined as

$\mu=IA\; \; \; \; \; \; \; \; 60$

where $I$ is current, and $A$ is the area of the loop.

Rewriting eq60 in terms of $I=\frac{q}{t}=\frac{qv_{\perp}}{2\pi r}$ (where $q$ is charge, $t$ is time and $v_{\perp}$ is the tangential velocity of the charged particle) and using $A=\pi r^{2}$ , we have $\mu=\frac{q}{2m}rmv_{\perp}$ , where $m$ is the mass of the charged particle. Substitute eq59 in $\mu=\frac{q}{2m}rmv_{\perp}$ , we have the relation between magnetic dipole moment and angular momentum:

$\boldsymbol{\mathit{\mu}}=\gamma\boldsymbol{\mathit{L}}\; \; \; \; \; \; \; \; 61$

where $\gamma=\frac{q}{2m}$ is the classical gyromagnetic ratio.

When placed in an external magnetic field , the magnetic dipole moment experiences a torque, whose energy is

$U=-\boldsymbol{\mathit{\mu}}\cdot\boldsymbol{\mathit{B}}\; \; \; \; \; \; \; \; 62$

Question

How is eq62 derived?

Answer

In order to rotate a current loop, we must do work $W$ against the torque $\tau$ due to the magnetic field $\boldsymbol{\mathit{B}}$ . For a rotating system (see diagram I below), $W=-\int F_{\perp}ds$ , where according to convention, the negative sign is added so that work on the system by the field is positive.

For small $\theta$ , $ds=rd\theta$ . Since $\boldsymbol{\mathit{\tau}}=\boldsymbol{\mathit{r}}\times\boldsymbol{\mathit{F}}$ and $W=-\Delta U$ , we have

$U_f-U_i=\int F_{\perp}ds=\int_{\theta_i}^{\theta_f}Fsin\theta rd\theta=\int_{\theta_i}^{\theta_f}\tau d\theta\; \; \; \; \; \; \; \; 62a$

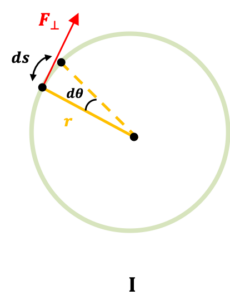
Diagram II below shows a square current loop with side 1 parallel to side 3 and side 2 parallel to side 4 (not shown in diagram). The length of each side is $b$ .

Since $\tau=r\times F=\frac{b}{2}Fsin\theta+\frac{b}{2}Fsin\theta$ and $F=BIb$ ( $\theta=90^{\circ}$ ),

$U_f-U_i=\int_{\theta_i}^{\theta_f}IBb^{2}sin\theta\, d\theta=IA B\left (- cos\theta_f+cos\theta_i \right)\; \; \; \; \; \; \; \; 62b$

where $A$ is the area of the loop.

Substitute eq60 in the above equation,

$U_f-U_i=\mu B\left (cos\theta_i-cos\theta_f \right)\; \; \; \; \; \; \; \; 63$

Comparing eq62a and eq62b, torque can also be expressed as

$\boldsymbol{\mathit{\tau}}=BIAsin\theta=\boldsymbol{\mathit{\mu}}\times\boldsymbol{\mathit{B}}\; \; \; \; \; \; \; \; 64$

The torque exerted by the magnetic field on the magnetic dipole tends to rotate the dipole towards a lower energy state. So, we let $\theta_i=90^{\circ}$ with $U_i=0$ and eq63 becomes $U_f=-\mu Bcos\theta_f$ , or simply:

$U=-\boldsymbol{\mathit{\mu}}\cdot \boldsymbol{\mathit{B}}\; \; \; \; \; \; \; \; 65$

Other than a torque, the external magnetic field may exert another force on the current loop. For a magnetic field pointing in the $z$ -direction, $\boldsymbol{\mathit{B}}=B_z(x,y,z)\boldsymbol{\mathit{k}}$ , where $B_z(x,y,z)$ is a scalar function. We substitute eq65 in $F_z=-\frac{\partial U}{\partial z}$ to give

$\boldsymbol{\mathit{F_z}}=\frac{\partial(\boldsymbol{\mathit{\mu}}\cdot\boldsymbol{\mathit{B}})}{\partial z}=\frac{\partial[(\mu_x\boldsymbol{\mathit{i}}+\mu_y\boldsymbol{\mathit{j}}+\mu_z\boldsymbol{\mathit{k}})\cdot B_z(x,y,z)\boldsymbol{\mathit{k}}]}{\partial z}=\mu_z\frac{\partial B_z(x,y,z)}{\partial z}\; \; \; \; \; \; \; \; 66$

where $\boldsymbol{\mathit{i}}$ , $\boldsymbol{\mathit{j}}$ and $\boldsymbol{\mathit{k}}$ are unit vectors, and $\frac{\partial B_z(x,y,z)}{\partial z}$ is the gradient of the external magnetic field along the $z$ -direction.

For a uniform field, $B_z(x,y,z)=B_0$ (i.e. a constant) and $\boldsymbol{\mathit{F_z}}=0$ ; while for an inhomogenous field, $B_z(x,y,z)=B_0+\alpha z$ , where $\alpha$ is a scalar representing the change in $B_0$ along the $z$ -axis, and $\boldsymbol{\mathit{F_z}}\neq 0$ .

Substituting eq61 in eq62,

$U=-\gamma\boldsymbol{\mathit{B}}\cdot\boldsymbol{\mathit{L}}\; \; \; \; \; \; \; \; 67$

For an inhomogenous magnetic field pointing in the $z$ -direction, the above equation becomes

$U=-\gamma(B_0+\alpha z)\boldsymbol{\mathit{k}}\cdot(L_x\boldsymbol{\mathit{i}}+L_y\boldsymbol{\mathit{j}}+L_z\boldsymbol{\mathit{k}})=-\gamma(B_0+\alpha z)L_z\; \; \; \; \; \; \; \; 68$

Eq68 is used as a starting point in analysing results from the Stern-Gerlach experiment.

Question

Does the magnetic field $\boldsymbol{\mathit{B}}=(B_0+\alpha z)\boldsymbol{\mathit{k}}$ violate Maxwell’s 2^nd equation of $\nabla\cdot\boldsymbol{\mathit{B}}=0$ where $\nabla=\frac{\partial}{\partial x}\boldsymbol{\mathit{i}}+\frac{\partial}{\partial y}\boldsymbol{\mathit{j}}+\frac{\partial}{\partial z}\boldsymbol{\mathit{k}}$ ?

Answer

The field should be $\boldsymbol{\mathit{B}}=-\alpha x\boldsymbol{\mathit{i}}+(B_0+\alpha z)\boldsymbol{\mathit{k}}$ , which satisfies $\nabla\cdot\boldsymbol{\mathit{B}}$ . However, the precession of the spin magnetic moment of a silver atom around $B_0$ in the Stern-Gerlach experiment is so fast that the $x$ -component of the spin moment averages to zero, resulting in an effective field of $\boldsymbol{\mathit{B}}=(B_0+\alpha z)\boldsymbol{\mathit{k}}$ interacting with the atom.

Question

Why is $\nabla\cdot\boldsymbol{\mathit{B}}=0$ ?

Answer

A simple way of showing $\nabla\cdot\boldsymbol{\mathit{B}}=0$ is to consider a two-dimensional diagrammatic representation of the vector function $\boldsymbol{\mathit{B}}=-y\boldsymbol{\mathit{i}}+x\boldsymbol{\mathit{j}}$ below, where each point in the two-dimensional space is associated with a vector.

When $y=0$ , the successive points in the negative $x$ direction and the positive $x$ direction are associated with the vectors $-\boldsymbol{\mathit{j}},-2\boldsymbol{\mathit{j}},\cdots$ and $\boldsymbol{\mathit{j}},2\boldsymbol{\mathit{j}},\cdots$ respectively. Similarly, when $x=0$ , the successive points in the negative $y$ direction and the positive $y$ direction are associated with the vectors $\boldsymbol{\mathit{i}},2\boldsymbol{\mathit{i}},\cdots$ and $-\boldsymbol{\mathit{i}},-2\boldsymbol{\mathit{i}},\cdots$ respectively. Clearly, $\nabla\cdot\boldsymbol{\mathit{B}}=0$ .