The Einstein-Podolsky-Rosen paradox (EPR paradox), conceived in 1935, proposes that a particle (or a system) has definite attributes (i.e., physical properties) prior to any measurements. It also suggests that each particle is influenced only by its immediate surroundings (locally), rather than by another particle at a distance. This contrasts with the statistical interpretation of a particle’s state as theorised by quantum mechanics, where the certainty of an attribute exists only after a measurement is made, and particles are considered entangled.
Let’s examine the case of the decay of a spin-0 particle at rest into two spin- particles with zero relative orbital angular momentum, and the subsequent passing of the two particles through two Stern-Gerlach devices. Setting aside the EPR paradox for a moment, experimental results show that if the spin of the first particle, which is measured in the
-direction, is an up spin, the spin of the second particle measured in the same direction is always a down spin. This is of course due to the principle of conservation of spin angular momentum. If we instead measure the spin of the second particle in the
-direction (see diagram below), we may record either an up spin or a down spin.
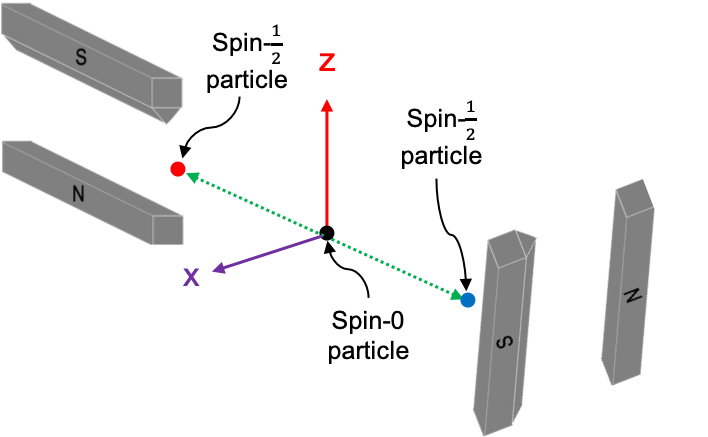
By repeating the experiment, say a hundred times, the results (assuming a random distribution of spins after decay) are as follows:-
| Particle 1 (measured in |
Particle 2 (inferred in |
Particle 2 (measured in |
||
| Population | Population | |||
| 50 | 25 | |||
| 25 | ||||
| 50 | 25 | |||
| 25 | ||||
where and
under
and
refer to up spins, while
and
refer to down spins.
The data revealed that amongst all the fifty runs of particle 1 returning results of up spins in the -direction, twenty five corresponding particle 2 have up spins in the
-direction, while the remaining twenty five have down spins. Similarly, for all fifty runs of particle 1 returning results of down spins in the
-direction, twenty five corresponding particle 2 have up spins in the
-direction, while the remaining twenty five have down spins.
Quantum theory, which states that we can only be certain that a system possesses an attribute after a measurement is made, accurately predicted the above results via eq226. The EPR paradox, on the other hand, proposes that each particle must have the definite spin angular momentum information necessary for the Stern-Gerlach device to reveal before the particle passes through it. This suggests that the population of particle 2 can be categorised as follows:
| Particle 2 |
which implies that the corresponding particle 1 can be grouped along with particle 2 as:
| Groups, |
Particle 1 | Particle 2 |

Question
Does such a categorisation of spin- particle pairs violate the uncertainty principle, which states that we cannot precisely determine the
-component of the spin angular momentum and the
-component of the spin angular momentum of a particle simultaneously?
Answer
No, because we are only measuring the spin angular momentum of each particle either in the -direction or the
-direction but not in both directions simultaneously.
Therefore, it appears that the EPR paradox is consistent with the results obtain from the double Stern-Gerlach device measurements for orthogonal axes. If is a variable that is measurable, we would be able to distinguish the particles. The EPR paradox suggests that such a variable is hidden from us (a hidden variable) and that quantum mechanics is incomplete. This challenge to quantum mechanics remained unresolved until John Bell, in 1964, conceptualised a method to determine whether quantum mechanics or the EPR paradox is correct.