The binomial theorem (or binomial expansion) expresses how to expand the power of a sum of two variables into a series of terms involving the individual powers of each variable, with coefficients determined by their positions in the expansion.
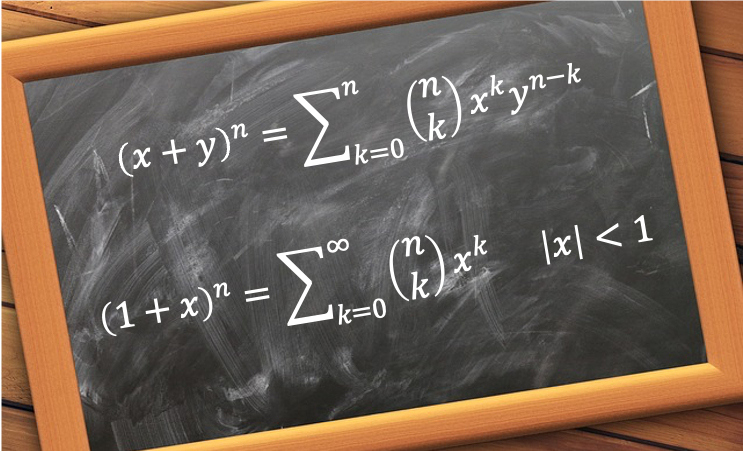
In general, the binomial expansion of is
where are variables,
and
are non-negative integers and
are the binomial coefficients.
The binomial theorem can be proven by induction. For , we have
If the theorem holds for all when
, then for
,
Substituting for the first summation yields
. Changing the index from
back to
gives

Question
Show that .
Answer
Therefore,
and the theorem holds for all and
.
The binomial theorem defines . This is evident from eq319, where
The binomial series, on the other hand, is a more general concept where can be any real number, including negative and fractional values. If one of the variables in eq319 is equal to one and the absolute value of the other variable is less than one, the binomial series provides a way to express an infinite series that converges: