Matrix elements of an operator

The matrix elements of an operator $\hat{O}$ are the entries of the matrix representation of the operator.

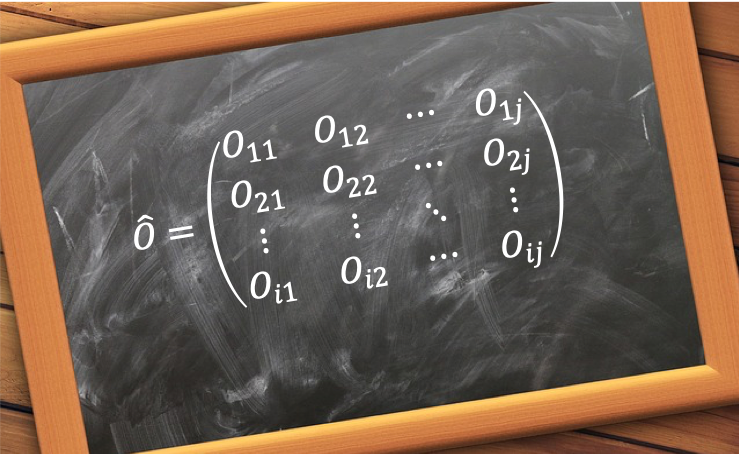
Consider a linear map from a vector space $\vert\psi\rangle$ to the same vector space, i.e. $\vert\phi\rangle=\hat{O}\vert\psi\rangle$ , where $\vert\psi\rangle,\vert\psi\rangle\in V$ and the orthonormal basis states $\vert\varphi_n\rangle$ span $V$ . The matrix representation of the equation is

$\begin{pmatrix} \phi_1\\\phi_2 \\ \vdots \end{pmatrix}=\begin{pmatrix} O_{11} &O_{12} &\cdots \\ O_{21} &O_{22} &\cdots \\ \vdots & \vdots &\ddots \end{pmatrix}\begin{pmatrix} \psi_1\\\psi_2 \\ \vdots \end{pmatrix}$

where $\phi_m$ and $\psi_n$ are the coefficients of the vectors $\vert\phi\rangle$ and $\vert\psi\rangle$ respectively.

The matrix elements of $\vert\phi\rangle$ are given by

$\phi_m=\sum_{n}O_{mn}\psi_n\; \; \; \; \; \; \; \; 32a$

Since the orthonormal basis states $\vert\varphi_n\rangle$ span $V$ , we have $\vert\psi\rangle=\psi_1\vert\varphi_1\rangle+\psi_2\vert\varphi_2\rangle+\cdots=\sum_n\vert\varphi_n\rangle\langle\varphi_n\vert\psi\rangle$ . So,

$\vert\phi\rangle=\hat{O} \sum_{n}\vert\varphi_n\rangle\langle\varphi_n\vert\psi\rangle =\sum_{n}\hat{O}\vert\varphi_n\rangle\langle\varphi_n\vert\psi\rangle$

$\langle\varphi_m\vert\phi\rangle=\sum_{n}\langle\varphi_m\vert\hat{O}\vert\varphi_n\rangle\langle\varphi_n\vert\psi\rangle$

Similarly, $\vert\phi\rangle=\phi_1\vert\varphi_1\rangle+\phi_2\vert\varphi_2\rangle+\cdots$ and so

$\phi_m=\sum_{n}\langle\varphi_m\vert\hat{O}\vert\varphi_n\rangle\psi_n\; \; \; \; \; \; \; \; 33$

Comparing eq33 with eq32a, $O_{mn}=\langle\varphi_m\vert\hat{O}\vert\varphi_n\rangle$ . Therefore, $O_{mn}$ are the matrix elements of $\hat{O}$ with respect to the basis states of $\vert\varphi_n\rangle$ .

Matrix elements of an operator

Next article: projection operator

Previous article: spectral decomposition of an operator

Content page of quantum mechanics

Content page of advanced chemistry

Main content page

Leave a Reply Cancel reply