What is the formula of the titration curve of a monoprotic weak acid versus a monoprotic strong base?
We make use of eq1 from the previous article except that Ca is now the concentration of a weak acid. Since the weak acid is partially ionised in water, at any point of the titration, the sum of the number of moles of HA and A– must equal to the number of moles of HA if it were undissociated. As the change in volume of the solution is common to all ions,
where Va and Vb are the volume of weak acid in the solution and the volume of strong base in the solution respectively. Substitute in eq5 where Ka is the dissociation constant of HA and rearranging, we have,
Assuming that the strong base is fully ionised in water, at any point of the titration, the sum of the number of moles of B+ must equal to the number of moles of BOH added to the solution. As the change in volume of the solution is common to all ions,
Substitute eq6, eq7, Kw = [H+][OH–] and [H+] = 10-pH in eq1,
Eq8 is the complete pH titration curve for a monoprotic weak acid versus monoprotic strong base system. We can input it in a mathematical software to generate a curve of pH against Vb. For example, if we titrate 10 cm3 of 0.200 M of CH3COOH (Ka = 1.75 x 10-5) with 0.100 M of NaOH, we have the following:
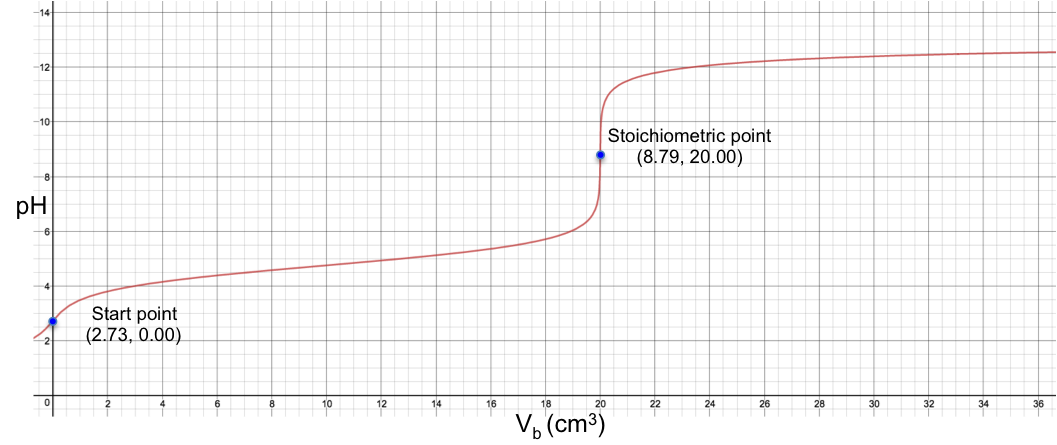
To understand the change in pH near the stoichiometric point versus the change in Vb, we assume that one drop of base is about 0.05 cm3 and substitute 19.95 cm3, 20.00 cm3 and 20.05 cm3 into eq8. The respective pH just before and just after the stoichiometric point (SP) are:
|
|
One drop before SP | SP |
One drop after SP |
|
Volume, cm3 |
19.95 | 20.00 |
20.05 |
|
pH |
7.36 | 8.79 |
10.22 |
The data shows that two drops of base cause a change of 2.86 in pH before and after the stoichiometric point. Since the change in pH at the stoichiometric point is smaller than that of a strong acid versus strong base titration, we can use fewer indicators that work within the range to monitor the titration. Lastly, we can derive the gradient equation for a weak acid versus strong base titration and investigate the inflexion point at pH 8.79 by differentiating eq8 implicitly (see previous article), resulting in , i.e. a gradient that makes angle of 89.51o with the horizontal.
