Normalisation constant for the wavefunctions of the quantum harmonic oscillator

The normalisation constant ensures that the wavefunctions of the quantum harmonic oscillator are properly scaled, thereby maintaining the probabilistic interpretation of quantum states.

To derive the normalisation constant for the wavefunctions of the quantum harmonic oscillator, we begin with the multiplication of eq33 by $e^{-y^2}$ and by itself (with a change of the dummy variable $t$ to $s$ and the dummy index $n$ to $m$ ) to give

$e^{-y^2}e^{-t^2+2ty}e^{-s^2+2sy}=e^{-y^2}\sum_{m=0}^{\infty}\sum_{n=0}^{\infty}\frac{H_m(y)}{m!}\frac{H_n(y)}{n!}s^mt^n\;\;\;\;\;\;\;\;41$

Integrating with respect to $y$ ,

$\int_{-\infty}^{\infty}e^{-y^2-t^2+2ty-s^2+2sy}dy=\int_{-\infty}^{\infty}e^{-y^2}\sum_{m=0}^{\infty}\sum_{n=0}^{\infty}\frac{H_m(y)}{m!}\frac{H_n(y)}{n!}s^mt^ndy$

Substituting the orthogonal relation $\int_{-\infty}^{\infty}e^{-y^2}H_m(y)H_n(y)dy=0$ when $m\neq n$ in the above equation and simplifying, we have

$e^{2st}\int_{-\infty}^{\infty}e^{-(y-s-t)^2}dy=\sum_{n=0}^{\infty}\frac{(st)^n}{n!n!}\int_{-\infty}^{\infty}e^{-y^2}\[H_n(y)\]^2dy\;\;\;\;\;\;\;\;42$

Question

Show that $I=\int_{-\infty}^{\infty}e^{-(y-s-t)^2}dy=\sqrt{\pi}$ .

Answer

Let $X=y-s-t$ and $dX=dy$ . So, $I=\int_{-\infty}^{\infty}e^{-X^2}dX$ with the limits unchanged. At the same time, let $I=\int_{-\infty}^{\infty}e^{-Y^2}dY$ , where we have changed the dummy variable $X$ to $Y$ .

$I^2=\int_{-\infty}^{\infty}e^{-X^2}dX\int_{-\infty}^{\infty}e^{-Y^2}dY=\int\int_{-\infty}^{\infty}e^{X^2+Y^2}dXdY$

In polar coordinates, $r^2=X^2+Y^2,X=rcos\theta,Y=rsin\theta$ . For infinitesimal changes, the change in area $dA$ can be approximated as a rectangle with sides $r$ and $rd\theta$ (see diagram below), with $dXdY=dA=rd\theta dr$ .

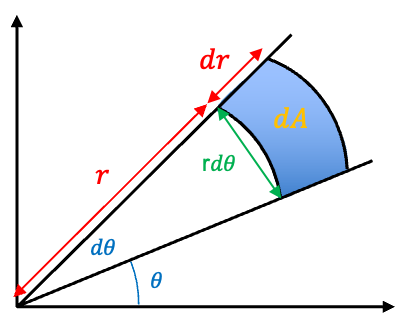
So,

$I^2=\int_{0}^{2\pi}d\theta\int_{0}^{\infty}re^{-r^2}dr=2\pi\int_{0}^{\infty}re^{-r^2}dr$

Let $u=r^2\;\Rightarrow\;du=2rdr$

$I^2=2\pi\int_{0}^{\infty}re^{-u}\frac{du}{2r}=\pi\int_{0}^{\infty}e^{-u}du=\pi$

$I=\int_{-\infty}^{\infty}e^{-(y-s-t)^2}dy=\sqrt{\pi}\;\;\;\;\;\;\;\;43$

Using eq43 and the Maclaurin series expansion of $e^x=\sum_{n=0}^{\infty}\frac{x^n}{n!}$ , eq42 becomes

$\sqrt{\pi}\sum_{n=0}^{\infty}\frac{2^n(st)^n}{n!}=\sum_{n=0}^{\infty}\frac{(st)^n}{n!n!}\int_{-\infty}^{\infty}e^{-y^2}\[H_n(y)\]^2dy\;\;\;\;\;\;\;\;44$

Expanding eq44 and comparing the coefficients of $(st)^n$ ,

$n=0$	$\int_{-\infty}^{\infty}e^{-y^2}\[H_n(y)\]^2dy=\sqrt{\pi}$
$n=1$	$\int_{-\infty}^{\infty}e^{-y^2}\[H_n(y)\]^2dy=2\sqrt{\pi}$
$n=2$	$\int_{-\infty}^{\infty}e^{-y^2}\[H_n(y)\]^2dy=8\sqrt{\pi}$
$n=3$	$\int_{-\infty}^{\infty}e^{-y^2}\[H_n(y)\]^2dy=48\sqrt{\pi}$
$\vdots$	$\vdots$
$n=n$	$\int_{-\infty}^{\infty}e^{-y^2}\[H_n(y)\]^2dy=2^nn!\sqrt{\pi}$

In an earlier article, we have made a change of variable of ${x=y\biggr$\frac{\hbar}{m\omega}\biggr$^{\frac{1}{2}}}$ in deriving the un-normalised wavefunction of the quantum harmonic oscillator. Since $x$ is the variable representing position in the one-dimensional space that the oscillator is moving in, the normalisation of the wavefunction refers to an integral with respect to $x$ , i.e.

$N^2\int_{-\infty}^{\infty}e^{-\frac{m\omega}{\hbar}x^2}\biggr\[H_n\biggr$\sqrt{\frac{m\omega}{\hbar}}x\biggr$\biggr\]^2dx=1\;\;\;\;\;\;\;\;45$

Using ${dx=\biggr$\frac{\hbar}{m\omega}\biggr$^{\frac{1}{2}}}dy$ , eq45 is equivalent to

$N^2\biggr$\frac{\hbar}{m\omega}\biggr$^{\frac{1}{2}}\int_{-\infty}^{\infty}e^{-y^2}\[H_n(y)\]^2dy=1$

Therefore,

$N^2\biggr$\frac{\hbar}{m\omega}\biggr$^{\frac{1}{2}}2^nn!\sqrt{\pi}=1\;\;or\;\;N=\sqrt[4]{\frac{m\omega}{\pi\hbar}}\frac{1}{\sqrt{2^nn!}}$

The normalised wavefunction is then

$\psi_n(y)=\sqrt[4]{\frac{m\omega}{\pi\hbar}}\frac{1}{\sqrt{2^nn!}}H_n(y)e^{-\frac{y^2}{2}}\;\;\;n=0,1,2,\cdots\;\;\;\;\;\;\;\;45$

$\psi_n(x)=\sqrt[4]{\frac{m\omega}{\pi\hbar}}\frac{1}{\sqrt{2^nn!}}H_n\biggr$\sqrt{\frac{m\omega}{\hbar}}x\biggr$e^{-\frac{m\omega}{2\hbar}x^2}\;\;\;n=0,1,2,\cdots\;\;\;\;\;\;\;\;46$

Question

Show that $\psi_n(x)$ is either an even function or an odd function.

Answer

An even function is defined as $f(x)=f(-x)$ , while and odd function is when $-f(x)=f(-x)$ . So, $e^{-\frac{m\omega}{2\hbar}x^2}$ is an even function. On the other hand, $H_n\biggr$\sqrt{\frac{m\omega}{\hbar}}x\biggr$$ is an even function if $n$ is even and an odd function if $n$ is odd. Since the product of two even functions is an even function, while the product of an odd function and an even function is an odd function, $\psi_n(x)$ is an even function when $n$ is even and an odd function if $n$ is odd.

Normalisation constant for the wavefunctions of the quantum harmonic oscillator

Question

Answer

Question

Answer

Next article: Vibration of diatomic molecules

Previous article: Generating function and Rodrigues’ formula for the Hermite polynomials

Content page of vibrational spectroscopy

Content page of advanced chemistry

Main content page

Leave a Reply Cancel reply