The quantum harmonic oscillator

The quantum harmonic oscillator is a model for studying an atom that moves back and forth about an equilibrium point. The model’s central premise involves the use of the potential energy term of the classical harmonic oscillator to construct the Schrodinger equation for the harmonic oscillator:

$\biggr$\frac{p^2}{2m}+\frac{1}{2}kx^2\biggr$\psi(x)=E\psi(x)\;\;\;\;\;\;\;\;4$
which is equivalent to

$\biggr$-\frac{\hbar^2}{2m}\frac{d^2}{dx^2}+\frac{1}{2}kx^2\biggr$\psi(x)=E\psi(x)$

where $x$ is the displacement of the mass from its equilibrium position.

Question

Show that $\frac{p^2}{2m}$ is equivalent to $-\frac{\hbar^2}{2m}\frac{d^2}{dx^2}$ .

Answer

The de Broglie relation is $p=\hbar k$ , where $k=\frac{2\pi}{\lambda}$ . The derivative of the wavefunction $\psi=Ae^{ikx}$ with respect to $x$ is

$\frac{d\psi}{dx}=ikAe^{ikx}=ik\psi$

$\frac{\hbar}{\sqrt{2m}}\frac{d}{dx}\psi=i\frac{\hbar}{\sqrt{2m}}k\psi=i\frac{p}{\sqrt{2m}}\psi$

This suggests that the operator $\frac{\hbar}{\sqrt{2m}}\frac{d}{dx}$ is equivalent to $i\frac{p}{\sqrt{2m}}$ , and hence, $\hat{p}=\frac{\hbar}{i}\frac{d}{dx}$ . Squaring the momentum operator $\hat{p}$ and dividing by $2m$ gives $-\frac{\hbar^2}{2m}\frac{d^2}{dx^2}$ .

Substituting $k=m\omega^2$ in the above equation and rearranging, we have

$\frac{d^2}{dx^2}\psi(x)+\biggr$\frac{2m}{\hbar^2}E-\frac{m^2\omega^2}{\hbar^2}\biggr$\psi(x)=0\;\;\;\;\;\;\;\;5$

The probability of locating an electron in the Hilbert space must be finite, i.e. $\int_{-\infty}^{\infty}\vert\psi(x)\vert^2dx<\infty$ . This implies that $\psi(x)$ vanishes as $x\rightarrow\pm\infty$ . We call such a well-behaved wavefunction square-integrable. Hence, we need to study the asymptotic characteristics of eq5 to find possible solutions.

Let’s begin by simplifying the differential equation using a change of variable. Substituting $x=y\biggr$\frac{\hbar}{m\omega}\biggr$^{1/2}$ and $\epsilon=\frac{E}{\hbar\omega}$ in eq5, we have

$\frac{d^2\psi(y)}{dy^2}+\(2\epsilon-y^2)\psi(y)=0\;\;\;\;\;\;\;\;6$

Since $2\epsilon$ is a constant, $y^2\gg 2\epsilon$ as $y\rightarrow\pm\infty$ , and eq6 approximates to

$\frac{d^2\psi(y)}{dy^2}-y^2\psi(y)=0\;\;\;\;\;\;\;\;7$

This suggests that the solution to eq6 could be a Gaussian function because $\psi(y)=e^{-\frac{y^2}{2}}$ is a solution to eq7. Let’s try $\psi(y)=u(y)e^{-\frac{y^2}{2}}$ , where $u(y)$ is a function of $y$ . Substituting $\psi(y)=u(y)e^{-\frac{y^2}{2}}$ in eq6 and computing the derivatives, we have

$\frac{d^2u(y)}{dy^2}-2y\frac{du(y)}{dy}+(2\epsilon-1)u(y)=0\;\;\;\;\;\;\;\;8$

Eq8 is known as the Hermite differential equation.

Question

Show that we can use a power series to solve eq8 around $y=0$ .

Answer

When $y=0$ , eq8 simplifies to $\frac{d^2u(y)}{dy^2}+c^2u(y)=0$ , where $c=\sqrt{2\epsilon-1}$ . It can be easily verified that $u=Acos(cy)$ and $u=Bsin(cy)$ are solutions to $\frac{d^2u(y)}{dy^2}+c^2u(y)=0$ . As the Taylor series for $u=Acos(cy)$ and $u=Bsin(cy)$ are $u=A\sum_{k=0}^{\infty}(-1)^k\frac{c^{2k}x^{2k}}{(2k)!}$ and $u=B\sum_{k=0}^{\infty}(-1)^k\frac{c^{2k+1}x^{2k+1}}{(2k+1)!}$ , respectively, a power series is a solution to eq8 around $y=0$ .

The above Q&A suggests that a possible solution to eq8 is a power series for a range of values of $y$ . Let $u(y)=\sum_{n=0}^{\infty}C_ny^n$ and substitute $\frac{du(y)}{dy}=\sum_{n=0}^{\infty}nC_ny^{n-1}$ and $\frac{d^2u(y)}{dy^2}=\sum_{n=0}^{\infty}n(n-1)C_ny^{n-2}$ in eq8 to give

$\sum_{n=0}^{\infty}n(n-1)C_ny^{n-2}-2y\sum_{n=0}^{\infty}nC_ny^{n-1}+(2\epsilon-1)\sum_{n=0}^{\infty}C_ny^{n}=0$

Substituting $2y\sum_{n=0}^{\infty}nC_ny^{n-1}=2\sum_{n=0}^{\infty}nC_ny^n$ and $\sum_{n=0}^{\infty}n(n-1)C_ny^{n-2}=\sum_{n=0}^{\infty}(n+2)(n+1)C_{n+2}y^n$ in the above equation, we have

$\sum_{n=0}^{\infty}\[(n+2)(n+1)C_{n+2}-2nC_n+(2\epsilon-1)C_n\]y^n=0$

The above equation is only true for all values of $y$ if every coefficient for each power of $y$ is zero. So, $(n+2)(n+1)C_{n+2}-2nC_n+(2\epsilon-1)C_n=0$ , which rearranges to

$C_{n+2}=\frac{2n+1-2\epsilon}{(n+2)(n+1)}C_n\;\;\;\;\;\;\;\;9$

Eq9 is a recurrence relation. If we know the value of $C_0$ , we can use the relation to find $C_2,C_4,C_6,\cdots$ . Similarly, if we know $C_1$ , we can find $C_3,C_5,C_7,\cdots$ .

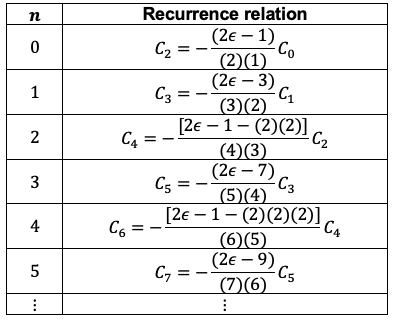
Comparing the recurrence relations for even-labelled coefficients,

$C_{2n}=-\[2\epsilon-1-2(2n-2)\]\frac{(2n-2)!}{(2n)!}C_{2n-2}\;\;\;where\;n=1,2,3\cdots\;\;\;\;10$

Similarly, the recurrence relations for odd-labelled coefficients can be expressed as

$C_{2n+1}=-\[2\epsilon-3-2(2n-2)\]\frac{(2n-1)!}{(2n+1)!}C_{2n-1}\;\;\;where\;n=1,2,3\cdots\;\;\;\;11$

Rewriting $\psi(y)$ as a sum of two power series, one containing terms with even-labelled coefficients and the other with odd-labelled coefficients,

$\psi(y)=\biggr\{C_0+\sum_{l=1}^{\infty}\biggr\[-\[2\epsilon-1-2(2l-2)\]\frac{C_{2l-2}}{\frac{(2l)!}{(2l-2)!}}\biggr\]y^{2l}\biggr\}e^{-\frac{y^2}{2}}+\\\biggr\{C_1y+\sum_{l=1}^{\infty}\biggr\[-\[2\epsilon-3-2(2l-2)\]\frac{C_{2l-1}}{\frac{(2l+1)!}{(2l-1)!}}\biggr\]y^{2l+1}\biggr\}e^{-\frac{y^2}{2}}\;\;\;\;\;\;\;\;12$

According to L’Hopital’s rule, $\psi(y)$ appears to be a possible solution to eq7 because each of the terms in eq12 (simplified to $Cy^n/e^{\frac{y^n}{2}}$ ) approaches zero for large $y$ :

$\lim\limits_{y\rightarrow\pm\infty}\frac{Cy^n}{e^{\frac{y^2}{2}}}=\lim\limits_{y\rightarrow\pm\infty}\frac{nCy^{n-1}}{e^{\frac{y^2}{2}}}=\cdots=\lim\limits_{y\rightarrow\pm\infty}\frac{k}{e^{\frac{y^2}{2}}}=0$

where $k$ is a constant.

However, this does not guarantee that the series as a whole also converges because the sum of an infinite number of infinitesimal terms can still be infinite, as is the case for a harmonic series. To see how the two infinite series behave for large $y$ , we carry out the ratio test as follows:

where we have set $n=2l$ and $n=2l+1$ in eq9 for the even series and odd series respectively.

By comparison, the ratio test for the power series expansion of $e^{y^2}=\sum_{l=0}^{\infty}\frac{y^{2l}}{l!}$ is also $\frac{1}{l}$ . Therefore, both infinite series behave the same as $e^{y^2}$ and diverge for large $y$ . To ensure that $\psi(y)$ is square-integrable, we need to truncate either one of the series after some finite terms $l$ and let all the coefficients of the other series be zero.

Question

Can we instead truncate both series of eq12 after some $l$ ?

Answer

Since the value of $l$ is arbitrary, the sum of two truncated series, each with finite terms, may still have an infinite number of terms. The only way to guarantee that $u(y)$ has finite terms is to truncate one of the series and let $C_0$ , if the odd series is truncated, or $C_1$ , if the even series is truncated, be zero.

To truncate either series, we let the numerator of eq9 be zero so that every successive term in the selected series is zero as well. This implies that

$\epsilon=n+\frac{1}{2}\;\;\;\;\;\;\;\;13$

Let’s rewrite eq12 as

$\psi(y)=\biggr\{\begin{matrix}\biggr\{C_0+\sum_{l=1}^{m}\biggr\[-\[2\epsilon-1-2(2l-2)\]\frac{C_{2l-2}}{\frac{(2l)!}{(2l-2)!}}\biggr\]y^{2l}\biggr\}e^{-\frac{y^2}{2}}&even\;series\;l=1,2,\cdots\\\biggr\{C_1y+\sum_{l=1}^{m}\biggr\[-\[2\epsilon-3-2(2l-2)\]\frac{C_{2l-1}}{\frac{(2l+1)!}{(2l-1)!}}\biggr\]y^{2l+1}\biggr\}e^{-\frac{y^2}{2}}&odd\;series\;l=1,2,\cdots\end{matrix}\;\;\;\;14$

Since eq14 is the result of substituting $\psi(y)=u(y)e^{-\frac{y^2}{2}}$ in eq6, it is a solution to eq6.

Question

Show that $\psi_4(y)=C_0\biggr\[1-\frac{2n}{2!}y^2+\frac{2^2n(n-2)}{4!}y^4\biggr\]e^{-\frac{y^2}{2}}$ .

Answer

We expand the even series in eq14 as follows:

$\psi_4(y)=\biggr\[C_0-(2\epsilon-1)\frac{C_0}{2!}y^2-2(2\epsilon-1-4)\frac{C_2}{4!}y^4\biggr\]e^{-\frac{y^2}{2}}$

Substituting eq9, where $C_2=\frac{1-2\epsilon}{2}C_0$ , in the above equation, we have

$\psi_4(y)=\biggr\[C_0-(2\epsilon-1)\frac{C_0}{2!}y^2-\frac{(2\epsilon-1-4)(1-2\epsilon)}{4!}C_0y^4\biggr\]e^{-\frac{y^2}{2}}$

Substituting eq13 in the above equation gives the required expression.

Re-expanding the even series of eq14 using eq9 and eq13, we have

$\boldsymbol{\mathit{n}}$	$\boldsymbol{\mathit{\psi_n(y)}}$
$0$	$C_0e^{-\frac{y^2}{2}}$
$2$	$C_0\biggr\[1-\frac{2n}{2!}y^2\biggr\]e^{-\frac{y^2}{2}}$
$4$	$C_0\biggr\[1-\frac{2n}{2!}y^2+\frac{2^2n(n-2)}{4!}y^4\biggr\]e^{-\frac{y^2}{2}}$
$6$	$C_0\biggr\[1-\frac{2n}{2!}y^2+\frac{2^2n(n-2)}{4!}y^4-\frac{2^3n(n-2)(n-4)}{6!}y^6\biggr\]e^{-\frac{y^2}{2}}$

Comparing the coefficients of each even series in the above table, we have

$\psi_n(y)=C_0\biggr\[1-\frac{2n}{2!}y^2+\frac{2^2n(n-2)}{4!}y^4+\cdots+\frac{2^n$\frac{n}{2}$!}{(-1)^{\frac{n}{2}}n!}y^n\biggr\]e^{-\frac{y^2}{2}}\;\;\;\;\;\;\;\;15a$

where $n=0,2,4,\cdots$ .

Similarly, the re-expansion of the odd series of eq14 using eq9 and eq13 gives

$\boldsymbol{\mathit{n}}$	$\boldsymbol{\mathit{\psi_n(y)}}$
$1$	$C_1ye^{-\frac{y^2}{2}}$
$3$	$C_1\biggr\[y-\frac{2(n-1)}{3!}y^3\biggr\]e^{-\frac{y^2}{2}}$
$5$	$C_1\biggr\[y-\frac{2(n-1)}{3!}y^3+\frac{2^2(n-1)(n-3)}{5!}y^5\biggr\]e^{-\frac{y^2}{2}}$
$7$	$C_1\biggr\[y-\frac{2(n-1)}{3!}y^3+\frac{2^2(n-1)(n-3)}{5!}y^5-\frac{2^3(n-1)(n-3)(n-5)}{7!}y^7\biggr\]e^{-\frac{y^2}{2}}$

Comparing the coefficients of each odd series in the above table, we have

$\psi_n(y)=C_1\biggr\[y-\frac{2(n-1)}{3!}y^3+\frac{2^2(n-1)(n-3)}{5!}y^5+\cdots+\frac{2^n$\frac{n-1}{2}$!}{(-1)^{\frac{n-1}{2}}2(n!)}y^n\biggr\]e^{-\frac{y^2}{2}}\;\;\;\;\;\;\;\;15b$

where $n=1,3,5,\cdots$ .

The recurrence relation of eq9 that led to eq14 only defines the value of $C_n$ in terms of $C_{n+2}$ . This implies that there are many solution sets depending on the value of $C_{n+2}$ . The convention is to set the leading coefficients (i.e. the coefficients of $y^n$ ) of eq15a and eq15b, which are independent of each other, as $2^n$ . Therefore,

$C_0\frac{2^n$\frac{n}{2}$!}{(-1)^{\frac{n}{2}}n!}=2^n\;\;or\;\;C_0=(-1)^{\frac{n}{2}}\frac{n!}{(\frac{n}{2})!}\;\;\;\;\;\;\;\;16$

and

$C_1\frac{2^n$\frac{n-1}{2}$!}{(-1)^{\frac{n-1}{2}}2(n!)}=2^n\;\;or\;\;C_1=(-1)^{\frac{n-1}{2}}\frac{2(n!)}{(\frac{n-1}{2})!}\;\;\;\;\;\;\;\;17$

Substituting eq16 and eq17 in eq14, we have $\psi(y)=H_n(y)e^{-\frac{y^2}{2}}$ , where $H_n(y)$ are the Hermite polynomials:

$H_n(y)=\biggr\{\begin{matrix}\biggr\{(-1)^{\frac{n}{2}}\frac{n!}{(\frac{n}{2})!}+\sum_{l=1}^{m}\biggr\[-\[2\epsilon-1-2(2l-2)\]\frac{C_{2l-2}}{\frac{(2l)!}{(2l-2)!}}\biggr\]y^{2l}\biggr\}e^{-\frac{y^2}{2}}&even\;series\;l=1,2,\cdots\\\biggr\{(-1)^{\frac{n-1}{2}}\frac{2(n!)}{(\frac{n-1}{2})!}y+\sum_{l=1}^{m}\biggr\[-\[2\epsilon-3-2(2l-2)\]\frac{C_{2l-1}}{\frac{(2l+1)!}{(2l-1)!}}\biggr\]y^{2l+1}\biggr\}e^{-\frac{y^2}{2}}&odd\;series\;l=1,2,\cdots\end{matrix}\;\;\;\;18$

The first few Hermite polynomials, un-normalised eigenfunctions of eq6 and their corresponding eigenvalues are

When $y=0$ , we can express the Hermite polynomials for odd $n$ as

$H_{2n+1}(0)=0\;\;\;n=0,1,2,\cdots\;\;\;\;\;\;\;\;19$

Similarly, when $y=0$ , we can express the Hermite polynomials for even $n$ as

$H_{2n}(0)=\frac{(-1)^n(2n)!}{n!}\;\;\;n=0,1,2,\cdots\;\;\;\;\;\;\;\;20$

Eq19 and eq20 are useful in proving the generating function for Hermite polynomials.

The table above also shows that the energy states $E_n$ of a quantum-mechanical harmonic oscillator are quantised:

$E_n=\epsilon\hbar\omega=\biggr$n+\frac{1}{2}\biggr$\hbar\omega\;\;\;n=0,1,2,\cdots\;\;\;\;\;21$

with the ground state $E_0=\frac{1}{2}\hbar\omega$ being non-zero. This energy is called the zero-point energy of the harmonic oscillator.

Question

Using the uncertainty principle, explain why the ground state of a quantum harmonic oscillator is non-zero.

Answer

If $E_0=0$ , then both the kinetic energy $E_k=\frac{p^2}{2m}$ and the potential energy $V$ of the oscillator are zero. $E_k=0$ implies that the momentum $p$ is zero and $\Delta p=0$ . $V=0$ means that the atom is at the equilibrium position, where $\Delta x=0$ . This would violate the uncertainty principle.

Since $H_n(y)$ is a solution to eq8, we can rewrite eq8 as

$\frac{d^2H_n(y)}{dy^2}-2y\frac{dH_n(y)}{dy}+(2\epsilon-1)H_n(y)=0\;\;\;\;\;\;\;\;22$

To complete the prove that $\psi_n(y)=H_n(y)e^{-\frac{y^2}{2}}$ is a solution to eq6, we will derive the normalisation constant $N_n$ of $\psi_n(y)=N_nH_n(y)e^{-\frac{y^2}{2}}$ and prove that the wavefunctions are orthogonal to one another in the next few articles.

Question

Do we have to change the variable $y$ back to $x$ ?

Answer

The Hermite polynomials are usually expressed in terms of $y$ because $H_n\biggr$\sqrt{\frac{m\omega}{\hbar}}x\biggr$$ looks more complicated:

Hermite polynomials

$H_0\biggr$\sqrt{\frac{m\omega}{\hbar}}x\biggr$=1$

$H_1\biggr$\sqrt{\frac{m\omega}{\hbar}}x\biggr$=2\sqrt{\frac{m\omega}{\hbar}}x$

$H_2\biggr$\sqrt{\frac{m\omega}{\hbar}}x\biggr$=4\biggr$\sqrt{\frac{m\omega}{\hbar}}x\biggr$^2-2$

$\vdots$
However, the normalisation constant is defined as an integral with respect to $x$ because $x$ is the variable representing position in the one-dimensional space that the oscillator is moving in.

The quantum harmonic oscillator

Question

Answer

Question

Answer

Question

Answer

Question

Answer

Question

Answer

Question

Answer

Next article: Orthogonality of the wavefunctions of the quantum harmonic oscillator

Previous article: The harmonic oscillator

Content page of vibrational spectroscopy

Content page of advanced chemistry

Main content page

Leave a Reply Cancel reply