What is the formula describing the buffer capacity of water?
Consider a solution containing water and a strong base with the following equilibria:
With reference to the above equilibria, the sum of the number of moles of H+ and Na+ must equal to that of OH– (see this article for details). As the volume of the solution is common to all ions,
Substituting cb = [Na+] and Kw = [H+][OH–] in eq3 yields
Substituting eq1 and pH = –log[H+] in eq4 gives
To determine the maximum or minimum buffer capacity of water, we take the derivative of eq5 with respect to [H+] to give:
Letting yields
Substituting Kw = [H+][OH–] in the above equation results in
This means that a stationary point for the function in eq5 occurs when [OH–] = [H+] , i.e. at pH = 7. Let’s investigate the nature of this stationary point by differentiating eq6 again.
Substituting Kw = [H+][OH–] in the above equation, and noting that [OH–] = [H+], gives
If we repeat the above steps and consider the case of the addition of a strong acid to water, we end up with the same conclusion. Hence, water has minimum buffer capacity at pH = 7.
The buffer capacity of water over the entire range of pH can be found by substituting [H+] = 10-pH in eq5 to give
The plot of the above equation with βw as the vertical axis and pH as the horizontal axis is shown in the diagram below.
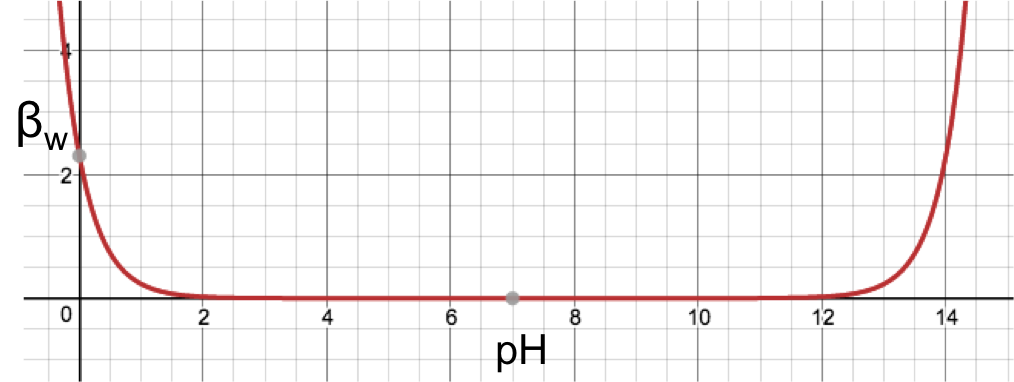
From the graph, the buffering capacity of water increases infinitesimally from a minimum of 0 at pH 7, to 0.023 at pH 2 on the left and pH 12 on the right, and then drastically to 2.303 at pH 0 on the left and pH 14 on the right. This means that water is only relatively effective as a buffer at extreme pH levels.
