Eigenvalues of quantum orbital angular momentum operators

As mentioned in an earlier article, if $\small \left [ \hat{L}^{2},\hat{L}_z \right ]=0$ , a common complete set of eigenfunctions can be selected for the two operators. Let $\small Y$ be the common set of normalised eigenfunctions with eigenvalues $\small b$ and $\small c$ for $\small \hat{L}^{2}$ and $\small \hat{L}_z$ respectively.

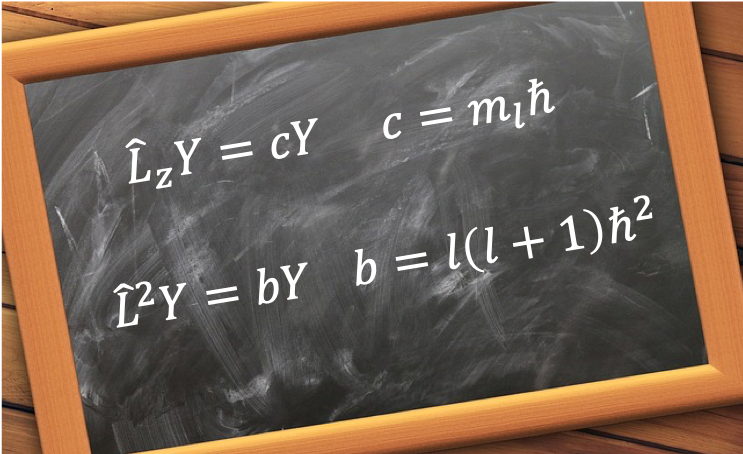
From eq75,

$\small \hat{L}^{2}-\hat{L}_z^{\; 2} =\hat{L}_x^{\; 2}+\hat{L}_y^{\; 2}$

Multiplying the above equation by $\small Y$ on the right, $\small Y^{*}$ on the left and integrating over all space, we have

$\small \langle L^{2}\rangle-\langle L_z^{\; 2}\rangle=\langle L_x^{\; 2}\rangle+\langle L_y^{\; 2}\rangle$

$\small b-c^{2}=\langle L_x^{\; 2}\rangle+\langle L_y^{\; 2}\rangle$

Even though $\small Y$ is not an eigenfunction of $\small \hat{L}_x^{\; 2}$ , we can still find the expectation value of $\small \hat{L}_x^{\; 2}$ , which is

$\small \langle L_x^{\; 2}\rangle=\int Y^{*}\hat{L}_x(\hat{L}_xY)d\tau=\int (\hat{L}_xY)(\hat{L}_xY)^{*}d\tau=\int \vert \hat{L}_xY\vert^{2}d\tau\geq 0$

Note that we have used the fact that $\small \hat{L}_x$ is Hermitian for the 2^nd equality (see eq37). Similarly, $\small \langle L_y^{\; 2}\rangle\geq 0$ . So,

$\small b-c^{2}\geq 0\; \; \; \; \; or\; \; \; \; \; -\sqrt{b}\leq c\leq \sqrt{b}$

Therefore, $\small c$ has an upper bound and a lower bound. Since the eigenvalues of $\small \hat{L}_z$ has an upper bound and a lower bound, from eq113 and eq114 we have

$\small \hat{L}_zY_{max}=c_{max}Y_{max}\; \; \; \; \; \; \; \; 122$

$\small \hat{L}_zY_{min}=c_{min}Y_{min}\; \; \; \; \; \; \; \; 123$

where $\small Y_{max}=\hat{L}_+^{\; k_{max}}Y$ , $\small c_{max}=c+k_{max}\hbar$ , $\small Y_{min}=\hat{L}_-^{\; k_{min}}Y$ and $\small c_{min}=c-k_{min}\hbar$ .

If we operate on eq122 with $\small \hat{L}_+$ , we supposedly have

$\small \hat{L}_z(\hat{L}_+Y_{max})=(c_{max}+\hbar)(\hat{L}_+Y_{max})$

However, the above equation contradicts the upper bound eigenvalue of $\small \hat{L}_z$ of $\small c_{max}$ . This implies that we must truncate the ladder beyond eq122. Since $\small c_{max}+\hbar\neq 0$ , we must have

$\small \hat{L}_+Y_{max}=0\; \; \; \; \; \; \; \; 124$

Using the same argument when operating on eq123 with $\small \hat{L}_-$ , we have

$\small \hat{L}_-Y_{min}=0 \; \; \; \; \; \; \; \; 125$

If we further operate on eq124 with $\small \hat{L}_-$ , we have

$\small \hat{L}_-\hat{L}_+Y_{max}=0$

Substitute eq116 in the above equation,

$\small (\hat{L}^{2}-\hat{L}_z^{\; 2}-\hbar\hat{L}_z)Y_{max}=0$

Using eq119 where $\small k=k_{max}$ , and eq122,

$\small (b-c_{max}^{\; \; \; \; \; \; 2}-\hbar c_{max})Y_{max}=0$

Since $\small Y_{max}\neq 0$

$\small b-c_{max}^{\; \; \; \; \; \; 2}-\hbar c_{max}=0 \; \; \; \; \; \; \; \; 126$

Similarly, operating on eq125 with $\small \hat{L}_+$ and using eq115, eq119 and eq123, we have

$\small b-c_{min}^{\; \; \; \; \; \; 2}+\hbar c_{min}=0 \; \; \; \; \; \; \; \; 127$

Subtracting eq127 from eq126, we have $\small (c_{max}+c_{min})(c_{min}-c_{max}-\hbar)=0$ . Since $\small c_{max}> c_{min}$ and $\small (c_{min}-c_{max}-\hbar)< 0$ , we have $\small c_{max}+c_{min}=0$ or

$\small c_{max}=-c_{min}\; \; \; \; \; \; \; \; 128$

As we know from eq122 and eq123, the value of $\small c_{max}-c_{min}$ of a particular system is dependent on the number of consecutive operations on $\small \hat{L}_zY$ by $\small \hat{L}_+$ or $\small \hat{L}_-$ , with each operation raising or lowering the eigenvalue of $\small \hat{L}_z$ by $\small \hbar$ . Therefore,

$\small c_{max}-c_{min}=0,\hbar,2\hbar,\cdots=2l\hbar\; \; \; \; \; \; \; \; 129$

where $\small l=0,\frac{1}{2},1,\frac{3}{2},\cdots$

Substituting eq128 in eq129, we have $\small c_{max}=l\hbar$ and $\small c_{min}=-l\hbar$ . Therefore, the eigenvalues of $\small \hat{L}_z$ are

$\small c=-l\hbar,-l\hbar+\hbar,-l\hbar+2\hbar,\cdots,l\hbar=-l\hbar,(-l+1)\hbar,(-l+2)\hbar,\cdots,l\hbar$

$\small c=m_l\hbar\; \; \; \; \; \; \; \; 130$

where $\small m_l=-l,-l+1,-l+2,\cdots,l$ .

Substituting $\small c_{min}=-l\hbar$ in eq127,

$\small b=l(l+1)\hbar^{2}\; \; \; \; \; \; \; \; 131$

where $\small l=0,\frac{1}{2},1,\frac{3}{2},\cdots$ .

As mentioned in the previous article, the raising and lowering operators also apply to the spin angular momentum $\small \boldsymbol{\mathit{S}}$ and the total angular momentum $\small \boldsymbol{\mathit{J}}$ . We would therefore expect the eigenvalues of $\small \hat{S}^{2}$ and $\small \hat{S}_z$ to be $\small s(s+1)\hbar^{2}$ and $\small m_s\hbar$ respectively, and the eigenvalues of $\small \hat{J}^{2}$ and $\small \hat{J}_z$ to be $\small j(j+1)\hbar^{2}$ and $\small m_j\hbar$ respectively. However, the quantum numbers $\small l$ and $\small m_l$ for the orbital angular momentum $\small \boldsymbol{\mathit{L}}$ , but not the quantum numbers for $\small \boldsymbol{\mathit{S}}$ and $\small \boldsymbol{\mathit{J}}$ , are restricted to integers. Therefore,

$\small \boldsymbol{\mathit{L}}$	$\small m_l\in \mathbb{Z}$	$\small l\in \mathbb{Z}$
$\small \boldsymbol{\mathit{S}}$	$\small m_s=-s,-s+1,-s+2,\cdots,s$	$\small s=0,\frac{1}{2},1,\frac{3}{2},\cdots$
$\small \boldsymbol{\mathit{J}}$	$\small m_j=-j,-j+1,-j+2,\cdots,j$	$\small j=0,\frac{1}{2},1,\frac{3}{2},\cdots$

Question

Why are the quantum numbers for restricted to integers?

Answer

The eigenvalue equation for $\small \hat{L}_z$ (see eq95) is:

$\small \frac{\hbar}{i}\frac{\partial}{\partial\phi}\psi=m_l\hbar\psi$

where $\small \psi=Ae^{im_l\phi}$ .

Since $\small \psi$ must be single-valued,

$\small Ae^{im_l\phi}=Ae^{im_l(\phi+2\pi)}$

$\small e^{im_l2\pi}=1$

$\small cosm_l2\pi + isinm_l2\pi=1$

The solution to the above equation is $\small m_l\in \mathbb{Z}$ . Furthermore, $\small l$ is also an integer because $\small m_l=-l,-l+1,-l+2,\cdots,l$ .

We would arrive at the same results (eq130 and eq131) if we have chosen $\small \hat{L}_x$ or $\small \hat{L}_y$ instead of $\small \hat{L}_z$ . The significance of eq130 and eq131 is that we can simultaneously assign eigenvalues of $\small \hat{L}^{2}$ and $\small \hat{L}_z$ (or $\small \hat{L}^{2}$ and $\small \hat{L}_x$ or $\small \hat{L}^{2}$ and $\small \hat{L}_y$ ) if the 2 operators commute. This, together with the fact that any pair of component angular momentum operators does not commute, implies that we cannot simultaneously specify eigenvalues of $\small \hat{L}^{2}$ and more than one component angular momentum operators.