Diprotic acid versus monoprotic strong base

What is the formula of the titration curve of a diprotic acid versus a monoprotic strong base?

Consider a solution containing water, a diprotic acid of concentration C_a and a strong base of concentration C_b with the following equilibria:

With reference to charge balance of the above equilibria, the sum of the number of moles of cations H⁺ and B⁺ must equal to that of anions HA^–, A^2- and OH^–. As the volume is of the solution is common to all ions,

Read this article to understand how to arrive at eq15.

With reference to eq13 and eq14, at any point of the titration, the sum of the number of moles of H₂A, HA^– and A^2– must equal to the number of moles of H₂A if it were undissociated. As the change in volume of the solution is common to all ions,

where V_a and V_b are the volume of diprotic acid in the solution and the volume of strong monoprotic base in the solution respectively. The equilibrium constant equations of eq13 and that of eq14 are:

Substitute eq16b in eq16a

Substitute eq16b and eq17 in eq16 and rearranging,

Substitute eq17a in eq16b

Substitute eq7, eq17a, eq18, K_w = [H⁺][OH^–] and [H⁺] = 10^-pH in eq15,

Eq19 is the complete pH titration curve for a diprotic acid (either strong or weak) versus monoprotic strong base system. We can input it in a mathematical software to generate a curve of pH against V_b. For example, if we titrate 10 cm³ of 0.200 M of H₂SO₄ (K_a1 = 1000, K_a2 = 1.02 x 10^-2) with 0.100 M of NaOH, we have the following:

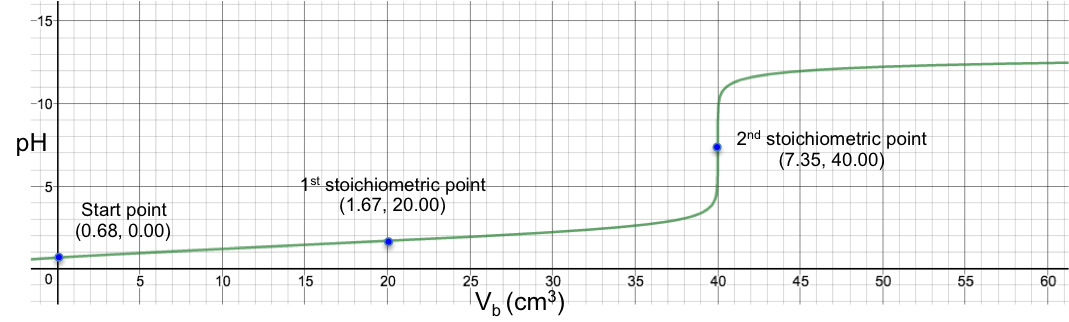
To understand the change in pH near the 1^st stoichiometric point versus the change in V_b, we assume that one drop of base is about 0.05 cm³ and substitute 19.95 cm³, 20.00 cm³ and 20.05 cm³ into eq19. The respective pH just before and just after the 1^st stoichiometric point (SP) are:

	One drop before SP	SP	One drop after SP
Volume, cm3	19.95	20.00	20.05
pH	1.665	1.668	1.670

The data shows that two drops of base cause a change of only 0.005 in pH before and after the 1^st stoichiometric point, which means we cannot monitor it during the titration process. The 1^st stoichiometric point is not discernable as the 1^st dissociation constant is a very large value, i.e. the first ionisation is complete. As for the 2^nd stoichiometric point,

	One drop before SP	SP	One drop after SP
Volume, cm3	39.95	40.00	40.05
pH	4.69	7.35	10.00

The data shows that just two drops of titrant cause a relatively big change of 5.31 in pH before and after the 2^nd stoichiometric point. We can therefore use many different indicators that work within the range of the change in pH at the 2^nd stoichiometric point to monitor the titration.

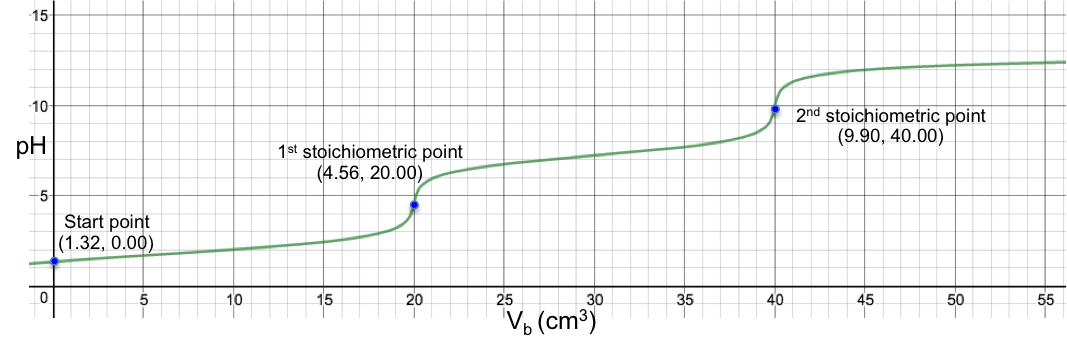
Another example is the titration of 10 cm³ of 0.200 M of H₂CO₃ (K_a1 = 4.5 x 10^-7, K_a2 = 4.8 x 10^-11) with 0.100M of NaOH. Using eq19, we have:

The changes of pH at both stoichiometric points are not sharp, with the 2^nd one almost non-discernable. Finally, for the titration of 10 cm³ of 0.200 M of H₂SO₃ (K_a1 = 1.5 x 10^-2, K_a2 = 6.2 x 10^-8) with 0.100M of NaOH, we have:

 

next article: Triprotic acid versus monoprotic strong base

Previous article: Comparison of strong acid versus strong base curve and weak acid versus strong base curve

Content page of complete ph titration curve

Content page of advanced chemistry

Main content page