The one-particle, time-dependent Schrodinger equation is a partial differential equation whose solutions are the one-particle, time-dependent wave functions of quantum-mechanical systems.
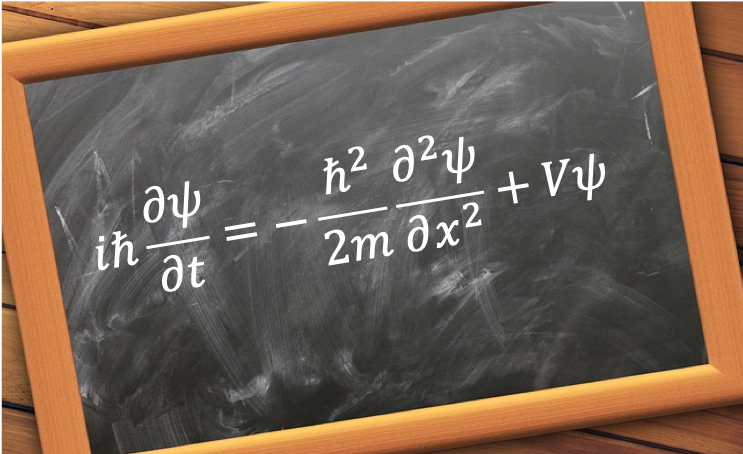
Even though the equation is widely regarded as a postulate, we can derive it using a general travelling wave equation . Since cosine is an even function,
, which in the complex square-integrable form is:
. Since
, we have
. Substituting Planck’s relation and de Broglie’s hypothesis in the wave equation, which is a mathematical description of the properties of a quantum-mechanical particle, we have
, where
.
The total energy of the particle is: , and so
To develop an expression for , we find the partial derivative of
with respect to
:
As for , we find the the 2nd-order partial derivative of
with respect to
:
Substituting eq55 and eq56 in eq54, we have
Eq57 is the one-particle, one-dimensional, time-dependent Schrodinger equation, which has the general solution .

Question
Show that is a solution to eq57.
Answer
For LHS of eq57
So,