Category: Advanced Chemistry

Entropy is a measure of the amount of disorder or the number of possible microscopic arrangements in a system, and the arrow of time is the one-way direction in which time flows. But how are the two concepts related? To unravel this, we must first understand what entropy really is.

Have you ever wondered why a tablespoon of salt dissolves spontaneously in water, or why certain foods turn rancid over time? These everyday occurrences may seem unrelated, but they are all tied to a powerful concept in physics and chemistry: entropy. Though the word may conjure images of complex equations or scientific jargon, entropy is something we all experience daily. It’s the quiet force behind why hot coffee cools, why ice melts at room temperature, and even why dust spreads everywhere and instead of obediently accumulating in the dustpan.

But what exactly is entropy? Many spontaneous processes that occur in our homes involve a dispersal of energy. When salt dissolves in water, the ordered solid lattice structure of sodium chloride breaks apart into freely moving ions, which can occupy many more positions within the liquid (see diagram below). Similarly, when butter turns rancid due to oxidation, gases and volatile organic compounds (such as aldehydes, ketones and short-chain fatty acids) are released, dispersing energy and matter into the surrounding air.

Entropy, denoted by , is a measure of such energy dispersal at a specific temperature. Because spontaneous processes tend towards greater disorganisation, entropy is often loosely described as a measure of disorder or randomness in a system. In fact, the Second Law of Thermodynamics, which states that the entropy of an isolated system increases over time, is rooted in countless everyday observations like these.

On closer examination, the second law is not an absolute rule but a statistical tendency that applies to systems with vast numbers of particles — atoms and molecules that move in countless ways. It describes the probabilistic tendency of these particles to evolve towards more disordered, more probable arrangements.

Consider the melting of ice at room temperature. The highly ordered crystalline structure of ice (low entropy) spontaneously transitions into liquid water (higher entropy) because there are far more ways for the molecules to arrange themselves in the fluid state than in a rigid crystal lattice. This transformation increases the total entropy of the system (ice plus water).

How then is entropy related to the arrow of time? On the macroscopic level, Newton’s equations are time-symmetric — they work just as well backward as forward. For example, if and are replaced by and in Newton’s second law , the same valid solution is obtained. But when we consider systems with enormous numbers of particles, like salt dissolving in water or ice melting, the laws of probability take over. The number of possible disordered (high-entropy) configurations vastly exceeds the number of ordered (low-entropy) ones. Thus, while nothing in the laws of physics forbids entropy from decreasing, it is astronomically unlikely. In other words, entropy increases not because it must, but because it is overwhelmingly probable that it will. This statistical bias gives rise to what physicist Arthur Eddington famously called the Arrow of Time. The arrow points in the direction of increasing entropy, defining the “forward” direction of time that we perceive in memory, causality and the evolution of the universe.

If the arrow of time points in the direction of increasing entropy, then it must have had a beginning — a moment when entropy was at its lowest. To understand the flow of time in the universe, we must look back to its earliest moments: the Big Bang.

At first glance, the Big Bang might not seem like a low-entropy event. After all, the early universe was extremely hot, dense, and filled with high-energy radiation — conditions we might intuitively associate with disorder. But entropy is not just about temperature; it also depends on how matter and energy are arranged. The early universe, though energetic, was remarkably smooth and uniform, with matter and radiation spread almost evenly in all directions. This evenness means it had very few possible configurations, and therefore, very low entropy.

The evidence for this comes from the cosmic microwave background (CMB), the faint afterglow of the Big Bang. Unlike radiation from stars or galaxies, the CMB is remarkably uniform in all directions. Observations of the CMB across the sky, interpreted using the Planck radiation law, reveal temperature variations of only about one part in 100,000. This extraordinary uniformity indicates that, in its infancy, the universe was astonishingly smooth and ordered. Had the universe begun in a random, high-entropy state, matter and radiation would have been far clumpier and more chaotic, and the arrow of time, the steady progression towards greater disorder, might never have emerged as we observe it.

As the universe expanded from the Big Bang, gravity began to play an increasingly important role in shaping entropy. Unlike most physical systems, gravity behaves counterintuitively with respect to order and disorder. In an ordinary gas, for example, molecules spread out to maximise entropy. But in a gravitational system, clumping actually increases entropy.

To see why, imagine a cloud of gas floating in space. If left alone, gravity will cause it to collapse and form a star or planet. The resulting structure seems more ordered, but in reality, the overall entropy has increased. That’s because the gravitational potential energy lost during collapse is converted into heat and radiation, which disperse into space. The final configuration, a hot star emitting light, represents a far greater number of microscopic possibilities than the original, uniform gas cloud.

This process explains how the universe could start with low entropy and still give rise to the complex, structured cosmos we see today — galaxies, stars, planets and eventually life. As gravity amplified tiny irregularities in the early universe, matter clumped together and entropy grew. The formation and evolution of stars, black holes and galaxies are all milestones on this cosmic journey towards higher entropy.

If entropy keeps increasing, what happens in the end? The answer depends on how the universe evolves, whether it will expand forever, halt and contract, or oscillate in cycles. These possibilities are described in four scenarios:

1. • Big Freeze (or heat death):

Observations over the past two decades, particularly of distant supernovae and the cosmic microwave background, reveal that the universe’s expansion is accelerating. In this case, the universe will grow ever colder and more diffuse. Over trillions of years, stars will burn out, stellar remnants will cool, and galaxies will fade into darkness. Eventually, the cosmos will approach a state of maximum entropy — a condition known as the heat death of the universe. At that point, the universe will be a thin, uniform haze of particles and radiation, with no free energy left to drive physical processes or sustain life. In such a state, the universe reaches thermodynamic equilibrium, and with no further increase in entropy possible, the arrow of time would effectively lose its direction.

1. • Big Crunch:

If gravity were strong enough, it could eventually halt the expansion of the universe and reverse it into a contraction, ending in what is known as a “Big Crunch.” In this scenario, galaxies and stars would merge, black holes would coalesce, and even atoms would be torn apart as the universe collapses into an increasingly dense and hot state. The gravitational collapse releases potential energy as heat and radiation, driving the total entropy of the universe ever higher. As densities and temperatures approach extreme values, known laws of physics break down, and space and time themselves may lose their classical meaning.

1. • Big Bounce:

The Big Bounce is a speculative but fascinating alternative to both the Big Freeze and Big Crunch scenarios. It proposes that the universe did not begin from a singular, one-time Big Bang, but rather from the collapse of a previous universe. In this picture, the cosmos undergoes a perpetual cycle of expansion and contraction — each “Big Crunch” giving rise to a new “Big Bang.” Instead of a final end, the universe continually renews itself through an endless series of bounces. This idea is based on the hypothesis that at extremely high densities, quantum effects could generate a repulsive force that halts the collapse before a singularity forms.

However, the Big Bounce faces a fundamental challenge from the Second Law of Thermodynamics. Entropy can only increase, so if each cycle carries forward the entropy produced in the previous one, the total disorder of the universe would accumulate from bounce to bounce. Over many iterations, the universe would grow larger and last longer with each cycle, trending towards a maximum entropy state, which leads to a heat-death-like equilibrium even within this cyclic framework. In other words, while a Big Bounce could prevent a singular end, it may not fully escape the thermodynamic arrow of time.

1. • Big Rip:

The Big Rip is one of the most dramatic and unsettling possibilities for the fate of the universe. In this model, the universe, fuelled by dark energy, keeps accelerating until it overcomes all other forces. First, distant galaxies would drift out of view as their light can no longer reach us. Then, the gravitational bonds holding galaxies together would fail. Stars and planets would be torn from their orbits, followed by the disintegration of solar systems and the destruction of individual stars. In the final moments, even atoms and subatomic particles would be ripped apart as the fabric of space itself expands faster than light can travel. The entire process would culminate in a singular event when spacetime and all known structures are shredded into oblivion.

Of all the scenarios, the Big Freeze is the only one consistent with current observations of accelerated expansion. In this case, time unquestionably continues, but it leads to a static, featureless epoch where there is no change and thus no discernible arrow of time.

Whatever the ultimate explanation, one fact remains: we live in a universe where entropy increases, where time moves inexorably forward, and where each passing moment marks a small but irreversible step in the great unfolding of cosmic history. The arrow of time, born in the furnace of the Big Bang, continues to guide the evolution of everything, from the orbits of galaxies to the beating of our own hearts.

 

Previous article: Statistical entropy

Content page of chemical thermodynamics

Content page of advanced chemistry

Main content page

An atomic clock is a highly precise timekeeping device that measures time based on the frequency of electromagnetic radiation emitted or absorbed during transitions between specific energy levels of atoms.

Traditional mechanical and even early electronic clocks are limited in accuracy because their timekeeping depends on macroscopic oscillations, such as pendulum swings or quartz vibrations, which are affected by temperature, friction, air pressure and other environmental factors. These variations cause conventional clocks to drift over time, making them unsuitable for applications that require extreme precision.

The idea of using atomic phenomena to measure time dates back to the 19th century. In his 1873 Treatise on Electricity and Magnetism, James Clerk Maxwell was among the first scientists to suggest that the oscillations of light waves could serve as a fundamental standard for time, laying the conceptual groundwork for the development of atomic clocks nearly a century later.

Building on Maxwell’s insight, modern atomic clocks achieve extraordinary precision by measuring the natural “ticks” of atoms rather than relying on macroscopic oscillations. One of the most widely used types is the caesium-133 atomic clock, which defines the second in the International System of Units (SI).

In the ground state, a caesium-133 atom has a single unpaired electron in its valence s-orbital, giving it the electronic configuration [Xe] 6s¹. For this electron, the orbital angular momentum is zero, and the spin angular momentum is ​, resulting in a total electronic angular momentum of and the term symbol . The nucleus, composed of protons and neutrons, has a collective nuclear spin of ​.

Both the electron and the nucleus generate magnetic fields associated with their respective magnetic dipole moments, and these fields interact with one another. This interaction, known as magnetic dipole coupling, causes the nuclear spin angular momentum to combine vectorially with the total electronic angular momentum (see diagram above), producing two total angular momentum states , where  and is the projection of onto the laboratory -axis. The small energy difference between these two states (see diagram below), known as the hyperfine splitting, forms the basis for the caesium atomic clock: by precisely measuring the frequency of radiation corresponding to transitions between these two states, the clock can maintain an exceptionally stable and accurate measure of time.

 

 

The main components of a caesium atomic clock include an atomiser, a magnetic state selector, a resonance chamber, a second magnetic selector, a detector and an electronics module (see diagram above). In the atomiser, an oven heats a small amount of metallic caesium, releasing atoms that pass through a narrow aperture to form a beam within a vacuum chamber. The oven temperature is carefully maintained at around 120°C to produce an adequate vapour pressure of caesium. At this temperature, the population ratio of atoms in the upper and lower hyperfine states follows the Boltzmann distribution:

Substituting , , and into the above equation gives:

Atoms in the lower hyperfine state are then selected and directed into the resonance chamber by a magnetic selector acting as a Stern-Gerlach device. Inside the chamber, the atoms are exposed to microwave radiation tuned near 9,192,631,770 Hz, corresponding to the transition between the two hyperfine levels. This frequency is generated by an electronic oscillator connected to both the resonance chamber and the detector in a feedback loop. When the microwave frequency exactly matches the transition, atoms in the lower state are driven to the upper state with maximum probability. After leaving the chamber, the beam passes through a second Stern–Gerlach device, which deflects unexcited atoms so that only those in the excited state reach the detector.

The detector measures the number of caesium atoms that arrive. Typically, this involves the atoms striking a hot surface, where they are ionised. The resulting ions or electrons are collected at an electrode, generating an electric current in the detector circuit that is proportional to the number of excited atoms reaching the detector. When the oscillator frequency is exactly equal to the transition frequency, the detector current reaches a maximum value, .

If temperature or other effects cause the oscillator frequency to drift from 9,192,631,770 Hz, the detector current decreases. A servo-control circuit detects this deviation and adjusts the oscillator frequency to restore the current to . This continuous feedback ensures that the oscillator remains precisely locked to the hyperfine transition frequency. The locked oscillator output is then sent to a counter circuit, which counts the oscillations and produces one output pulse each time exactly 9,192,631,770 cycles are completed — defining one second. These pulses drive a digital display, allowing the clock to show the precise time corresponding to the oscillations of the caesium atoms.

Modern caesium atomic clocks maintain accuracy to within a few billionths of a second per day and form the basis of Coordinated Universal Time (UTC), as well as global positioning and communication systems.

 

 

Previous article: f-orbital

Content page of quantum mechanics

Content page of advanced chemistry

Main content page

The energy–time uncertainty relation states that the more precisely a system’s energy is defined, the longer it takes for the system to undergo a significant change.

Mathematically, it is expressed as:

It can be derived from the general form of the uncertainty principle , where and are the observables corresponding to the Hermitian operators and , respectively. In this case, we let be a system’s energy , which is the observable associated with the Hamiltonian operator . Any uncertainty in therefore corresponds to a change in .

However, in non-relativistic quantum mechanics, time is a parameter, not a Hermitian operator. Hence, we cannot replace with a time operator . Instead, we begin with the time evolution of the expectation value of an operator that does not explicitly depend on time:

 

 

Differentiating eq27b with respect to time using the product rule gives:

The time evolution of the state is governed by the time-dependent Schrödinger equation . Taking its Hermitian conjugate yields . Substituting these two equations into eq27c results in:

 

 

Comparing eq27d with the general uncertainty principle gives:

where .

Since , eq27e becomes the energy-time uncertainty relation. Here, corresponds to the time scale for the system’s evolution, i.e. the time required for the expectation value of to change by one standard deviation .

An important example of this relation occurs in the excited state of a molecule, where corresponds to the lifetime of the excited state, and is the uncertainty in the transition energy between the excited and relaxed states. In other words, the shorter the lifetime of an unstable state, the larger the uncertainty in its transition energy. A large means the emitted photon’s energy is not a single, sharp value, but a range of values, leading to a broadened line in the spectrum.

The broadening of spectral lines can also be caused by molecular interactions. For example, collisions between atoms or molecules lead to shortened excited-state lifetimes by inducing transitions via a non-radiative pathway. When two particles approach closely enough to interact, their potential energy varies according to the internuclear distance, producing a perturbation that couples their internal energy levels. If part of the internal energy of particle A, which is in an excited state, is transferred to particle B during the collision, particle A undergoes collisional de-excitation, and the excess energy is converted into additional kinetic energy of the colliding pair rather than being emitted as a photon. This process effectively reduces the lifetime of the excited state, leading to spectral broadening.

Finally, since , eq27a is sometimes written less precisely as:

or

 

Next article: spectral decomposition of an operator

Previous article: The uncertainty principle (derivation)

Content page of quantum mechanics

Content page of advanced chemistry

Main content page

The Wigner D-matrix , where the total angular momentum  is a unitary matrix that represents all rotation symmetry operations corresponding to the irreducible representations of the SU(2) group.

The irreducible representations of SU(2), or special unitary group of degree 2, consists of unitary matrices with determinant 1. They describe angular momentum transformation for particles with both integer and half-integer values of . In the case where is an integer, the corresponding Wigner D-matrices also represent the irreducible representations of the SO(3) group, which is the group of all proper rotations in 3D space.

As shown in the previous article, the total rotation operator transforms a quantum state with total angular momentum projection along the molecular -axis into a linear combination of states with the projection along the lab -axis:

Since forms a complete orthogonal basis set for SO(3), the coefficients of , according to group theory, are the matrix elements of . Multiplying the above equation on the left by the bra gives the Wigner D-matrix elements:

Substituting eq115 into eq120 yields:

where is an eigenstate of by convention.

If , then (see this article for proof). So,  and eq121 becomes:

Since and is Hermitian, i.e. , we have . It follows that

where is the Wigner small-d matrix element.

The corresponding Wigner small-d matrix is a single-axis, single-angle rotation operator of the SO(3) group in the basis.

 

 

, other than being matrix elements of Wigner D-matrices, are also rotational wavefunctions. To explain why, we refer to the great orthogonality theorem for finite groups, given by:

where

• • refers to the matrix entry in -th row and -th column of the -th matrix of the -th irreducible representation.
  • is the order of the group, and is also the normalisation factor for the sum.
  • is the dimension of the irreducible representation.

The theorem can be extended to infinite groups like SO(3), with the normalised sum over group elements replaced by a normalised integral over all rotation angles :

where

• • , the total volume of the SO(3) manifold (intrinsic rotation space), is the normalisation factor for the integral, i.e. .
  • (see this article for further explanation).
  • is a specific set of values.

Eq124 reveals that the functions are complex and orthonormal. They are eigenfunctions of and (in units), where:

and

Since there are infinite SO(3) irreducible representations, are associated with all possible combinations of the three rotational quantum numbers and hence all possible eigenvalues. In other words, form a complete orthonormal set of wavefunctions for symmetric rotors.

 

Previous article: Rotation operator

Content page of rotational spectroscopy

Content page of advanced chemistry

Main content page

A rotation operator is a unitary operator that rotates quantum states by an angle about an axis in a Hilbert space.

Mathematically, it is given by:

where , the angular momentum operator, is the generator of rotations about axis .

To derive eq100, we begin by noting that the probability outcome of a quantum mechanical measurement is described by the Born interpretation:

where and are wavefunctions representing quantum states.

Since rotations are symmetry operations that do not change physical probabilities, we require:

Using the matrix identity (see property 13 of this article for proof), we have . Therefore, is unitary, where .

Let’s approximate as a power series of a small change in :

where and are first and second order rotation generator matrices respectively.

Since a rotation by zero angle must do nothing, , and eq101 (ignoring higher order terms) becomes

To preserve unitarity, . Expanding this equation and ignoring higher-order terms gives or . This implies that is not Hermitian, which contradicts the postulate that all physical observables in quantum mechanics are represented by Hermitian operators. It follows that eq102 must have the following form:

For a quantum mechanical operator to represent the same physical observable in the passively rotated frame, its expectation value must be the same whether calculated in the initial frame or with respect to the new frame:

Substituting into eq104 gives , which means that and are related by the similarity transformation or equivalently,

where because .

Substituting eq103 into eq105 yields:

where we have let the operator be the position operator .

Expanding eq106 and ignoring higher order terms results in , or equivalently,

where .

To determine the nature of , we refer to the active classical rotation of the vector by an infinitesimal angle about an arbitrary axis represented by the unit vector (see diagram above), with the infinitesimal change in given by:

where the cross product results in a vector with a magnitude proportional to and a direction tangential to the path of rotation.

Using the methodology of replacing classical variables with their quantum mechanical analogues to derive quantum mechanical expressions, eq108 becomes:

where we have added a minus sign because in eq108 is defined by an active rotation about the axis, while in eq107 describes a passive rotation about the axis.

 

 

Substituting into eq109 and expanding it gives:

Since , and , we have:

Substituting into 107 and setting to be the -axis for simplicity gives:

Comparing eq110 with eq111 yields:

Comparing these three commutation relations with the total angular commutation relations of , and , we find that . In other words, is the -th component of the total angular momentum operator, expressed in units of .

A finite rotation by an angle can be constructed by apply successive infinitesimal rotations, each by an angle . From eq103,

 

 

Therefore, eq112 becomes:

Eq113 is usually written as:

where the eigenvalues of and hence are expressed in units of

Since is a finite-dimensional linear operator acting on a finite-dimensional vector space, can be represented by an matrix in both group theory and quantum mechanical calculations. In general, the rotation operator in an Euler angle system, which is defined by three successive rotations by the angles , and , is given by:

where , and .

The application of eq115 involves the rotation operator transforming a quantum state with total angular momentum projection along the molecular axis into a linear combination of states with the projection along the lab -axis:

where , the entries of the Wigner D-matrix, are the coefficients of .

 

 

Next article: Wigner D-matrix

Previous article: Centrifugal distortion

Content page of rotational spectroscopy

Content page of advanced chemistry

Main content page

The SO(3) group, or special orthogonal group in three dimensions, is an infinite group of all 3D rotations.

Each element of the group corresponds to a rotation operator, characterised by a unit rotation axis and an angle . Since there are infinitely many possible rotation angles and axes, SO(3) contains infinitely many elements. These elements satisfy the properties of a group. For example, the binary operation of two rotation operators, each with a specific rotation angle about an axis, results in another rotation operator, demonstrating the closure property.

Consider the rotation of the spherical harmonics around the -axis. When the rotation operator acts on by an angle around the -axis, only the azimuthal angle is affected and each basis is transformed into . The transformation for all basis functions is summarised as:

 

 

In other words, , and according to the above Q&A,

where is the -component of the angular momentum operator, expressed in units.

Since an irreducible representation of a group is expressed by a set of matrices (or matrix-valued functions) that represent the group elements as linear operators on a vector space, an irreducible representation of the SO(3) group is –dimensional (see eq120), with the set forming a basis for the irreducible representation for a fixed .

 

 

The character of the rotation matrix is , which is a geometric series , where , and . This implies that

Using Euler’s formula of ,

The SO(3) group does not have a standard character table in the same way that finite point groups do. Its irreducible representations are labelled by a non-negative integer , which form a discrete set. However, the group elements and their associated characters are continuous functions that depend on the rotation angle.

Nevertheless, for illustrative purposes, we can express the relationships between group elements, their irreducible representations and corresponding characters in the following way:

where each can be further expanded as follows:

 

 

In general, a rotation operator of the SO(3) group is given by (see this article for derivation):

where , and are the Euler angles.

The question, then, is whether the table relating the general rotation group elements, their irreducible representations and corresponding characters will be the same as that of a single-axis, single-angle rotation operator such as ?

The character of an irreducible representation of SO(3) depends only on the total rotation angle, not on the specific rotation axis or the individual Euler angles. Since SO(3) is the infinite group of all possible 3D rotations, there exists a one-to-one correspondence in symmetry between three consecutive rotations described by and a single rotation described by . In other words, even though is expressed in terms of Euler angles, it represents the same group element as some , because we can always find a symmetry operation about an appropriate axis that produces the same group element and therefore the same character value. Hence,

Finally, the rotation of , the Wigner D-matrix elements, for fixed and fixed is given by:

This shows that, for fixed , the set forms a basis of the same -dimensional irreducible representation of SO(3) as the set . Since are eigenfunctions of the angular momentum operators ( and ), it follows that are also eigenfunctions of the corresponding operators and . In other words, are also rotational wavefunctions.

 

 

Previous article: Correlation diagram

Content page of group theory

Content page of advanced chemistry

Main content page

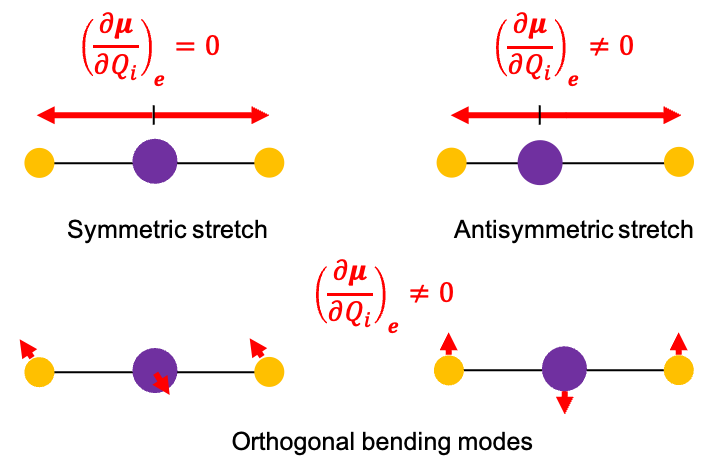
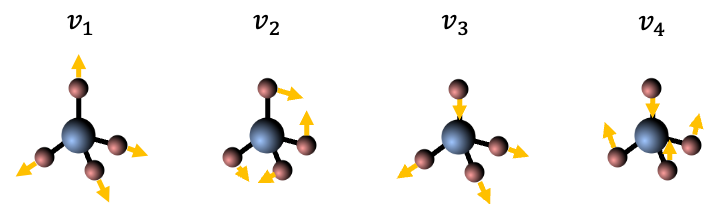
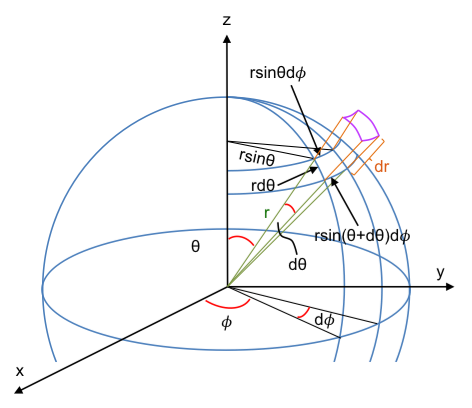
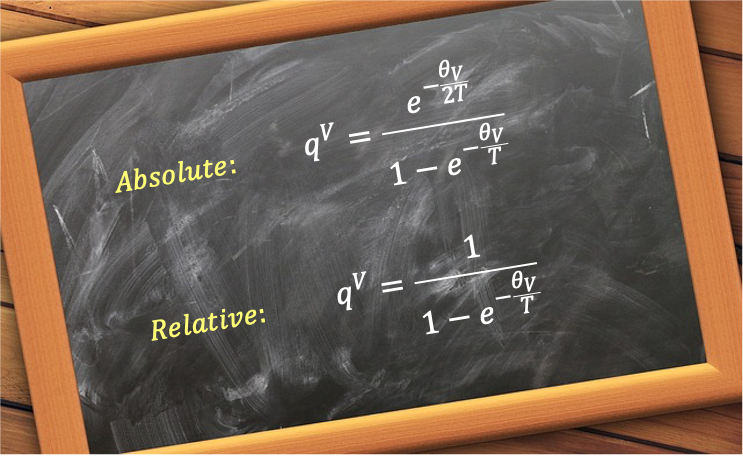
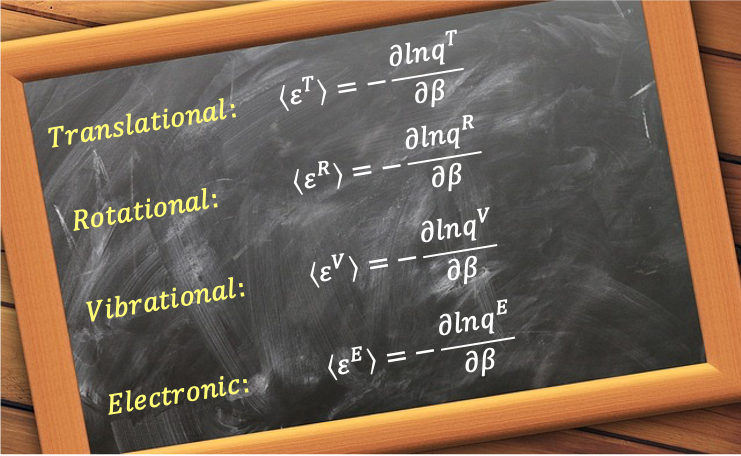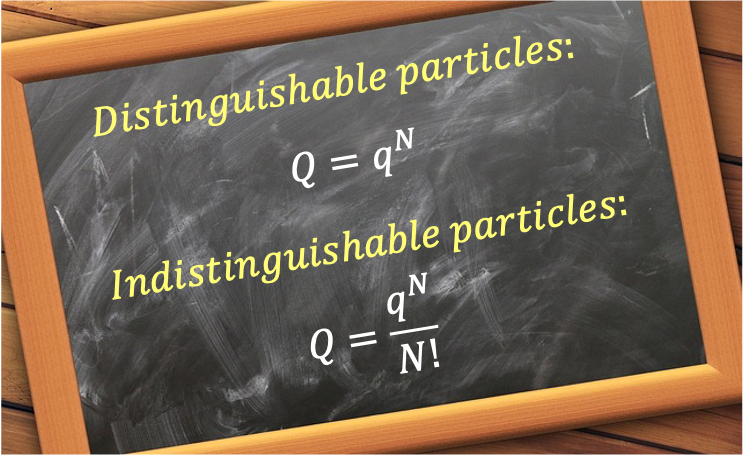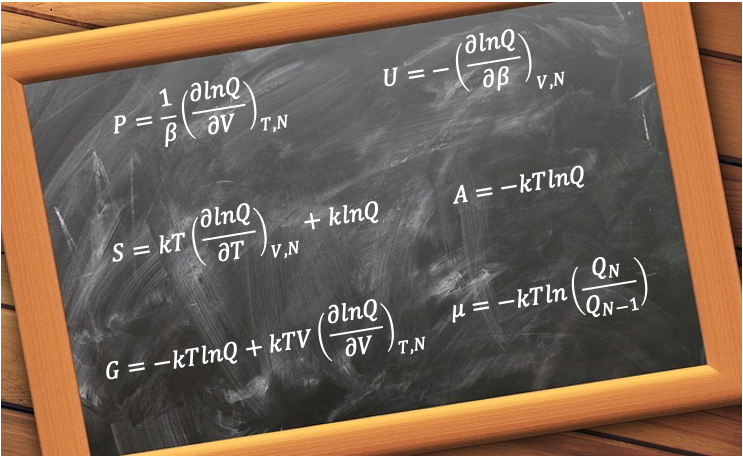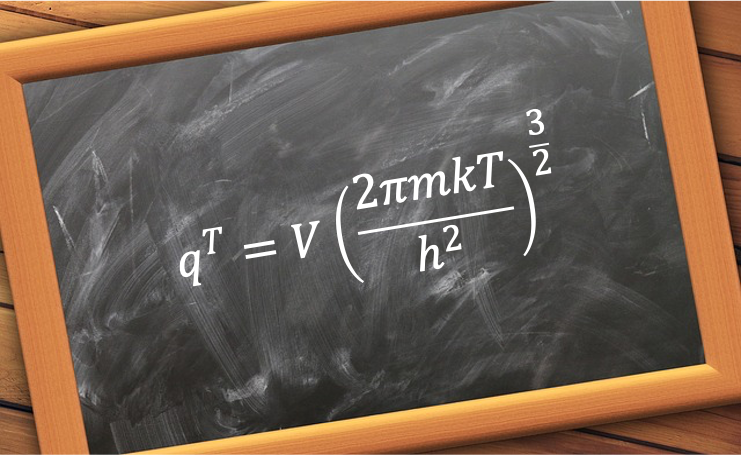The Coriolis effect is the apparent deflection of a moving object when observed from a rotating reference frame.

Consider a person holding a ball and standing on the edge of a large circular platform that is rotating anti-clockwise as viewed from above. At the twelve o’clock position, the person throws the ball directly towards the centre point O of the platform.

From the perspective of a stationary observer hovering above the platform (i.e. in an inertia frame of reference), the ball follows a straight-line path towards point X, slightly to the left of O. This trajectory occurs because, at the moment of release, the ball has two components of velocity:

• • A radial component directed towards O.
  • A tangential velocity due to the rotation of the platform at the point of release (in this case, towards the nine o’clock direction).

The resulting trajectory is the vector sum of these two components, which points towards X.

However, to the thrower, who is in the rotating frame of reference, the motion of the ball appears quite different. In this rotating frame, the ball seems to curve clockwise, as if being deflected to the right of its intended path. This illusion arises because, after release, the ball retains its original tangential speed, while the platform between the thrower and point O are rotating more slowly (closer to the center, where tangential speed is lower). In other words, the ball appears to be moving ahead of the rotating platform beneath it.

It follows that an apparent force, acting perpendicular to the direction of the ball’s motion, is influencing its path. This fictitious force, introduced to account for the apparent deflection experienced in rotating frames, is known as the Coriolis force.

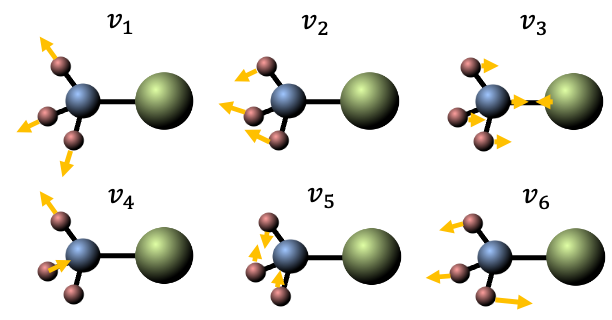
This phenomenon isn’t just a curiosity of rotating platforms. On the molecular level, the Coriolis force introduces a crucial vibration-rotation interaction (also known as Coriolis coupling) in the dynamics of a rotating molecule — an interaction that would otherwise be neglected in a first-order approximation, where vibration and rotation are treated as independent motions.

When a molecule rotates, its internal motion (vibration) occurs simultaneously within the rotating molecular frame. To an observer in this rotating frame, the atoms undergoing vibration appear to be deflected perpendicular to their vibrational velocity. The resulting Coriolis force, associated with this apparent deflection, acts to couple the molecule’s vibrational angular momentum with its overall rotational angular momentum.

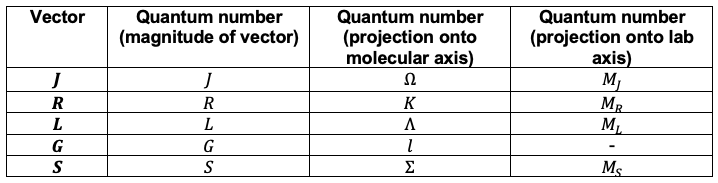
In quantum mechanics, this coupling appears as an additional term in the Hamiltonian that couples vibrational and rotational states:

where is proportional to the product of vibrational and rotational angular momentum operators.

Explicitly,

where

denotes the molecular principal axes ().
is the operator for the -component of the total angular momentum of the molecule (rotation).
is the operator for the -component of the vibrational angular momentum.

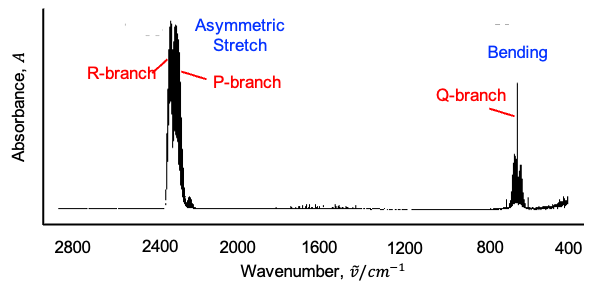
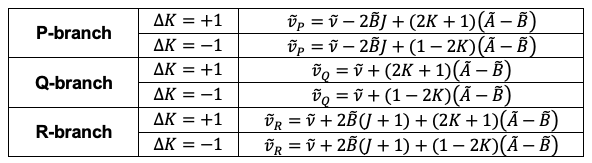
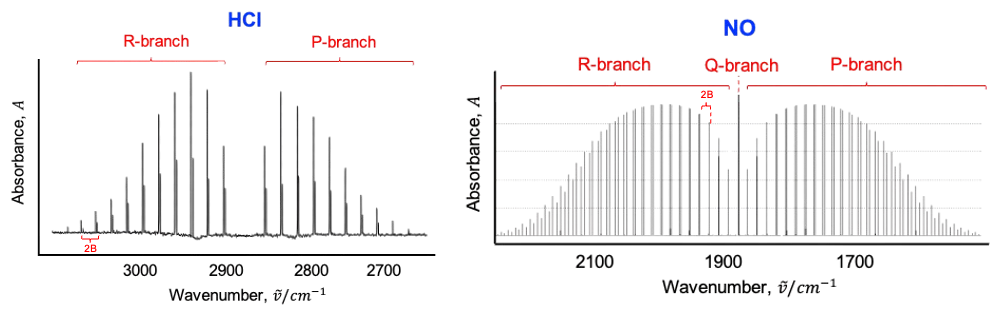
This leads to shifts in rotational energy levels depending on the vibrational state, as well as the splitting or mixing of rotational levels associated with degenerate vibrations. In the infrared spectrum, this manifests as an anomalous splitting of the P, Q and R branches.

On a much larger scale, the Coriolis effect plays a crucial role in shaping natural systems on Earth. Because Earth is a rotating sphere, this effect influences the motion of air masses, ocean currents and weather patterns. For instance, a cyclone’s spin results from two forces acting on the atmosphere simultaneously:

• • The pressure gradient force moves air from a high-pressure region to a low-pressure region.
  • The Coriolis effect deflects that moving air

A low-pressure system is essentially a partial vacuum that draws in air from all directions. Consider a low-pressure region in the Northern Hemisphere (the upper yellow region in the diagram below). To an observer in a rotating frame of reference (e.g. a satellite moving at the same angular velocity as Earth from west to east), winds moving from the equator towards the low-pressure region appear to be deflected to the right, while winds flowing towards the region from the north appear to be deflected to the left. The combined movements result in an anti-clockwise sprial. The same principle causes winds to sprial clockwise in the Southern Hemisphere.

In conclusion, the Coriolis effect demonstrates the profound influence of rotational motion across vastly different scales — from affecting molecular behaviour to shaping global weather systems. At the molecular level, the principle manifests as Coriolis coupling, where rotational motion interacts with vibrational motion, subtly altering energy levels and spectral properties. On Earth, it governs the deflection of winds and ocean currents, giving rise to the rotation of cyclones and other large-scale atmospheric patterns. Together, these phenomena highlight the unifying power of rotational dynamics in both macroscopic and microscopic systems.

 

Previous article: Vibration-rotation spectra of polyatomic molecules

Content page of rovibrational spectroscopy

Content page of advanced chemistry

Main content page

Vibration-rotation spectra arise from the simultaneous vibrational and rotational transitions of molecules, typically observed in the infrared (IR) region of the electromagnetic spectrum. These spectra provide detailed information about molecular structure, bond strength, and moment of inertia.

For a molecule to be IR active, it must have a permanent dipole or a dipole moment that changes over time. Since polyatomic molecules have vibrational modes, some which do not result in a change in the molecules’ dipole moments, only certain vibration-rotation transitions are IR active. The transition selection rules are given by eq7 or eq8b, depending on whether the molecule is linear or non-linear.

 

Linear molecules

An example of a polyatomic linear molecule is CO₂, which has the same expressions for , and as those for diatomic molecules. The symmetric stretch of CO₂ is IR inactive because the net electric dipole moment is always zero (see diagram above).

 

 

In contrast, the antisymmetric stretch is IR active because it produces a dipole moment that varies with displacement. Since CO₂ is a closed-shell molecule, . Furthermore, this vibrational mode does not produce vibrational angular momentum (). Therefore, the absorption of the photon’s angular momentum must be accounted for by , resulting in (P-branch or R-branch).

The degenerate bending modes are also IR active but differ from the asymmetric stretch in an important way: they can involve vibrational angular momentum. Because the two bending modes occur in perpendicular planes and are degenerate, their linear combination can produce a circular (or elliptical) motion of the O atoms about the C atom, effectively giving rise to vibrational angular momentum. This allows the molecule to absorb the photon’s angular momentum along an alternate pathway without requiring a change in the rotational quantum number. In other words, after photon absorption, but the vector addition of and can result in the magnitude of being unchanged (possible when ), enabling transitions to appear in the IR spectrum as the Q-branch.

 

Symmetric tops

For a symmetric top, its molecular rotational energy is given by eq51. Therefore, eq9 becomes:

Any allowed vibrational transition of a symmetric rotor like CH₃I can involve a change in the dipole moment along the molecule’s symmetry axis (known as a parallel transition), as well as changes in and , which are perpendicular to (perpendicular transitions). The general selection rules governing parallel transitions for symmetric rotors are given by eq8:

while those for perpendicular transitions are given by eq8a:

It follows that the parallel transition expressions for , and  are the same as those for linear molecules. For perpendicular transitions, each branch has two sub-branches depending on :

 

Therefore, the IR spectrum for CH₃I (see diagram above) is more complicated than that for CO₂. Furthermore, there are vibrational modes, which are categorised into six types:

The first three modes are symmetric vibrations (see diagram below), with a change in dipole moment parallel to the C₃ axis. The remaining three modes are doubly degenerate asymmetric vibrations, with a change in dipole moment perpendicular to the C₃ axis.

 

Spherical tops

Although a spherical top is non-polar overall, some of its vibrational modes are IR active due to temporary dipole moments during vibration. The first-order approximation of the vibration-rotation energy levels of a spherical top is also given by eq9, with the selection rules governing IR-active transitions between these levels being the same as those for linear molecules. An example is CH₄, which has vibrational modes (see table and diagram below): one symmetric stretch, one doubly degenerate bend, and two triply degenerate modes (one stretch and one bend).

Mode	Symmetry	Description	Wavenumber /cm-1
		Symmetric C-H stretch	IR inactive
		Symmetric bend (scissoring)	IR inactive
		Asymmetric C-H stretch	3020
		Asymmetric bend (umbrella)	1300

However, only the modes are IR active due to their symmetry and ability to cause a dynamic dipole moment (see diagram below).

In conclusion, the derivation of the three branches using the first-order vibration-rotation energy levels (eq9 and eq17) of an IR-active polyatomic molecule allows us to analyse most IR spectra with ease. However, these energy levels are more complex due to changes in at higher , centrifugal distortion, anharmonicity and vibration-rotation interactions such as Coriolis coupling.

 

Next article: coriolis effect and Coriolis coupling

Previous article: Vibration-rotation spectra of diatomic molecules

Content page of rovibrational spectroscopy

Content page of advanced chemistry

Main content page

Vibration-rotation spectra arise from the simultaneous vibrational and rotational transitions of molecules, typically observed in the infrared (IR) region of the electromagnetic spectrum. These spectra provide detailed information about molecular structure, bond strength, and moment of inertia.

For a molecule to be IR active, it must have a permanent dipole or a dipole moment that changes over time. Homonuclear diatomic molecules, such as O₂, lack these properties and are therefore IR inactive. In contrast, when a heteronuclear diatomic molecule absorbs IR radiation, it can undergo a change in its vibrational energy level along with a change in its rotational state, resulting in a distinctive pattern of spectral lines. These energy levels (expressed in wavenumbers), characterised by the rigid rotor–harmonic oscillator approximation, are the sum of its allowed rotational and vibrational energies:

The selection rules governing transitions are given by eq7:

However, the condition applies only to molecules with a nonzero projection of the electronic orbital angular momentum onto the internuclear axis (i.e. ), such as NO. To elaborate, the rotational quantum number represents the magnitude of the coupled total angular momentum vector, given by

where:

is the nuclear rotation angular momentum (end-over-end rotation).
is the electronic orbital angular momentum.
is the vibrational angular momentum.
is the electronic spin angular momentum.

Note: Nuclear spin angular momentum is excluded because it couples only weakly to the other components.

 

 

The selection rules for a pure vibrational transition are , while those for pure rotational transitions (ignoring the -related fine structure transitions, which are often unresolved in most experimental spectra) are . Additionally, the total angular momentum of the system must be conserved during a photon-mediated transition, as a photon carries one unit of angular momentum.

For a closed shell heteronuclear diatomic molecule like HCl ( ), , with . Therefore, the absorption of the IR photon’s angular momentum during must be accounted for by , resulting in .

Conversely, the ground state of NO () has an unpaired electron in a degenerate molecular orbital, giving , , . This allows the coupling (vector addition) of and to reorient internally in such a way that it can exactly compensate for the photon’s angular momentum (see diagram above). In other words,  can be preserved without changing , making the transitions , where , no longer forbidden.

In general, the absorption lines in a vibration-rotation spectrum can be grouped into three types, called branches (see diagram below). The R branch consists of all transitions, with energies given by:

where .

transitions form the P branch, with energies:

where .

The energy separation between adjacent lines in the P and R branches corresponds to . Therefore, the vibration-rotation spectrum, like the pure rotation spectrum, allows the moment of inertia and bond length of molecules to be calculated. The relative intensities of the lines in the P and R branches depend on the product of and , the same mechanism that governs the intensities in a pure rotational spectrum.

 

 

Finally, the Q branch, if allowed by selection rules, consists of  transitions, all of which have the same energy:

This means that transitions such as and appear as a single line in the spectrum under the rigid rotor-harmonic oscillator approximation. In reality, the Q branch appears as a series of closely spaced lines rather than a single line. This is because changes with the vibrational quantum number , where the bond length increases slightly in higher vibrational states, causing the moment of inertia to increase and to decrease. From eq9,

Eq14 shows that transitions such as and now appear as separate lines. Since , the spacing between these lines decreases with . At lower resolution, these closely spaced lines give the Q-branch the characteristic appearance of a single, strong, broad peak.

Using the same logic,

which rearranges to:

As the spacing between the lines of the R-branch decreases slightly as increases.

 

 

For the P-branch,

Since the third term on RHS of eq16 is negative, the lines of the P-branch diverge slightly as increases. Note that eq14, eq15 and eq16 reduce to eq13, eq11 and eq12 respectively if .

 

 

Next article: Vibration-rotation spectra of polyatomic molecules

Previous article: Selection rules for Vibration-rotation transitions

Content page of rovibrational spectroscopy

Content page of advanced chemistry

Main content page

The selection rules for vibration-rotation transitions govern which simultaneous changes in vibrational and rotational energy levels are allowed when a molecule absorbs or emits infrared radiation.

These rules arise from quantum mechanical principles and the requirement that transitions involve a change in the molecule’s electric dipole moment , and they combine the selection rules for pure vibrational and pure rotational transitions.

In determining the selections rules for pure vibrational and pure rotational transitions, we evaluated conditions under which and  separately. However, the selection rules for vibration-rotation transitions require that . To show that this ultimately reduces to the selection rules for pure vibrational and pure rotational transitions, we begin with

where , and are the orthogonal lab-frame axes; , and are the orthogonal principal axes of inertia of the molecule (molecular frame); and the ’s are unit vectors along the axes.

The relationship between the two frames is shown in the diagram above, with the origin of both frames commonly placed at the centre of mass of the molecule. The lab-frame is stationary in space and does not rotate with the molecule, whereas the molecular frame moves with the molecule and rotates as it does. , known as the line of nodes, denotes the line passing through the intersections of the and planes. The orientation of the molecular frame with respect to the lab frame is described by the Euler angles , , and .

From eq1,

where , and .

Similarly, and . However, we can simplify the analysis to , which can always be arbitrarily chosen as the polarisation direction of the incident oscillating radiation.

To express the cosines in terms of the Euler angles, we need to derive the full rotation matrix according to the convention of a specific sequence of three consecutive elemental rotations to describe any 3D orientation.

The first rotation is about the lab-frame -axis by :

The second rotation is about the axis, also known as the line of nodes , by . It lies in the initial -plane but is fixed in its direction after the first rotation and is perpendicular to both the initial -axis and the final -axis:

The last rotation is about the axis, which is also the molecular -axis, by :

Therefore, the full rotation matrix is:

A rotation matrix is the transpose of the change of basis matrix , which in this case is

Therefore,

and eq2 becomes

We can also determine and in terms of the Euler angles by repeating the steps above.

Now, certain vibrational motions change a molecule’s electric dipole moment, such that , where represents the normal coordinates typically used to describe vibrational motions. Similarly, rotational motions also affect the dipole moment, with , where collectively denotes the Euler angles. This implies that the dipole moment is a function of both and , i.e. . Since the components , and in eq3 are projections of onto the molecular axes, which rotate with the molecule, they are independent of the Euler angles, and must therefore be functions of alone.

If we assume that the atoms in the molecule vibrate with a small amplitude about their equilibrium positions, we can expand , and  in a Taylor series, e.g.

where for non-linear polyatomic molecules and for linear molecules.

Substituting eq4 (by ignoring the higher terms) and the corresponding expansions for and  into gives:

where

.
.
.
.
.

 

 

Since the vibrational wavefunctions are orthonormal, the first integral on RHS of eq5 is non-zero only if for all , i.e. when there is no vibrational transition. Assuming , the selection rules associated with this term pertain to pure rotational transitions. The second term is non-zero only if both and the terms in the square bracket are non-zero. This implies that the selection rules associated with the 2^nd term correspond to those of both pure vibrational and pure rotational transitions.

For a polar linear molecule with as the molecular axis, while , and the first term on RHS of eq5 reduces to if for all . Furthermore,  for non-degenerate normal modes like stretching, but may not be zero for degenerate modes like bending. Despite that, and therefore, the general selection rules for vibration-rotation transitions of polar linear molecules, which require for linearly polarised light, are:

In practice, eq6 is written more simply as:

The condition is omitted because the -related fine structure is often unresolved in most experimental spectra. Additionally, the inclusion of highlights that total angular momentum conservation must be satisfied during a photon-mediated vibrational transition, even though is forbidden for most linear molecules in electric dipole transitions.

Eq7 also corresponds to be the vibration-rotation selection rules for spherical tops.

For a symmetric rotor, any allowed vibrational transition can involve a change in along the symmetry axis (known as parallel transitions), as well as changes in and , which are perpendicular to (perpendicular transitions). Since the pure rotational selection rules for a symmetric rotor (neglecting -related fine structure), where the permanent dipole lies along the symmetry axis, are , the selection rules for parallel transitions are:

In the case of a perpendicular transition, and , while . This results in the following selection rules:

 

 

Combining eq8 and eq8a, the general vibration-rotation selection rules for a symmetric rotor are:

 

Next article: Vibration-rotation spectra of diatomic molecules

Content page of rovibrational spectroscopy

Content page of advanced chemistry

Main content page