Radial wavefunction

The radial wavefunction $R_{n,l}(r)$ describes the probability distribution of the distance between an electron and the nucleus of a hydrogenic atom.

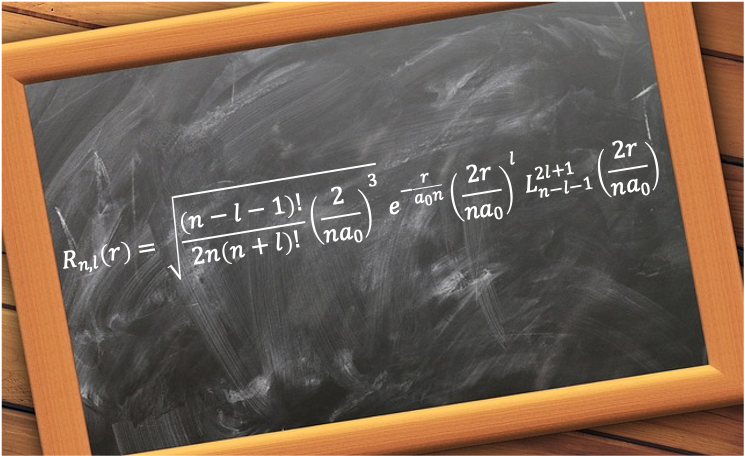
It is given by

$R_{n,l}(r)=\sqrt{\frac{(n-l-1)!}{2n(n+l)!}\biggr$\frac{2}{na_0}\biggr$^3}e^{-\frac{r}{a_0n}}\biggr$\frac{2r}{na_0}\biggr$^lL_{n-l-1}^{2l+1}\biggr$\frac{2r}{na_0}\biggr$\;\;\;\;\;\;\;\;458$

where

$L_{n-l-1}^{2l+1}$ are the associated Laguerre polynomials.
$n$ is the principal quantum number.
$l$ is the orbital angular momentum quantum number
$a_0$ is the Bohr radius.
$r$ is the distance between an electron and the nucleus of a hydrogenic atom.

Question

What is a hydrogenic atom?

Answer

It is an atom with only one electron, regardless of the number of protons in its nucleus, making it similar to a hydrogen atom in terms of its electronic structure. Examples of hydrogenic atoms include the hydrogen atom, He⁺, Li²⁺, and others.

To derive the un-normalised form of eq458, consider the Schrodinger equation of a hydrogenic atom, which is a two-particle problem. Utilising the concepts of center of mass and reduced mass, we have

$\biggr$-\frac{\hbar^2}{2M}\nabla^2_{cm}-\frac{\hbar^2}{2\mu}\nabla^2_{rm}-\frac{Ze^2}{4\pi\varepsilon_0r}\biggr$\psi=E\psi\;\;\;\;\;\;\;\;459$

where

$-\frac{\hbar^2}{2M}\nabla^2_{cm}$ is the kinetic energy operator of the translational motion of the system.
$-\frac{\hbar^2}{2\mu}\nabla^2_{rm}$ is the kinetic energy operator of the internal motion (rotational and vibrational motions) of the system.
$M$ is the combined masses of the electron and the nucleus.
$\mu$ is the reduced mass.
$\nabla^2_{cm}$ and $\nabla^2_{rm}$ are the laplacian operators acting on the centre of mass coordinates and the reduced mass coordinates, respectively.
$\hbar$ is the ratio of the Planck constant and $2\pi$ .
$Z$ is the atomic number of the atom.
$\varepsilon_0$ is the vacuum permittivity.
$\psi$ is the total wavefunction of the atom.
$E$ is the eigenvalue corresponding to $\psi$ .

Since translational motion is independent from rotational and vibrational motions, $E=E_M+E_{\mu}$ , where $E_M$ and $E_{\mu}$ are the translational energy of the system and the internal motion energy of the system respectively. This implies that $\psi=\psi_M\psi_{\mu}$ . Noting that translational energy is purely kinetic, we can separate eq459 into two one-particle problems:

$-\frac{\hbar^2}{2M}\nabla^2_{cm}\psi_M=E_M\psi_M\;\;\;\;\;\;\;\;460$

$\biggr$-\frac{\hbar^2}{2\mu}\nabla^2_{rm}-\frac{Ze^2}{4\pi\varepsilon_0r}\biggr$\psi_{\mu}=E_{\mu}\psi_{\mu}\;\;\;\;\;\;\;\;461$

Eq460 is associated with the translational motion of the entire atom. Therefore, we are only concerned with eq461, which corresponds to the motion of the electron relative to the nucleus. Since ${\nabla^2_{rm}=\frac{\partial^2}{\partial r^2}+\frac{2}{r}\frac{\partial}{\partial r}+\frac{1}{r^2}\biggr$\frac{1}{sin^2\theta}\frac{\partial^2}{\partial\phi^2}+\frac{1}{sin\theta}\frac{\partial}{\partial\theta}sin\theta\frac{\partial}{\partial\theta}\biggr$}$ (see this article for derivation), we can assume that $\psi_{\mu}=R(r)Y(\theta,\phi)$ , where $Y(\theta,\phi)$ are the spherical harmonics. Multiplying eq461 through by $\frac{r}{Y(\theta,\phi)}$ and recognising that ${\frac{d^2R(r)r}{dr^2}=r\frac{d^2R(r)}{dr^2}+2\frac{dR(r)}{dr}}$ gives

$-\frac{\hbar^2}{2\mu}\frac{d^2\rho}{dr^2}-\frac{\hbar^2\rho}{2\mu r^2Y(\theta\phi)}\biggr$\frac{1}{sin^2\theta}\frac{\partial^2}{\partial\phi^2}+\frac{1}{sin\theta}\frac{\partial}{\partial\theta}sin\theta\frac{\partial}{\partial\theta}\biggr$Y(\theta,\phi)-\frac{Ze^2\rho}{4\pi\varepsilon_0r}=E_{\mu}\rho$

where $\rho=R(r)r$ .

Substituting eq96 and eq133 yields the radial differential equation:

$-\frac{\hbar^2}{2\mu}\frac{d^2\rho}{dr^2}+\frac{l(l+1)\hbar^2\rho}{2\mu r^2}-\frac{Ze^2\rho}{4\pi\varepsilon_0r}=E_{\mu}\rho$

where $l=0,1,2,\cdots$ .

Question

If $b=\lambda r$ , show that $\frac{1}{\lambda^2}\frac{d^2\rho}{dr^2}=\frac{d^2\rho}{db^2}$ .

Answer

Substituting $\frac{db}{dr}=\lambda$ in the chain rule $\frac{d\rho}{dr}=\frac{d\rho}{db}\frac{db}{dr}$ gives $\frac{d\rho}{dr}=\lambda\frac{d\rho}{db}$ , which when substituted in $\frac{d^2\rho}{dr^2}=\frac{d}{dr}\frac{d\rho}{dr}$ yields $\frac{d^2\rho}{dr}=\lambda\frac{d}{dr}\frac{d\rho}{db}$ . Substituting $\frac{db}{dr}=\lambda$ in the chain rule $\frac{d}{dr}\biggr$\frac{d\rho}{db}\biggr$=\frac{d}{db}\biggr$\frac{d\rho}{db}\biggr$\frac{db}{dr}$ results in $\frac{d}{dr}\biggr$\frac{d\rho}{db}\biggr$=\lambda\frac{d^2\rho}{db^2}$ . Finally substituting $\frac{d\rho}{dr}=\lambda\frac{d\rho}{db}$ in $\frac{d}{dr}\biggr$\frac{d\rho}{db}\biggr$=\lambda\frac{d^2\rho}{db^2}$ gives $\frac{1}{\lambda^2}\frac{d^2\rho}{dr^2}=\frac{d^2\rho}{db^2}$ .

Letting $\lambda^2=-\frac{2\mu E_{\mu}}{\hbar^2}$ , $a=\frac{\mu Ze^2}{2\pi\varepsilon_0\hbar^2\lambda}$ , $b=\lambda r$ and noting that $\frac{1}{\lambda^2}\frac{d^2\rho}{dr^2}=\frac{d^2\rho}{db^2}$ gives

$\frac{d^2\rho}{db^2}=\biggr\[1-\frac{a}{b}+\frac{l(l+1)}{b^2}\biggr\]\rho\;\;\;\;\;\;\;\;462$

To determine the solution to eq462, we analyse its asymptotes. As $b\rightarrow\infty$ , eq462 approximates to $\frac{d^2\rho}{db^2}=\rho$ , which has a possible solution of $\rho(b)=Ae^{-b}$ , where $A$ is a constant. When $b\rightarrow 0$ , the $b^{-2}$ term dominates, giving $\frac{d^2\rho}{db^2}=\frac{l(l+1)}{b^2}\rho$ , which has a solution of $\rho(b)=Bb^{l+1}$ , where $B$ is a constant. Each solution on its own is not square-integrable over the interval $0\leq b\leq\infty$ . However, we can combine them to give a square-integrable form: $\rho(b)=ABb^{l+1}e^{-b}$ .

Question

Verify that $\rho(b)=ABb^{l+1}e^{-b}$ is a solution to eq462. Hence, show that $\rho(b)=b^{l+1}e^{-b}v(b)$ , where $v(b)=\sum_{k=0}^{\infty}C_kb^k$ , is also a solution to eq462.

Answer

Substituting the second derivative of $\rho(b)=ABb^{l+1}e^{-b}$ in eq462, we get $a=2(l+1)$ , which when substituted in $a=\frac{\mu Ze^2}{2\pi\varepsilon_0\hbar^2\lambda}$ and then in $\lambda^2=-\frac{2\mu E_{\mu}}{\hbar^2}$ yields the eigenvalue $E_{\mu}=-\frac{\mu}{2\hbar^2}\biggr\[\frac{Ze^2}{4\pi\varepsilon_0(l+1)}\biggr\]^2$ .

Consider $\rho(b)=Cb^{l+1+k}e^{-b}$ , where $k=0,1,2,\cdots$ . Substituting the second derivative of $\rho(b)=Cb^{l+1+k}e^{-b}$ in eq462, we get $a=2(l+1+k)$ and the eigenvalue $E_{\mu}=-\frac{\mu}{2\hbar^2}\biggr\[\frac{Ze^2}{4\pi\varepsilon_0(l+1+k)}\biggr\]^2$ , which implies that $\rho(b)=Cb^{l+1+k}e^{-b}$ is a solution to eq462. Since eq462 is a linear differential equation, each term in $\rho(b)=b^{l+1}e^{-b}\sum_{k=0}^{\infty}C_kb^k=C_0b^{l+1}e^{-b}+C_1b^{l+2}e^{-b}+\cdots$ , and hence the entire function, is a solution to eq462. We can simplify $a=2(l+1+k)$ to $a=2n$ , where $n=1,2,\cdots$ because $l=0,1,2,\cdots$ and $k=0,1,2,\cdots$ .

$n$ is known as the principal quantum number. Since $n=l+1+k$ , we have

$l\leq n-1\;\;\;\;\;\;\;\;462a$

Consequently, the eigenvalue associated with eq462, and hence with the radial differential equation, is a function of $n$ :

$E_n=-\frac{Z^2\mu e^4}{32\pi^2\varepsilon_0^2\hbar^2}\frac{1}{n^2}\;\;\;\;n=1,2,\cdots\;\;\;\;\;\;462b$

where we have replaced $E_{\mu}$ with $E_n$ .

Substituting $a=2n$ , $\rho(b)=b^{l+1}e^{-b}v(b)$ and its second derivative in eq462 gives

$b\frac{d^2v(b)}{db^2}+2(l+1-b)\frac{dv(b)}{db}+2(n-l-1)v(b)=0\;\;\;\;\;\;\;\;463$

To solve eq463, we transform it into an associated Laguerre differential equation, which has known solutions. This is accomplished by setting $x=2b$ . Then, $db=\frac{dx}{2}$ , $db^2=\frac{dx^2}{4}$ and eq463 becomes

$x\frac{d^2v(b)}{dx^2}+[2(l+1)-x]\frac{dv(b)}{dx}+[n-(l+1)]v(b)=0\;\;\;\;\;\;\;\;464$

Eq464 is an associated Laguerre differential equation. Comparing eq464 and eq442, we have

$x\frac{d^2L_{n-l-1}^{2l+1}(x)}{dx^2}+[2(l+1)-x]\frac{dL_{n-l-1}^{2l+1}(x)}{dx}+[n-(l+1)]L_{n-l-1}^{2l+1}(x)=0$

where $L_{n-l-1}^{2l+1}(x)$ are the associated Laguerre polynomials.

The explicit expression of $L_{n-l-1}^{2l+1}(x)$ can then be found by carrying out the following substitutions:

1. Substituting $a=2n$ in $\lambda=\frac{\mu Ze^2}{2\pi\varepsilon_0\hbar^2a}$ yields $\lambda=\frac{1}{na_0}$ , where $a_0=\frac{4\pi\varepsilon_0\hbar^2}{\mu Ze^2}$ is the Bohr radius.
2. Substituting $\lambda=\frac{1}{na_0}$ in $b=\lambda r$ gives $b=\frac{r}{na_0}$ .
3. Substituting $b=\frac{r}{na_0}$ in $x=2b$ results in $x=\frac{2r}{na_0}$ .
4. Substituting $x=\frac{2r}{na_0}$ in eq444, where $m=2l+1$ and $n=n-l-1$ , gives

$L_{n-l-1}^{21+1}\biggr$\frac{2r}{na_0}\biggr$=\sum_{k=0}^{n-l-1}(-1)^k\frac{(n+l)!}{k!(k+2l+1)!(n-l-1-k)!}\biggr$\frac{2r}{na_0}\biggr$^k\;\;\;\;\;\;\;\;465$

Substituting $\rho=R(r)r$ in $\rho=b^{l+1}e^{-b}v(b)$ yields $R(r)=\frac{1}{r}b^{l+1}e^{-b}v(b)$ . Substituting $b=\frac{r}{na_0}$ and noting that $v(b)=L_{n-l-1}^{2l+1}\biggr$\frac{2r}{na_0}\biggr$$ , we have

$R(r)=\frac{1}{r}\biggr$\frac{r}{na_0}\biggr$^{l+1}e^{-\frac{r}{na_0}}L_{n-l-1}^{2l+1}\biggr$\frac{2r}{na_0}\biggr$\;\;\;\;\;\;\;\;466$

Eq466 is the un-normalised radial wavefunction for a hydrogenic atom. To derive its normalisation constant $N$ , we begin by substituting $m=2l+1$ , $k=n-l-1$ and $x=\frac{2r}{na_0}$ in eq457 to give

$\int_0^{\infty}\biggr\[\biggr$\frac{r}{na_0}\biggr$^{2l+2}e^{-\frac{2r}{na_0}}L_{n-l-1}^{2l+1}\biggr$\frac{2r}{na_0}\biggr$L_{n-l-1}^{2l+1}\biggr$\frac{2r}{na_0}\biggr$\biggr\]dr=\frac{2n(n+l)!}{(n-l-1)!}\frac{na_0}{2^{2l+3}}\;\;\;\;\;467$

The expression for the normalisation of eq466 is $\int_0^{\infty}N^2\vert R(r)\vert^2r^2dr=1$ or equivalently

$N^2\int_0^{\infty}\biggr$\frac{r}{na_0}\biggr$^{2l+2}e^{-\frac{2r}{na_0}}L_{n-l-1}^{2l+1}\biggr$\frac{2r}{na_0}\biggr$L_{n-l-1}^{2l+1}\biggr$\frac{2r}{na_0}\biggr$dr=1$
Substituting eq467 yields

$N=\sqrt{\frac{(n-l-1)!}{2n(n+l)!}\frac{2^{2l+3}}{na_0}}\;\;\;\;\;\;\;\;468$

Therefore, the normalised radial wavefunction is

$R_{n,l}(r)=\sqrt{\frac{(n-l-1)!}{2n(n+l)!}\frac{2^{2l+3}}{na_0}}\frac{1}{r}\biggr$\frac{r}{na_0}\biggr$^{l+1}e^{-\frac{r}{na_0}}L_{n-l-1}^{2l+1}\biggr$\frac{2r}{na_0}\biggr$$

which can be easily rearranged to eq458.

Radial wavefunction

Question

Answer

Question

Answer

Question

Answer

Next article: Total wavefunction of a hydrogenic atom

Previous article: Normalisation constant of the associated Laguerre polynomials

Content page of quantum mechanics

Content page of advanced chemistry

Main content page

Leave a Reply Cancel reply