Advanced Chemistry - Mono Mole

July 13, 2022August 13, 2025

Completeness of a vector space

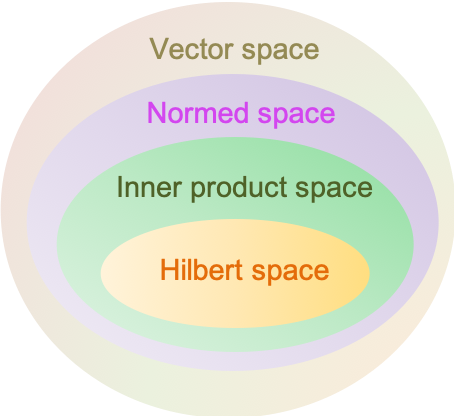
A complete vector space is one that has no “missing elements” (e.g. no missing coordinates).

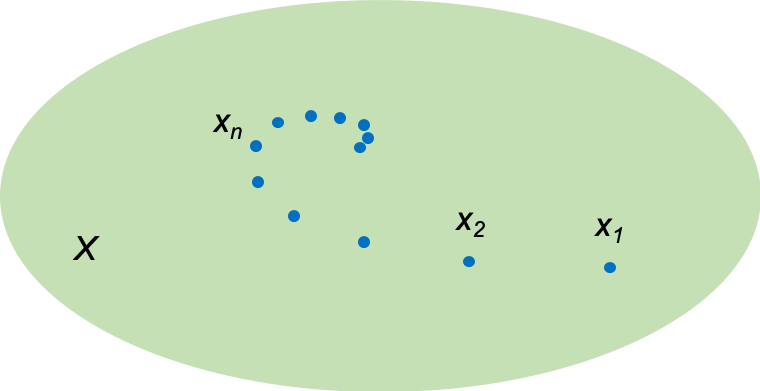
In the previous article, we learned that the function $d(\boldsymbol{\mathit{u}},\boldsymbol{\mathit{v}})$ defines the distance between two elements of the vector space. Such a function is called a metric and it measures the ‘closeness’ of elements (or points) in a vector space. Since a vector space is a collection of elements, we can use a sequence, e.g. $\left \{ x_n \right \}_{n=1}^{\infty}$ , to represent elements of a vector space $X$ . If the distance between two members of the sequence gets closer as $n$ gets larger (see diagram below), i.e.

$\lim_{m,n\rightarrow \infty}d(x_n-x_m)=0$

we call the sequence a Cauchy sequence.

Cauchy sequences are useful in determining the completeness of a vector space. A vector space $V$ is complete if every Cauchy sequence in $V$ converges to an element of $V$ . For example, the sequence $\left \{ x_n \right \}_{n=1}^{\infty}$ , where $x_n=\sum_{k=1}^{n}\frac{(-1)^{k+1}}{k}$ , is one of many Cauchy sequences of rational numbers in the rational number space $\mathbb{Q}$ . However, the sequence converges to $ln2$ , which is not an element of $\mathbb{Q}$ . Therefore, $\mathbb{Q}$ is not complete, and has “missing elements” or “gaps”, as compared to the real space number space $\mathbb{R}$ , which is complete.

Question

Show that $\sum_{k=1}^{\infty}\frac{(-1)^{k+1}}{k}=ln2$ .

Answer

If $\left | x \right |<1$ , then $(1-x)(1+x+x^{2}+\cdots)$ . So, $\frac{1}{1-x}=1+x+x^{2}+\cdots$ or

$\frac{1}{1-(-x)}=\frac{1}{1+x}=1-x+x^{2}+\cdots$

Integrating the 2^nd equality of the above equation on both sides gives

$ln\left | 1+x \right |=x-\frac{x^{2}}{2}+\frac{x^{3}}{3}+\cdots=\sum_{k=1}^{\infty}(-1)^{k+1}\frac{x^{k}}{k}$

Substituting $x=1$ in the above equation yields $ln2=\sum_{k=1}^{\infty}\frac{(-1)^{k+1}}{k}$ .

A vector space with no “missing elements” is essential for scientists to work with to formulate theories (e.g. kinematics) and solving problems associated with those theories. Furthermore, the ability to compute limits in a complete vector space implies that we can apply calculus to solve problems defined by the space. For example, a complete inner product space called a Hilbert space, which will be discussed in the next article, is used to formulate the theories of quantum mechanics.

Next article: hilbert space

Previous article: Vector subspace and eigenspace

Content page of quantum mechanics

Content page of advanced chemistry

Main content page

July 13, 2022July 14, 2025

Inner product space

An inner product space is a vector space with an inner product.

An inner product is an operation that assigns a scalar to a pair of vectors $\langle\boldsymbol{\mathit{u}}\vert\boldsymbol{\mathit{v}}\rangle$ , or a scalar to a pair of functions $\langle f\vert g\rangle$ . The way to assign the scalar may be through the matrix multiplication of the pair of vectors, for instance

$\langle\boldsymbol{\mathit{u}}\vert\boldsymbol{\mathit{v}}\rangle=\begin{pmatrix} u_{1}^{*} &u_{2}^{*}& \cdots &u_{N}^{*} \end{pmatrix}\begin{pmatrix} v_1\\v_2 \\ \vdots \\ v_N \end{pmatrix}=\sum_{i=1}^{N}u_{i}^{*}v_i\; \; \; \; \; \; \; \; 3$

or it may be through an integral of the pair of functions:

$\langle f\vert g\rangle=\int_{-\infty}^{\infty}f(x)^{*}g(x)dx$

You may notice that eq3 resembles a dot product. The dot product pertains to vectors in $\mathbb{R}^{3}$ , where $\boldsymbol{\mathit{A}}\cdot\boldsymbol{\mathit{B}}=\sum_{i=1}^{3}A_iB_i$ , which can be extended to $N$ -dimensions, where $\langle\boldsymbol{\mathit{A}}\vert\boldsymbol{\mathit{B}}\rangle=\sum_{i=1}^{N}A_iB_i$ , and to include complex and real functions, $\langle f\vert g\rangle=\int_{-\infty}^{\infty}f(x)^{*}g(x)dx$ . Therefore, an inner product is a generalisation of the dot product.

An inner product space has the following properties:

1. Conjugate symmetry: $\langle f\vert g\rangle=\langle g\vert f\rangle^{*}$
2. Additivity: $\langle f+g\vert h\rangle=\langle f\vert h\rangle+\langle g\vert h\rangle$
3. Positive semi-definiteness: $\langle f\vert f\rangle\geq 0$ , with $\langle f\vert f\rangle= 0$ if $f=0$

Question

i) Why is the inner product space positive semi-definite?
ii) Show that orthogonal vectors are linearly independent.
iii) Prove that $\boldsymbol{\mathit{A}}\cdot\boldsymbol{\mathit{B}}=\vert\boldsymbol{\mathit{A}}\vert\vert\boldsymbol{\mathit{B}}\vert cos\theta$ .

Answer

i) A general vector space of $\langle\boldsymbol{\mathit{a}}\vert\boldsymbol{\mathit{a}}\rangle$ can be positive or negative. The inner product space is defined such that $\langle f\vert f\rangle\geq 0$ , with $\langle f\vert f\rangle= 0$ if $f=0$ , which is useful in quantum mechanics.

ii) Let the set of vectors $\left \{ \boldsymbol{\mathit{v_k}} \right \}$ in eq1 be orthogonal vectors. The dot product of eq1 with $\boldsymbol{\mathit{v_i}}$ gives $c_i\boldsymbol{\mathit{v_i}}\cdot\boldsymbol{\mathit{v_i}} =c_i\left |\boldsymbol{\mathit{v_i}} \right |^{2}=0$ . Since the magnitudes of orthogonal vectors are non-zero, $c_i=0$ . Hence, orthogonal vectors are linearly independent.

iii) Let’s consider two vectors $\boldsymbol{\mathit{A}}$ and $\boldsymbol{\mathit{B}}$ as position vectors starting from the origin. Then the vector $\boldsymbol{\mathit{A-B}}$ forms a triangle with them. According to the law of cosines, we have:

$\vert\boldsymbol{\mathit{A-B}}\vert^2=\vert\boldsymbol{\mathit{A}}\vert^2+\vert\boldsymbol{\mathit{B}}\vert^2-2\vert\boldsymbol{\mathit{A}}\vert\vert\boldsymbol{\mathit{B}}\vert cos\theta$

Substituting $\vert\boldsymbol{\mathit{A-B}}\vert^2=$\boldsymbol{\mathit{A-B}}$\cdot$\boldsymbol{\mathit{A-B}}$=\vert\boldsymbol{\mathit{A}}\vert^2-2\boldsymbol{\mathit{A}}\cdot\boldsymbol{\mathit{B}}+\vert\boldsymbol{\mathit{B}}\vert^2$ into the above equation gives:

$\boldsymbol{\mathit{A}}\cdot\boldsymbol{\mathit{B}}=\vert\boldsymbol{\mathit{A}}\vert\vert\boldsymbol{\mathit{B}}\vert cos\theta$

which completes the proof.

Two functions (or two vectors) are orthogonal if $\langle f\vert g\rangle= 0$ . Elements of a set of basis functions are orthonormal if $\langle \phi_i\vert \phi_j\rangle=\delta_{ij}$ where

$\delta_{ij}= \{\; \begin{matrix} 1 & for\; \; i=j\\ 0 & for\; \; i\neq j \end{matrix}$

In other words, two functions (or two vectors) are orthonormal if they are orthogonal and normalised.

Finally, the norm (or length) of a vector $\boldsymbol{\mathit{u}}$ is denoted by $\left \|\boldsymbol{\mathit{u}}\right \|$ and is defined as $\left \|\boldsymbol{\mathit{u}}\right \|=\sqrt{\langle\boldsymbol{\mathit{u}}\vert\boldsymbol{\mathit{u}}\rangle}=\sqrt{\left |\boldsymbol{\mathit{u}}\right |\left |\boldsymbol{\mathit{u}}\right |cos\: 0^{\circ} }=\left |\boldsymbol{\mathit{u}}\right |$ . With this association of inner product and the length of a vector, we can establish the relationship between inner product $\langle\boldsymbol{\mathit{u}}\vert\boldsymbol{\mathit{v}}\rangle$ and the Euclidean distance $d(\boldsymbol{\mathit{u}},\boldsymbol{\mathit{v}})$ between 2 vectors $\boldsymbol{\mathit{u}}$ and $\boldsymbol{\mathit{v}}$ . Using the $\mathbb{R}^{2}$ space as an example, where $\boldsymbol{\mathit{u}}=\begin{pmatrix} 6\\9 \end{pmatrix}$ and $\boldsymbol{\mathit{v}}=\begin{pmatrix} 3\\5 \end{pmatrix}$ , we have

$d(\boldsymbol{\mathit{u}},\boldsymbol{\mathit{v}})=\left \|\boldsymbol{\mathit{u}}-\boldsymbol{\mathit{v}}\right \|=\sqrt{\langle\boldsymbol{\mathit{u}}- \boldsymbol{\mathit{v}}\vert\boldsymbol{\mathit{u}}- \boldsymbol{\mathit{v}}\rangle}=\sqrt{\langle\begin{pmatrix} 3\\4 \end{pmatrix}\vert \begin{pmatrix} 3\\4 \end{pmatrix}\rangle}$

$=\sqrt{\begin{pmatrix} 3 &4 \end{pmatrix}\begin{pmatrix} 3\\4 \end{pmatrix}}=5$

Next article: vector subspace and eigenspace

Previous article: vector space of functions