The orthogonality of the associated Legendre polynomials states that the integral of the product of two distinct associated Legendre polynomials over a specified interval is zero.
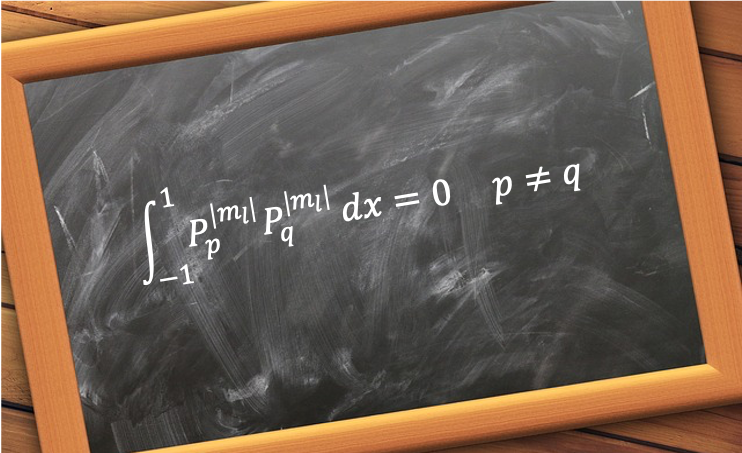
It is defined mathematically as:
The values of 
differ for the two associated Legendre polynomials, while the values of 
are the same. This is because the eigenvalue of an associated Legendre polynomial is a function of .
Question
Why are the limits of integration in eq380 from -1 to 1?
Answer
The associated Legendre polynomials are used to describe spherical harmonics, where 
and 
. Therefore, the orthogonality of the associated Legendre polynomials is analysed within the interval of 
.
To prove eq380, we substitute eq364 in the LHS of eq380 to give
^{\vert m_l\vert}}{2^{p+q}p!q!}\int_{-1}^1X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\frac{d^{q+\vert m_l\vert}X^q}{dx^{q+\vert m_l\vert}}dx\;\;\;\;\;\;\;\;381)
where 
and )
.
The proof involves carrying out the integral on the RHS of eq381 by parts 
times. Let 
and 
. So dx)
and 
.
We begin with the integration of the RHS of eq381 by parts times. The first integration yields
^{\vert m_l\vert}}{2^{p+q}p!q!}\biggr\{\biggr\[X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\frac{d^{q+\vert m_l\vert-1}X^q}{dx^{q+\vert m_l\vert-1}}\biggr\]^1_{-1}-\int_{-1}^1\frac{d^{q+\vert m_l\vert-1}X^q}{dx^{q+\vert m_l\vert-1}}\frac{d}{dx}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)dx\biggr\})
This implies that 
. Otherwise, the first integration would be the first of 
integrations by parts. Since , the boundary term 
equals zero and
^{\vert m_l\vert+1}}{2^{p+q}p!q!}\int_{-1}^1\frac{d^{q+\vert m_l\vert-1}X^q}{dx^{q+\vert m_l\vert-1}}\frac{d}{dx}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)dx)
Let )
and 
. Then, dx)
and 
. Repeating the integration by parts a second time, we have
^{\vert m_l\vert+1}}{2^{p+q}p!q!}\biggr\{\biggr\[\frac{d}{dx}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)\frac{d^{q+\vert m_l\vert-2}X^q}{dx^{q+\vert m_l\vert-2}}\biggr\]^1_{-1}-\int_{-1}^1\frac{d^{q+\vert m_l\vert-2}X^q}{dx^{q+\vert m_l\vert-2}}\frac{d^2}{dx^2}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)dx\biggr\})
=\vert m_l\vert X^{\vert m_l\vert-1}2x\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}+X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert+1}X^p}{dx^{p+\vert m_l\vert+1}})
. Using the same logic mentioned in the first integration, 
and the boundary term again vanishes, resulting in
^{\vert m_l\vert+2}}{2^{p+q}p!q!}\int_{-1}^1\frac{d^{q+\vert m_l\vert-2}X^q}{dx^{q+\vert m_l\vert-2}}\frac{d^2}{dx^2}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)dx)
For subsequent integrations, the boundary term includes )
, which retains the factor 
in each term after carrying out the derivatives. Hence, for integrations by parts, the boundary term vanishes after each integration, giving
^{2\vert m_l\vert}}{2^{p+q}p!q!}\int_{-1}^1\frac{d^{q}X^q}{dx^{q}}\frac{d^{\vert m_l\vert}}{dx^{\vert m_l\vert}}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)dx)
For the first of integrations by parts, let )
and 
. Then, dx)
and 
. We have
^{2\vert m_l\vert}}{2^{p+q}p!q!}\biggr\{\biggr\[\frac{d^{\vert m_l\vert}}{dx^{\vert m_l\vert}}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)\frac{d^{q-1}X^q}{dx^{q-1}}\biggr\]^1_{-1}-\int_{-1}^1\frac{d^{q-1}X^q}{dx^{q-1}}\frac{d^{\vert m_l\vert+1}}{dx^{\vert m_l\vert+1}}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)dx\biggr\})
Since 
, the boundary term again vanishes, resulting in
^{2\vert m_l\vert+1}}{2^{p+q}p!q!}\int_{-1}^1\frac{d^{q-1}X^q}{dx^{q-1}}\frac{d^{\vert m_l\vert+1}}{dx^{\vert m_l\vert+1}}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)dx)
For the second integration by parts till the 
integration by parts, 
, where 
. Therefore, the boundary term vanishes each time. The final integration by parts gives
^{2\vert m_l\vert+q-1}}{2^{p+q}p!q!}\biggr\{\biggr\[\frac{d^{\vert m_l\vert+q}}{dx^{\vert m_l\vert+q}}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)\frac{d^{q-q}X^q}{dx^{q-q}}\biggr\]^1_{-1}-\int_{-1}^1\frac{d^{q-q}X^q}{dx^{q-q}}\frac{d^{\vert m_l\vert+q}}{dx^{\vert m_l\vert+q}}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)dx\biggr\})
In other words, for integrations by parts, we have
^{2\vert m_l\vert+q}}{2^{p+q}p!q!}\int_{-1}^1X^q\frac{d^{\vert m_l\vert+q}}{dx^{\vert m_l\vert+q}}\biggr\(X^{\vert m_l\vert}\frac{d^{p+\vert m_l\vert}X^p}{dx^{p+\vert m_l\vert}}\biggr\)dx\;\;\;\;\;\;\;\;382)
Using Leibniz’s theorem,
=\sum_{k=0}^{\vert m_l\vert+q}\begin{pmatrix}\vert m_l\vert+q\\k\end{pmatrix}\biggr\(\frac{d^k}{dx^k}X^{\vert m_l\vert}\biggr\)\frac{d^{2\vert m_l\vert+p+q-k}X^p}{dx^{2\vert m_l\vert+p+q-k}})
Since ^{\vert m_l\vert})
is a polynomial of degree 
, then 
only when 
. Similarly, 
only when 
, or equivalently, when 
. These two conditions imply that the only non-zero term in the sum occurs when 
and 
. Eq382 becomes,
^{2\vert m_l\vert+q}}{2^{2q}(q!)^2}\begin{pmatrix}\vert m_l\vert+q\\2\vert m_l\vert\end{pmatrix}\int_{-1}^1X^q\biggr\(\frac{d^{2\vert m_l\vert}}{dx^{2\vert m_l\vert}}X^{\vert m_l\vert}\biggr\)\frac{d^{2q}X^q}{dx^{2q}}dx\;\;\;\;\;\;\;\;383)
which is equivalent to eq380 when 
.