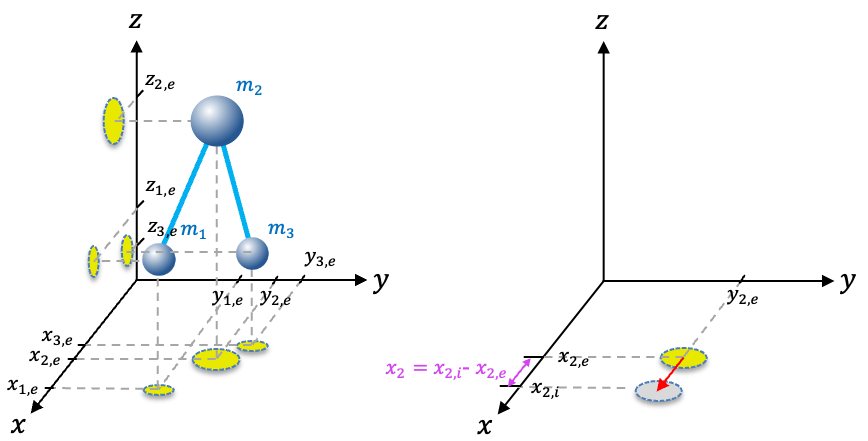
Vibration of polyatomic molecules

The vibration of polyatomic molecules involves stretching, bending and torsional motions.

The classical kinetic energy $T$ of a vibrating $N$ -nuclei molecule is

$T=\frac{1}{2}\sum_{\alpha=1}^Nm_{\alpha}\biggr\[\biggr$\frac{dx_{\alpha}}{dt}\biggr$^2+\biggr$\frac{dy_{\alpha}}{dt}\biggr$^2+\biggr$\frac{dz_{\alpha}}{dt}\biggr$^2\biggr\]\;\;\;\;\;\;\;\;85$

where $x_{\alpha}$ , $y_{\alpha}$ and $z_{\alpha}$ are the displacements of the $\alpha$ -th nucleus from its equilibrium position of $(x_{\alpha,e},y_{\alpha,e},z_{\alpha,e})$ (see diagram below).

Eq85 can be simplified by changing the Cartesian displacement coordinates $x_{\alpha},y_{\alpha},z_{\alpha}$ to mass-weighted displacement coordinates $q_1,\cdots,q_{3N}$ , where

$\begin{matrix} q_1=m_1^{1/2}x_1&q_2=m_1^{1/2}y_1&q_3=m_1^{1/2}z_1\\q_4=m_2^{1/2}x_2&\cdots &q_{3N}=m_N^{1/2}z_N\end{matrix}$

which gives

$T=\frac{1}{2}\sum_{i=1}^{3N}\dot q^2_i=\frac{1}{2}(\dot q_1\cdots \dot q_{3N})\begin{pmatrix}\dot q_1\\\vdots\\\dot q_{3N}\end{pmatrix}=\frac{1}{2}\boldsymbol{\mathit{\dot q}}^T\boldsymbol{\mathit{\dot q}}\;\;\;\;\;\;\;\;86$

where $\dot q_{i}=\frac{dq_i}{dt}$ and $\boldsymbol{\mathit{\dot q}}$ is a column vector with elements $\dot q_1,\cdots,\dot q_{3N}$ .

The potential energy $U$ of a vibrating $N$ -nuclei molecule is also a function of $x_{\alpha},y_{\alpha},z_{\alpha}$ and hence a function of $q_1,\cdots,q_{3N}$ . We can approximate the multivariable function $U$ by expanding it in a Maclaurin series around the equilibrium positions:

$U(q_1,\cdots,q_{3N})=U(q_{1,e=0},\cdots,q_{3N,e=0})+\sum_{i=1}^{3N}\frac{\partial U(q_{1,e=0},\cdots,q_{3N,e=0})}{\partial q_i}q_i+\frac{1}{2!}\sum_{i,j=1}^{3N}\frac{\partial^2 U(q_{1,e=0},\cdots,q_{3N,e=0})}{\partial q_i\partial q_j}q_iq_j+\cdots$

which can be abbreviated to

$U=U_e+\sum_{i=1}^{3N}\biggr$\frac{\partial U}{\partial q_i}\biggr$_eq_i+\frac{1}{2!}\sum_{i,j=1}^{3N}\biggr$\frac{\partial^2 U}{\partial q_i\partial q_j}\biggr$_eq_iq_j+\cdots$

When the nuclei are at their equilibrium positions, the slope of is zero, i.e. ${\biggr$\frac{\partial U}{\partial q_i}\biggr$_e=0}$ . For small vibrations, where $q_i$ is close to $q_{i,e}$ , we ignore all the higher power terms, giving

$U=U_e+\frac{1}{2}\sum_{i,j=1}^{3N}\biggr$\frac{\partial^2 U}{\partial q_i\partial q_j}\biggr$_eq_iq_j\;\;\;\;\;\;\;\;87$

or in matrix notation

$U=U_e+\frac{1}{2}\boldsymbol{\mathit{q}}^T\boldsymbol{\mathit{Uq}}\;\;\;\;\;\;\;\;88$

where $\boldsymbol{\mathit{q}}$ is the column vector with elements $q_1,\cdots,q_{3N}$ and $\boldsymbol{\mathit{U}}$ is a $3N\times 3N$ matrix, called the Hessian matrix, with elements $u_{ij}=\small{\biggr$\frac{\partial^2 U}{\partial q_i\partial q_j}\biggr$_e}$ .

Question

Show that $\boldsymbol{\mathit{q}}^T\boldsymbol{\mathit{Uq}}=\sum_{i,j=1}^{3N}\biggr$\frac{\partial^2 U}{\partial q_i\partial q_j}\biggr$_e$ .

Answer

$\begin{pmatrix}q_1&q_2&\cdots&q_{3N}\end{pmatrix}\begin{pmatrix}u_{11}&u_{12}&\cdots&u_{1j}\\u_{21}&u_{22}&\cdots&u_{2j}\\\vdots&\vdots&\ddots&\vdots\\u_{i1}&u_{i2}&\cdots&u_{ij}\end{pmatrix}\begin{pmatrix}q_1\\q_2\\\vdots\\q_{3N}\end{pmatrix}=\\\begin{pmatrix}q_1&q_2&\cdots&q_{3N}\end{pmatrix}\begin{pmatrix}\sum_{j=1}^{3N}u_{1j}q_j\\\sum_{j=1}^{3N}u_{2j}q_j\\\vdots\\\sum_{j=1}^{3N}u_{ij}q_j\end{pmatrix}=\sum_{i,j=1}^{3N}u_{ij}q_iq_j$

We want to derive an eigenvalue equation that resembles the harmonic oscillator equation so that we can easily solve for the vibrational energies of a polyatomic molecule. However, the Hamiltonian formed by combining eq86 and eq87 doesn’t resemble the Hamiltonian of a harmonic oscillator. Therefore, we need to further simply eq86 and eq87 with a change of variables.

With regard to eq88, the potential energy of a system is a real-valued physical property. This implies that $\boldsymbol{\mathit{U}}$ must consists of real-valued entries $u_{ij}$ . Since $u_{ij}=\small{\biggr$\frac{\partial^2 U}{\partial q_i\partial q_j}\biggr$_e=\biggr$\frac{\partial^2 U}{\partial q_j\partial q_i}\biggr$_e=u_{ji}}$ , the matrix $\boldsymbol{\mathit{U}}$ is both real and symmetric.

Question

i) Prove that eigenvectors of a real and symmetric matrix $O$ with distinct eigenvalues are orthogonal.

ii) What if some of the eigenvalues are degenerate?

Answer

i) Let $Ox=ax$ and $Oy=by$ , where $a\neq b$ . Multiply the first eigenvalue equation by $y^T$ , we have

$y^Tax=(y^TO)x=(O^Ty)^Tx=by^Tx$

where the 2^nd equality uses the identity mentioned in this article, while the 3^rd equality employs the property of a symmetrc matrix.

Since $a\neq b$ , we have $y^Tx=0$ , i.e. $y$ is orthogonal to $x$ .

ii) When some eigenvalues are degenerate, the corresponding eigenvectors belong to the same eigenspace. Since we can always select a set of linearly independent eigenvectors that span the eigenspace, these eigenvectors can be orthogonalised using the Gram-Schmidt process without changing the eigenvalue. So, for a real symmetric matrix, we can always find a basis of eigenvectors that are orthogonal to each other, regardless of whether the eigenvalues are distinct or degenerate.

Let the orthogonal eigenvectors $\boldsymbol{\mathit{l}}_m$ of $\boldsymbol{\mathit{U}}$ be the columns of the matrix $\boldsymbol{\mathit{L}}$ , where $\boldsymbol{\mathit{L}}^T\boldsymbol{\mathit{L=I}}$ . As shown in this article, we can express the eigenvalue problem $\boldsymbol{\mathit{Ul}}_m=\lambda_j\boldsymbol{\mathit{l}}_m$ as

$\boldsymbol{\mathit{UL=L\Lambda}}\;\;\;or\;\;\;\boldsymbol{\mathit{L}}^T\boldsymbol{\mathit{UL=\Lambda}}$

where $\boldsymbol{\mathit{\Lambda}}$ is the diagonal eigenvalue matrix with diagonal entries $\lambda_m$ .

Computing the secular equation $det(u_{ij}-\lambda_m\delta_{ij})$ allows us to find $\lambda_m$ , and solving the simultaneous equations of $((\boldsymbol{\mathit{U}}-\lambda_m \boldsymbol{\mathit{I}})\boldsymbol{\mathit{l}}_m=0$ , which is equivalent to $\sum_{j=1}^{3N}(u_{ij}-\lambda_m\delta_{ij})l_{jm}=0$ (where $i=1,\cdots,3N$ , enables us to find $\boldsymbol{\mathit{l}}_m$ .

Consider the following linear combinations of mass-weighted displacement coordinates

$Q_k=\sum_{j=1}^{3N}l_{jk}q_{j}=\boldsymbol{\mathit{L}}^T\boldsymbol{\mathit{q=Q}}\;\;\;\;\;\;k=1,\cdots,3N\;\;\;\;\;\;\;\;89$

where $\boldsymbol{\mathit{Q}}$ is the column vector with elements $Q_1,\cdots,Q_{3N}$ .

Since $l_{jk}$ are the components of the $k$ -th orthogonal eigenvector of $\boldsymbol{\mathit{U}}$ and each basis $q_j$ describes the displacement of a nucleus, $Q_k$ , known as the normal coordinates, is a set of orthogonal vectors that expresses $3N$ independent motions (called degrees of freedom) of the molecule as a whole.

Substituting $\boldsymbol{\mathit{q=LQ}}$ in eq88

$U=U_e+\frac{1}{2}(\boldsymbol{\mathit{LQ}})^T\boldsymbol{\mathit{ULQ}}=U_e+\frac{1}{2}\boldsymbol{\mathit{Q}}^T\boldsymbol{\mathit{L}}^T\boldsymbol{\mathit{ULQ}}=U_e+\frac{1}{2}\boldsymbol{\mathit{Q}}^T\boldsymbol{\mathit{\Lambda Q}}=U_e+\frac{1}{2}\sum_{j=1}^{3N}\lambda_iQ_j^2\;\;\;\;\;\;\;\;90$

Since $\boldsymbol{\mathit{q=LQ}}=\sum_{j=1}^{3N}l_{kj}Q_j=q_k$ , we have $\frac{dq_k}{dt}=\sum_{j=1}^{3N}l_{kj}\frac{dQ_j}{dt}$ or $\boldsymbol{\mathit{\dot q=L\dot Q}}$ and eq86 becomes

$T=\frac{1}{2}\boldsymbol{\mathit{\dot q}}^T\boldsymbol{\mathit{\dot q}}=\frac{1}{2}(\boldsymbol{\mathit{L\dot Q}})^T\boldsymbol{\mathit{L\dot Q}}=\frac{1}{2}\boldsymbol{\mathit{\dot Q}}^T\boldsymbol{\mathit{L}}^T\boldsymbol{\mathit{L\dot Q}}=\frac{1}{2}\boldsymbol{\mathit{\dot Q}}^T\boldsymbol{\mathit{\dot Q}}=\frac{1}{2}\sum_{j=1}^{3N}\dot Q_j^2\;\;\;\;\;\;\;\;91$

Combining eq90 and eq91, the classical total energy of the system is $E=\frac{1}{2}\sum_{j=1}^{3N}\dot Q_j^2+\frac{1}{2}\sum_{j=1}^{3N}\lambda_j Q_j^2$ , where we have set $U_e=0$ . This is because we are usually interested in computing transition energies and the eigenvalue corresponding to $U_e$ is subtracted out when we calculate vibrational transition energies for a given electronic state. The Hamiltonian is derived by replacing the classical observables by quantum-mechanical operators:

$\hat{H}=-\frac{\hbar^2}{2}\sum_{j=1}^{3N}\frac{\partial^2}{\partial Q^2_j}+\frac{1}{2}\sum_{j=1}^{3N}\lambda_j Q_j^2\;\;\;\;\;\;\;\;92$

Question

Show that the classical kinetic energy term $\frac{1}{2}\sum_{k=1}^{3N}\dot Q_k^2$ can be replaced by the quantum-mechanical operator $-\frac{\hbar^2}{2}\sum_{k=1}^{3N}\frac{\partial^2}{\partial Q^2_k}$ .

Answer

From eq89, $\dot Q_k=\sum_{j=1}^{3N}l_{kj}\dot q_j$ . Substituting the momentum operator $\hat{p}_1=m_1\dot x_1=\frac{\hbar}{i}\frac{\partial}{\partial x_1}$ in $\dot q_1=m_1^{1/2}\dot x_1$ , we have $\dot q_1=m_1^{-1/2}\frac{\hbar}{i}\frac{\partial}{\partial x_1}$ . Using the single-variable chain rule $\frac{\partial}{\partial x_1}=\frac{\partial}{\partial q_1}\frac{\partial q_1}{\partial x_1}$ , we have $\frac{\partial}{\partial x_1}=m_1^{1/2}\frac{\partial}{\partial q_1}$ , which when substituted in $\dot q_1$ gives $\dot q_1=\frac{\hbar}{i}\frac{\partial }{\partial q_1}$ . We can find similar expressions for $\dot q_2,\cdots,\dot q_{3N}$ . So, $\dot Q_k=\frac{\hbar}{i}\sum_{j=1}^{3N}l_{jk}\frac{\partial}{\partial q_j}$ . Since $U$ is a function of $Q_k$ , and $Q_k$ is a function of $q_j$ , the multi-variable chain rule gives $\frac{\partial}{\partial Q_k}=\sum_{j=1}^{3N}\frac{\partial}{\partial q_j}\frac{\partial q_j}{\partial Q_k}$ . Relabelling $q_k=\sum_{j=1}^{3N}l_{kj}Q_j$ as $q_j=\sum_{k=1}^{3N}l_{jk}Q_k$ , we have $\frac{\partial q_j}{\partial Q_k}=l_{jk}$ and therefore, $\frac{\partial}{\partial Q_k}=\sum_{j=1}^{3N}l_{jk}\frac{\partial}{\partial q_j}$ , which when substituted in $\dot Q_k$ gives $\dot Q_k=\frac{\hbar}{i}\frac{\partial}{\partial Q_k}$ . Substituting this last expression of $\dot Q_k$ in $\frac{1}{2}\sum_{k=1}^{3N}\dot Q_k^2$ gives $-\frac{\hbar^2}{2}\sum_{k=1}^{3N}\frac{\partial^2}{\partial Q_k^2}$ .

The kinetic energy term in eq92 involves a change in $Q_j$ , which is a function of $q_k$ and hence a function of the displacement coordinates $x_{\alpha}$ , $y_{\alpha}$ and $z_{\alpha}$ , which are relative to the equilibrium positions of the nuclei. In other words, $Q_j$ is a function of the displacements of all the nuclei from their respective equilibrium positions. For the molecule as a whole, all the equilibrium positions of the nuclei can be taken as the center of mass of the molecule. Since translational and rotational motions of the molecule involve no displacement relative to the molecule’s centre of mass, $\frac{\partial^2}{\partial Q_j^2}=0$ for such motions. Furthermore, translational and rotational motions are purely kinetic with no potential energy component. Therefore, we can rewrite eq92 as:

$\hat{H}=-\frac{\hbar^2}{2}\sum_{j=1}^m\frac{\partial^2}{\partial Q_j^2}+\frac{1}{2}\sum_{j=1}^m\lambda_jQ_j^2\;\;\;\;\;\;\;\;92$

where $m=3N-6$ and $m=3N-5$ for a non-linear molecule and a linear molecule respectively.

Each $m$ is called a normal mode and corresponds to a unique vibrational motion of the molecule. The Schrodinger equation is

$-\frac{\hbar^2}{2}\sum_{j=1}^m\frac{\partial^2}{\partial Q_j^2}\psi_{vib}+\frac{1}{2}\sum_{j=1}^m\lambda_jQ_j^2\psi_{vib}=E\psi_{vib}\;\;\;\;\;\;\;\;93$

where the separation of variables technique allows us to approximate the total vibrational wavefunction as $\psi_{vib}=\prod_{j=1}^m\psi_j(Q_j)$ and hence, $E=\sum_{j=1}^mE_j$ .

It follows that each $\psi_j(Q_j)$ describes a normal mode of the molecule. Comparing with eq4a, eq93 is the Schrodinger equation for a harmonic oscillator of unit mass and force constant $\lambda_j$ . This implies that (see eq46)

$\psi_j(Q_j)=N_jH_j\biggr$\sqrt{\frac{\omega_j}{\hbar}}Q_j\biggr$e^{-\frac{\omega_jQ^2_j}{2\hbar}}\;\;\;\;\;\;\;\;94$

where $N_j=\sqrt[4]{\frac{\omega_j}{\pi\hbar}}\frac{1}{\sqrt{2^nn!}}$ is the normalisation constant for the Hermite polynomials $H_j$ .

Therefore, eq93 can be solved by computing $m$ individual Schrodinger equations. With reference to eq21, each solution gives

$E_j=\biggr$n_j+\frac{1}{2}\biggr$\hbar\omega_j\;\;\;\;\;\;n_j=0,1,2,\cdots\;\;\;\;\;\;\;\;95$

where $n_j$ is the vibrational quantum number of the $j$ -th normal mode of the molecule.

The total vibrational energy is

$E=\sum_{j=1}^m\biggr$n_j+\frac{1}{2}\biggr$\hbar\omega_j\;\;\;\;\;\;\;\;96$

The vibrational state of a polyatomic molecule $\vert n_1,n_2,\cdots,n_m\rangle$ is characterised by $m$ quantum numbers. For example the vibrational ground state of $H_2O$ , where $m=3N-6=3$ , is $\vert 0,0,0\rangle$ . The ground state vibrational wavefunction of any polyatomic molecule is

$\vert 0,0,\cdots,0\rangle=\psi_0=N\prod_{j=1}^mH_j\biggr$\sqrt{\frac{\omega_j}{\hbar}}Q_j\biggr$e^{-\frac{\omega_jQ_j^2}{2\hbar}}=N\prod_{j=1}^me^{-\frac{\omega_jQ_j^2}{2\hbar}}\;\;\;\;\;\;\;\;97$

Eq97 is a Gaussian function that is symmetrical in $Q_j$ . The implication of this in group theory is that the ground state vibrational wavefunction of any polyatomic molecule is totally symmetric under all symmetry operations of the point group that the molecule belongs to. In fact, group theory provides an easy way to determine the vibrational modes of a molecule and to analyse the possible transitions between different vibrational states.

Vibration of polyatomic molecules

Question

Answer

Question

Answer

Question

Answer

Next article: Vibrational selection rules for molecules

Previous article: Anharmonicity

Content page of vibrational spectroscopy

Content page of advanced chemistry

Leave a Reply Cancel reply