A direct product representation of a group is a representation with each element
being the Kronecker product of the elements of two other irreducible representations
and
of
corresponding to the same symmetry operation
.
For example, if the dimensions of and
are 2 and 3 respectively, i.e.
and
, then
In general, if the dimensions of and
are
and
respectively, then the dimension of
is
:

Question
Show that
Answer
With reference to eq75, let’s denote the block by
. So,
If is also a representation of
, then it must be consistent with the closure property of
, i.e.
. The proof is as follows:
where the 2nd equality uses the identity proven in the Q&A above.
With reference to eq75, the trace of is
It follows that the direct product of three representations is
which can be extended to direct products of more than three representations.

Question
With reference to the point group, show that
, while
can be decomposed into the direct sum of
.
Answer
Using eq76, we include and
in the character table of the
point group as follows:

Clearly, , which implies that
is an irreducible representation of
. Since the number of irreducible representations of a point group is equal to the number of classes of the group,
is a reducible representation because its characters are not equivalent to the characters of any of the 3 irreducible representations. We then use eq27 for the decomposition of
, where

Therefore, .
Finally, consider the sets of linearly independent functions
i) , where
ii) , where
iii) , where
that transform according to ,
and
respectively.
Since ,
and
are sets of linearly independent functions, we can write
According to a previous article, we can also write
where is a symmetry operation of
and
,
and
are the matrix entries of
,
and
respectively.

Question
What is the relation between the matrix entries of ,
and
in
?
Answer
Let the matrix entries of ,
and
be
,
and
respectively, where
Using the ordering convention called dictionary order, where the order of or
is given by
or in terms of the notation or
,
In other words, is determined by
and
, and
is determined by
and
. For example, if
and
,
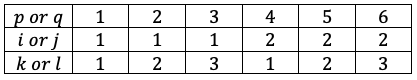
We can then express the matrix entries of as
Multiplying eq77a by eq77b,
From eq77g, we have and we can rewrite the RHS of eq77h as
, where
. Hence, eq77h is equivalent to eq77c. Combining eq77f and eq77h, we have
This implies that if the functions and
are bases for the irreducible representations of
and
of a point group respectively, then
is the basis for
, which is the direct product of
and
. In other words,
If the functions and
transform according to the irreducible representations of
and
of a point group respectively, then the function
, which is the product of the functions
and
must transform according to the direct product of
.

Question
Show that the reducible representation of the direct product of two irreducible representations and
contains the totally symmetric representation if and only if
is the complex-conjugate representation of
.
Answer
Using eq27a, the number of times the totally symmetric representation appears in the decomposition of is
Substitute eq76 in eq78
Comparing eq79 with eq22, if and only if
and vanishes otherwise.