Category: Advanced Chemistry

The rate law for the 3rd order reaction of the type A + 2B → P is:

Using the same logic described in a previous article, we can rewrite the rate law as:

where a = [A₀] and b = [B₀].

Integrating the above equation throughout yields

Substituting the partial fraction expression   in eq15 and working out some algebra gives

 

 

 

Next article: 3rd order reaction of the type (A+B+c→P)

Previous article: 2nd order autocatalytic reaction

Content page of advanced chemical kinetics

Content page of Advanced chemistry

Main content page

An autocatalytic reaction is one in which a product catalyses the reaction. An example is the Mn²⁺-catalysed oxidation of oxalic acid by potassium manganate (VII):

The equation can be reduced to A + P → 2P, or simply, A → P, with the rate law:

Using the same logic described in a previous article, we can rewrite the rate law as:

where a = [A₀] and p = [P₀].

Integrating throughout, we have

Substituting the partial fraction expression in eq14 and working out the algebra yields

which is equivalent to

 

 

 

Next article: 3rd order reaction of the type (A+2B→P)

Previous article: 2nd order reaction of the type (A+2B→P)

Content page of advanced chemical kinetics

Content page of Advanced chemistry

Main content page

The rate law for the 2nd order reaction of the type A + 2B → P is:

Using the same logic described in the previous article, we can rewrite the rate law as:

where a = [A₀] and b = [B₀].

Rearranging the above equation and integrating throughout, we have

Substituting the partial fraction expression in eq13 and after some algebra, we have,

which is equivalent to

 

Next article: 2nd order Autocatalytic reaction

Previous article: 2nd order reaction of the type (A+B→P)

Content page of advanced chemical kinetics

Content page of Advanced chemistry

Main content page

The transition state theory provides a theoretical way to calculate the pre-exponential factor A and the activation energy E_a, and therefore the rate constant k of an elementary chemical reaction. This is in contrast with the Arrhenius equation, where A and E_a are obtained empirically. The theory is based on statistical thermodynamics and can be illustrated for a gas-phase bimolecular reaction using the following assumptions:

1) The reacting system, expressed as , proceeds along an energy path that includes a point of highest potential energy called the saddle point, where a distinct species known as the activated complex  is formed. The conversion of the activated complex to the product P can be seen as an asymmetric vibrational stretch:

2) A rapid pre-equilibrium is established between the activated complex and the reactants: .

3) The rate of the reaction is attributed to the rate-determining step, which is the rate of conversion of the activated complex to the product: , where is proportional to the vibrational frequency of the activated complex.

The expression for the gas-phase rapid equilibrium from assumption 2 is:

which in terms of molar concentration is:

Substituting eq1 into the rate equation , , yields:

In general, the equilibrium constant, when written in terms of standard molar partition functions, is (see this article for derivation), where is the respective stoichiometric coefficient of the J-th species. For our bimolecular reaction,

where .

The activated complex in our example is a linear molecule with N = 3 atoms and therefore has 3N – 5 = 4 modes of vibration (2 bending, 1 symmetric stretch and 1 asymmetric stretch). The standard molar partition function for the activated complex is the product of the partition functions of different modes of motion: , which we can rewrite in the form: , where .

The standard molar partition function for the asymmetric vibration is , where is the vibrational frequency that leads to the conversion of the activated complex to the product. Assuming , . Therefore,

Substituting eq3 into eq2 gives:

where

Substituting eq4 into the rate constant equation yields:

From assumption 3, is proportional to the vibrational frequency of the activated complex,

where is the proportionality constant called the transmission coefficient, which accounts for the notion that not every oscillation of the activated complex leads to its conversion to the product.

Substituting eq6 into eq5 results in:

The standard Gibbs energy is . By analogy, we define the standard Gibbs activation energy as  , which we shall substitute into eq7 to give:

 

 

Eq7 and eq8 are different forms of the Eyring equation. Substituting eq8 in the definition of activation energy and differentiating, noting that and are standard states, which are temperature-independent, gives:

Substituting eq9 into eq8 yields:

Comparing eq10 and the Arrhenius equation, we have:

Repeating all the above steps for a gas-phase unimolecular reaction, we arrive at an activation energy of instead of eq9. Therefore, the transition state theory predicts that the activation energy for a gas-phase elementary reaction is

where for a unimolecular reaction and for a bimolecular reaction.

Next article: 1st order reversible reaction

Content page of advanced chemical kinetics

Content page of Advanced chemistry

Main content page

The rate law for the reaction A + B → P is:

Unlike the 2nd order reaction of the type A → P with a rate law of , eq11 cannot be integrated unless we express [B] in terms of [A], or [A] and [B] in terms of a third variable x. Since A and B react in a ratio of 1:1, the remaining concentrations of A and B at any time of the reaction are:

where [A₀] and [B₀] are the initial concentrations of A and B respectively.

Differentiating both sides of [A] = [A₀] – x with respect to time yields

Therefore, we can rewrite eq11 as

where a = [A₀] and b = [B₀]

Integrating eq12 using the partial fraction expression of gives

After some algebra, we have,

which is equivalent to

 

Next article: 2nd order reaction of the type (A+2B→P)

Previous article: 1st order consecutive reaction

Content page of advanced chemical kinetics

Content page of Advanced chemistry

Main content page

The equilibrium constant of a chemical reaction is the reaction quotient of the reaction at dynamic equilibrium.

The derivation of the equilibrium constant involves the following steps:

1. 1. Derive the expression for the Gibbs energy of a multi-component reaction.
   2. Derive the chemical potential of a multi-component system.
   3. Combine the two expressions.

Step 1

From eq14 of the article on the Nernst equation, the reaction Gibbs energy for a reaction is

and at standard state

Step 2

From eq24 of the article on the Nernst equation, the chemical potential of a multi-component system is

Step 3

Combining eq1 and eq3

Substitute eq2 in eq4

Since lnx^a +lnx^b +… = ln(x^ax^b …), eq5 becomes

Let , where Q is the reaction quotient.

At equilibrium, a reversible reaction is spontaneous in neither direction. Hence the change in Gibbs energy with respect to the change in the extent of the reaction, i.e. the reaction Gibbs energy , is zero. Eq7 becomes

where K is the reaction quotient at equilibrium, i.e.

Since the value K of does not change for a particular reaction at constant temperature, we call it the equilibrium constant.

But how does , which is dimensionless, relate to and ? and are approximations of the thermodynamic equilibrium constant . For an ideal gas,

where .

Similarly, for solutes in dilute solutions,

Eq21a and eq21b show that and are only unitless when . Equating the two expressions gives:

 

Next article: Phase equilibria OVerview

Content page of advanced chemical equilibrium

Content page of Advanced chemistry

Main content page

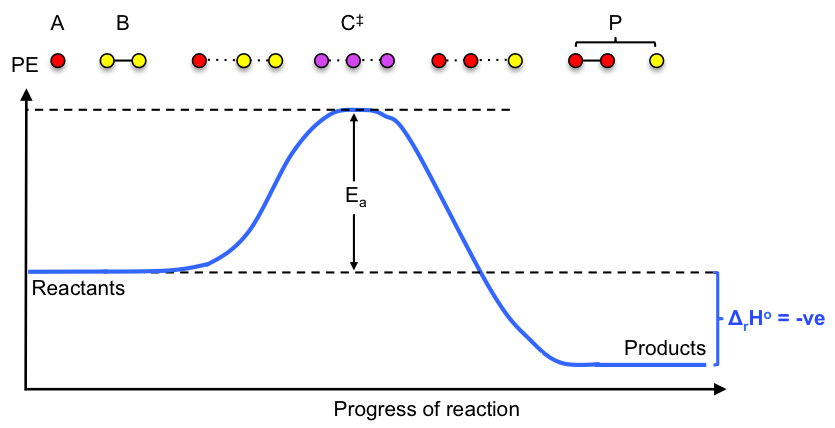
In 1887, Walther Nernst, a German chemist, developed the Nernst equation, which describes the relationship between an electrochemical reaction’s potential and its standard electrode potential under standard and non-standard conditions.

The derivation of the Nernst equation involves the following steps:

1. 1. Derive the formula for the reversible open circuit potential , E^o, of an electrochemical cell at constant temperature and pressure under standard conditions.
   2. Derive the expression for E^o in terms of chemical potentials of the reaction species at constant temperature and pressure.
   3. Derive a ‘correction factor’ for E^o in step2. This final expression, the Nernst equation, describes how the resultant E^o varies with activities of chemical species.

 

Step 1

From the definition of enthalpy, H = U + pV, we have

Substitute the definition of the internal energy of a system undergoing reversible change, dU = dq_rev + dw_rev in eq1

Substitute the definition of the change in Gibbs energy of a system, dG = dH – d(TS) in eq2

At constant temperature and pressure, dT = dp = 0

Substitute the definition of the change in entropy of a system, dS = dq_rev/T in eq3

Substitute the definition of total reversible work, w_rev = w_ex + w_add, where w_ex is reversible expansion work and w_add is reversible additional work other than reversible expansion work, in eq4

Substitute the definition of the change in reversible expansion work, dw_ex = –pdV in eq5

Substitute the definition of the reversible electrical work between two points in an electric circuit, dw_add = –nFEdξ in eq6

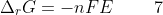
where n is the number of moles of electrons, F is the Faraday constant, E is the open circuit potential of the electrochemical cell and Δ_rG = dG/dξ is the Gibbs energy of the electrochemical cell reaction.

When the open circuit potential of an electrochemical cell is measured at standard conditions (1 bar, 298K, 1M), we write eq7 as:

 

Step 2

The chemical potential of the j-th species, in a multi-component system is

where n‘ is all n other than nj.

The total differential of the Gibbs energy of the system at constant temperature and pressure is

Substituting eq9 in eq10

Eq11 can be expressed in another way by considering the reversible reaction

with the change in Gibbs energy at constant temperature and pressure of the system given by eq11. Next, let’s define

where vj is the stoichiometric number of the j-th species in the reversible reaction and dξ is the amount of substance that is being changed in the reaction.

ξ is called the extent of a reaction and has units of amount in moles. Note that the stoichiometric numbers for reactants are negative by convention and those for products are positive. Substituting eq12 in eq11

Substitute the definition of the Gibbs energy of an electrochemical cell reaction, , into eq13

Substitute eq7 from Step1 in eq14

When the open circuit potential of an electrochemical cell is measured at standard conditions (1 bar, 298K, 1M), we write eq15 as:

 

Step 3

Firstly, let’s consider a system with a single pure substance. Substitute the definition of the change in Gibbs energy of a system, dG = dH – d(TS), in the change of enthalpy of the system dH = dU + d(pV)

The reversible change in internal energy of a pure substance (a system with a single pure substance only does expansion work) can be expressed as

Substitute the definitions of entropy, dS = dq_rev/T, and expansion work, –pdV, in eq18

Substitute eq19 in eq17

At constant temperature, dT = 0 and eq20 becomes dG = Vdp. Integrating this new expression on both sides from  to ,

Let p_i = 1 bar = p^o (i.e. standard conditions) and p_f = p

Secondly, we extend the above working to a multi-component system of ideal gases. Since G is an extensive property, we add the Gibbs energies of all components to give the total Gibbs energy of the system:

Let’s assume the reference pressures of the component gases are the same since they are all ideal gases, i.e. p_a^o = p_b^o = p^o 

For simplicity, let G_Total and G^o_Total be G and G^o respectively.

Taking the partial derivative of the above with respect to n_j, at constant temperature, pressure and the amount of the other components, n‘, we have

For the last partial derivative of the above equation, only the j-th term survives.

Substitute eq9 from Step 2 in eq21

Similarly, for a solute in a dilute solution satisfying Henry’s law, we can write

where c_j and c^o are the j-th solute’s concentration and molar concentration respectively.

We can fine-tune eq22 and eq23 by replacing p_j/p^o and c_j/c^o with γ_j(p_j/p^o) and γ_j(c_j/c^o) where γ_j is a factor called the activity coefficient that can account for both ideal and non-ideal fluids (γ = 1 and γ < 1 for ideal and non-ideal fluids respectively). Next, we combine the modified equations into a single equation by letting a_j = γ_j(p_j/p^o) and a_j = γ_j(c_j/c^o), where a_j is the activity of species j.

Substituting eq24 in eq15 from Step 2,

Substituting eq16 from Step 2 in eq25

where Q is the reaction quotient with Q = . 

Hence, is the ‘correction factor’ for E^o, as the activities of reaction species vary. Eq26 is the Nernst equation.

For an electrochemical equation of the form , the Nernst equation can be written as:

Note that since E^o is by definition a reduction potential, the reference equation is always and hence eq27.

 

Next article: pH glass electrode

Content page of advanced electrochemistry

Content page of Advanced chemistry

Main content page

A typical experiment setup for pH measurement consists of a glass electrode (indicator electrode), a reference electrode (a regular AgCl electrode), the analyte and a potentiometer. The potentiometer ensures that current flow is negligible in the electrochemical circuit so that IR drop and overpotential are minimised, resulting in more accurate measurements. Such a method in electrochemistry where current is negligible is called potentiometry.

Comparing the setup with a circuit diagram (see above diagram), the overall potential of the electrochemical cell is:

The liquid junction potential arises due to the difference in mobility of K⁺ and Cl^– in the controlled flow of the saturated KCl solution out of the porous frit of the external reference AgCl electrode (to complete the circuit). E_analyte = IR = 0 since current flow is negligible in potentiometric methods.

 

 

Lastly, before a pH meter is put to use, it is calibrated using solutions of known pH. The reason for this is as follows:

Substituting eq30 from the previous article in eq31,

where L’ = L – E_{ext ref elec} + E_{liq jn}.

Since E_{liq jn} cannot be calculated or measured directly, the value of L’ must be determined by calibrating the pH meter with solutions of known pH.

 

Next article: IR drop

Previous article: pH glass electrode

Content page of advanced electrochemistry

Content page of Advanced chemistry

Main content page

IR drop in electrochemistry is the voltage drop due to the resistance of the analyte (and the internal KCl solution if a calomel or AgCl electrode is used) in a close circuit.

In the previous article regarding pH measurement, which involves potentiometry, no net current flows in the circuit. When a current flows, e.g. in coulometry and electrogravimetry, we have to consider two effects:

• • IR drop
  • Overpotential

We can represent IR drop as an internal resistance of a battery in the following circuit diagram:

The general equation for a galvanic cell is:

where E_{cell, open} = E_R – E_L.

Eq32 when applied to a Zn electrochemical cell gives the following values:

E_{cell, open} and E_galvanic for the above example are recorded as negative values by the voltmeters because of the way the terminals of the voltmeters are connected to the respective circuits. From eq32, is always less than .

For an electrolytic cell,

If no current is flowing in the electrolytic cell, eq33 reduces to E_applied = E_{cell, open}. For electrolysis to proceed, . For example, the variation of E_applied for the electrolytic reduction of Zn²⁺ is illustrated by the following diagram:

Once again, E_applied for the above example is recorded as a negative value by the voltmeter because of the way the voltmeter is connected to the circuit.

 

 

Next article: Polarisation

Previous article: pH measurement using a glass electrode

Content page of advanced electrochemistry

Content page of Advanced chemistry

Main content page

A pH glass electrode is an ion-selective electrode that is sensitive to H⁺, and therefore, used to measure pH of solutions. It is composed of a glass bulb that is H⁺-selective and an internal AgCl electrode.

The glass bulb is specially manufactured with a composition of 70+% SiO₂ and 20+% Na₂O. It has a silicate network within the glass matrix, with some Si atoms singly bonded to O atoms and other Si atoms coordinated with Na⁺ (see diagram above). On the outer and inner surfaces of the bulb, some of the Si–O^– arms are also coordinated with Na⁺, while the rest of the Si–O^– are uncoordinated.

Before the pH glass electrode can be used, it must be hydrated (soaked in water of pH = 7) to form two layers of gel. This is to allow H⁺ to diffuse into the gel layers to convert the Si–O^–Na⁺ groups on both surfaces to Si–O^–H⁺. Since the inner surface of the bulb is already in contact with a KCl buffer solution at pH 7, both the inner and outer surfaces develop the same ion-exchange equilibrium when the electrode is dipped in water:

This ion-exchange occurs because H⁺ binds more strongly to SiO^– than Na⁺. Therefore, the position of the equilibrium is almost entirely to the right. On the inner surface, the displaced sodium ions enter the buffer solution, and on the outer surface, displaced sodium ions enter water. The hydrated surfaces now consist of a combination of SiO^–H⁺ and SiO^– arms, and is ready for use. The electrode is immersed in an analyte of unknown pH, establishing the following equilibrium on the outer surface:

and the following equilibrium on the inner surface equilibrium:

If the pH of the analyte is less than 7, the outer surface will have fewer uncoordinated Si–O^– arms and hence, less negative charges as compared to the inner wall, whose equilibrium remains unchanged. The difference in charges on the surfaces produces a potential difference across the glass bulb. Furthermore, it confers enough activation energy for sodium ions within the glass matrix to dissociate from the silicate network. These mobile sodium ions then migrate interstitially across the bulb and along the potential gradient, to complete the circuit.

Even though the above equilibria are ion-exchanges and not redox reactions, the potential across the bulb E_b is found empirically to be Nernst-like, where:

 

 

Comparing with a circuit diagram (see above diagram), the overall potential of the glass electrode is:

where E_{int elec} is the potential of the internal AgCl electrode and E_{int soln} is the potential drop across the saturated KCl solution.

Since no current flows in potentiometric methods,

Furthermore, manufacturing defect or damage of electrode over time results in the electrode recording a non-zero potential when the analyte is pH = 7. We call this an asymmetry potential, which must be included in E_glass:

Substituting eq28 in eq29, where a_inner, E_asym and E_{int elec} are constants,  E_{glass eletrode} at rtp is:

where L = E_{int elec} + E_asym – 0.0592 log a_inner.

Next article: pH measurement using a glass electrode

Previous article: Nernst equation

Content page of advanced electrochemistry

Content page of Advanced chemistry

Main content page