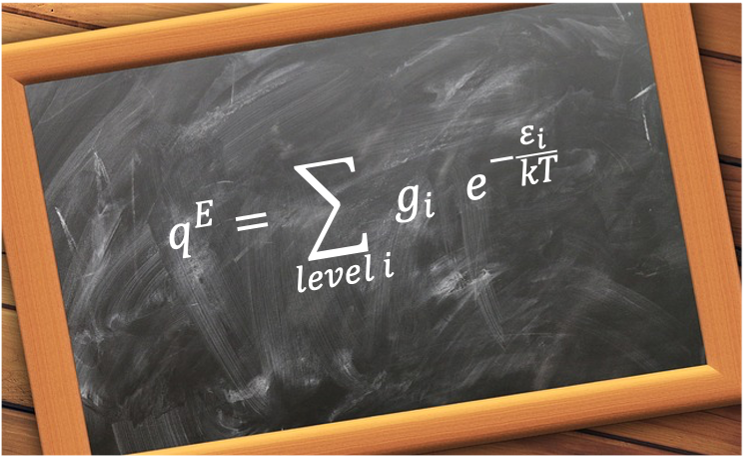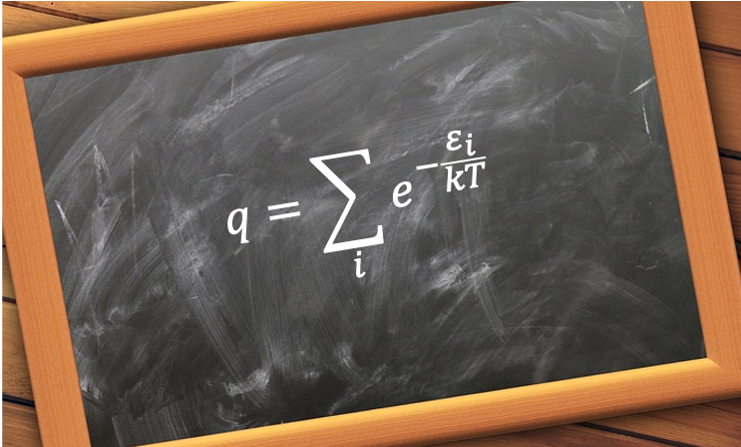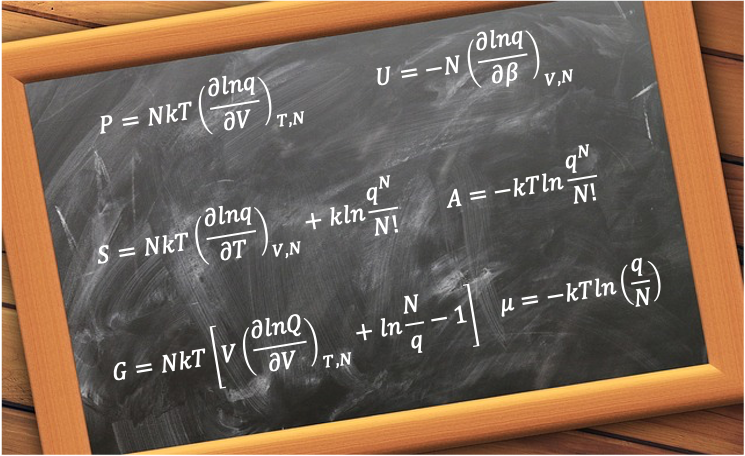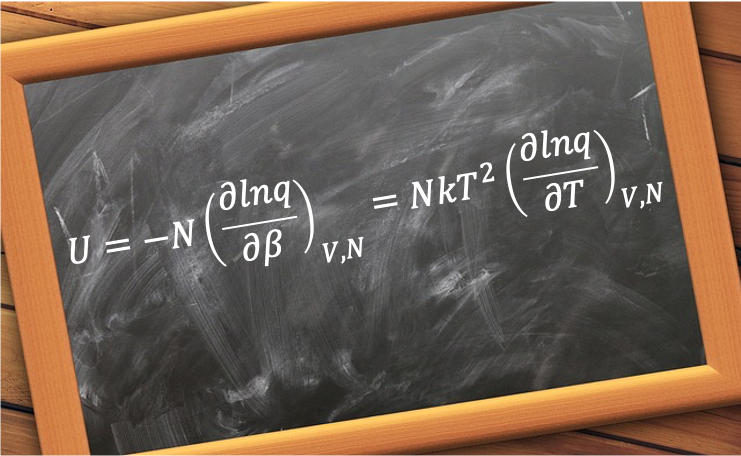The rotational molecular partition function 
is a measure of the number of thermally accessible rotational energy states available to a single non-interacting molecule at a specific temperature.
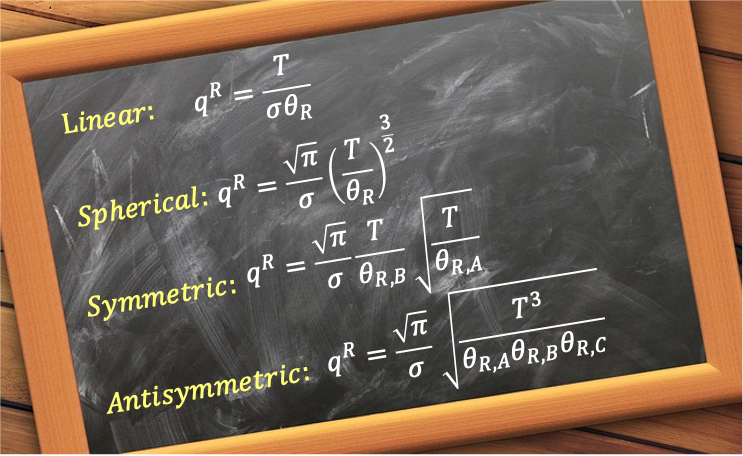
Linear rotors
Consider a heteronuclear diatomic molecule with rotational energies given by eq45: )
. Substituting this equation into the rotational component of eq257, noting that each energy level is )
-fold degenerate, gives:
e^{-\frac{hcBJ(J+1)}{kT}}\;\;\;\;\;\;\;\;270)
At low temperatures, is calculated by substituting experimental values of 
into eq270. At higher temperatures, such as typical laboratory conditions at room temperature, many rotational energy levels become thermally accessible and populated because 
. Since the exponential term varies smoothly with 
at room temperature when }{kT}\ll 1)
, we can simplify eq270 by approximating the sum as an integral:
e^{-\frac{hcBJ(J+1)}{kT}}dJ\;\;\;\;\;\;\;\;271)
Substituting }{kT})
and \frac{hcB}{kT}dJ)
into eq271 yields 
, which evaluates to:
Since 
has units of Kelvin, eq272 can also be expressed as:
where 
is known as the characteristic rotational temperature.
Eq273 is valid only when 
, which is generally true at room temperature. For this reason, it is often referred to as the high-temperature approximation to the rotational partition function.
As discussed in the article on the effect of nuclear statistics on rotational states, nuclear spins dictate which rotational states are populated for symmetrical molecules such as O2 and CO2, resulting in half of all possible rotational states being inaccessible. For such molecules, eq273 becomes:
In general, the rotational partition function for all linear rotors can be written as:
where the symmetry number 
is 1 for non-symmetrical linear molecules like HCl and 2 for for symmetrical linear molecules such as O2 and CO2.
Question
Is unique to rotational partition functions?
Answer
Yes. The symmetry number appears specifically in rotational partition functions. Although we have explained it using quantum mechanics, can also be understood within the framework of classical physics.
At high temperatures, the spacing between rotational energy levels becomes very small compared to 
, making the discrete quantum levels effectively continuous. The rotational partition function is then approximated classically as an integral over phase space, i.e. over all possible orientations and angular momenta. Here, each energy state corresponds to a particular configuration of positions and momenta. However, for symmetric molecules like CO₂, certain rotational operations (such as a 180° rotation around the molecular axis) produce configurations that are physically indistinguishable. To avoid overcounting these indistinguishable orientations, the symmetry number is introduced as a correction factor in the rotational partition function.
Spherical rotors
The rotational partition function for spherical rotors is derived using the same logic as that for linear rotors. Since the rotational energy levels of a spherical rotor is given by the same expression as that for a linear rotor, the rotational partition function of a spherical rotor, noting that each energy level is ^2)
-fold degenerate, is given by:
^2e^{-\frac{hcBJ(J+1)}{kT}}\;\;\;\;\;\;\;\;276)
Similar to linear rotors, at low temperatures is calculated by substituting experimental values of into eq276. At higher temperatures, we apply the high-temperature approximation, with eq276 becoming:
^2e^{-\frac{\theta_RJ(J+1)}{T}}\;\;\;\;\;\;\;\;277)
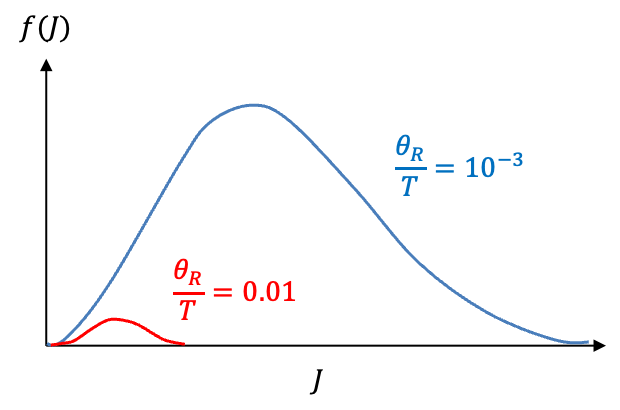
The factor in the function ^2e^{-\frac{\theta_RJ(J+1)}{T}})
increases quadratically with , while }{T}})
decreases exponentially. It follows that the graph of 
versus forms a skewed bell curve with a maximum. If , the contribution of to the integral is negligible at low and becomes significant only at higher (see diagram below). Therefore, eq277 can be simplified to:
Question
Prove that 
for 
.
Answer
We shall begin with the identity 
(see this article for derivation).
So,
Eq278 evaluates to:
Like linear rotors, the symmetry number is added to prevent overcounting of rotational states, giving:
^{\frac{3}{2}}\;\;\;\;\;\;\;\;280)
Symmetric rotors
The energy equation of a symmetric rotor depends on three quantum numbers: =hc\tilde BJ(J+1)+K^2hc(\tilde A-\tilde B))
. Therefore, the rotational partition function for symmetric rotors involves three summations:
}{kT}}\;\;\;\;\;\;\;\;281)
or equivalently,
e^{-\frac{\varepsilon(J,K,M_J)}{kT}}\;\;\;\;\;\;\;\;282)
The double summation in eq282 involves fixing a value of and summing the allowed values of 
. Since runs from 
to 
, with 
for each , the minimum allowed value of for a given is 
. This allows us to change the order of summation in eq282 by fixing a value for and then summing over the allowed values of :
e^{-\frac{\varepsilon(J,K,M_J)}{kT}}\;\;\;\;\;\;\;\;283)
Substituting into eq283 and using the high-temperature approximation gives:
K^2}{kT}}dK \int_{\vert K\vert}^{\infty}(2J+1)e^{-\frac{hc\tilde BJ(J+1)}{kT}}dJ\;\;\;\;\;\;\;\;284)
Using the earlier argument that if , the contribution of e^{-\frac{hc\tilde BJ(J+1)}{kT}})
to the 2nd integral in eq284 is negligible at low and becomes significant only at higher , we have
K^2}{kT}}dK \int_{\vert K\vert}^{\infty}2Je^{-\frac{hc\tilde BJ^2}{kT}}dJ\;\;\;\;\;\;\;\;285)
Since 
, the 2nd integral in eq285 evaluates to 
and eq285 becomes:
K^2}{kT}}e^{-\frac{hc\tilde BK^2}{kT}}dK=\frac{kT}{hc\tilde B}\int_{-\infty}^{\infty}e^{-\frac{hc\tilde AK^2}{kT}}dK)
Using the identity 
, we have
where we have added the symmetry number (e.g. 
for NH3), with 
and 
.
Question
What is the expression for the rotational partition function of an asymmetric rotor?
Answer
An asymmetric rotor has three distinct moments of inertia: 
. Its rotational energy is characterised by three rotational constants: 
, 
and 
. If two of the moments of inertia are equal, the molecule becomes a symmetric rotor. It follows that the rotational partition function of asymmetric rotor is:
which reduces to eq286 when 
.
